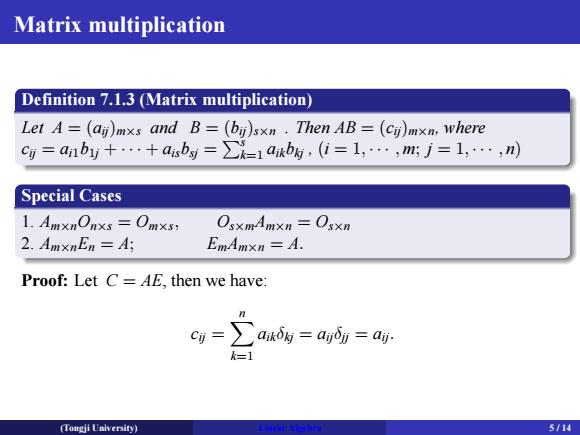
Matrix multiplication Definition 7.1.3(Matrix multiplication) Let A=(ay)mxs and B=(by)sxn.Then AB=(cy)mxn,where c可=a1b1y+…+asbg=k=1abg,(i=1,…,m;j=1,…,n)） Special Cases 1.AmxnOnxs =Omxs; OsxmAmxn=Osxn 2.AmxnEn =A; EmAmxn =A. Proof:Let C=AE,then we have: cg=∑aig=agdi=ag: k=1 (Tongji University) 5/14Matrix multiplication . Definition 7.1.3 (Matrix multiplication) . . Let A = (aij)m×s and B = (bij)s×n . Then AB = (cij)m×n, where cij = ai1b1j + · · · + aisbsj = ∑s k=1 aikbkj , (i = 1, · · · , m; j = 1, · · · , n) . Special Cases . . 1. Am×nOn×s = Om×s , Os×mAm×n = Os×n 2. Am×nEn = A; EmAm×n = A. Proof: Let C = AE, then we have: cij = ∑n k=1 aikδkj = aijδjj = aij. (Tongji University) Linear Algebra 5 / 14