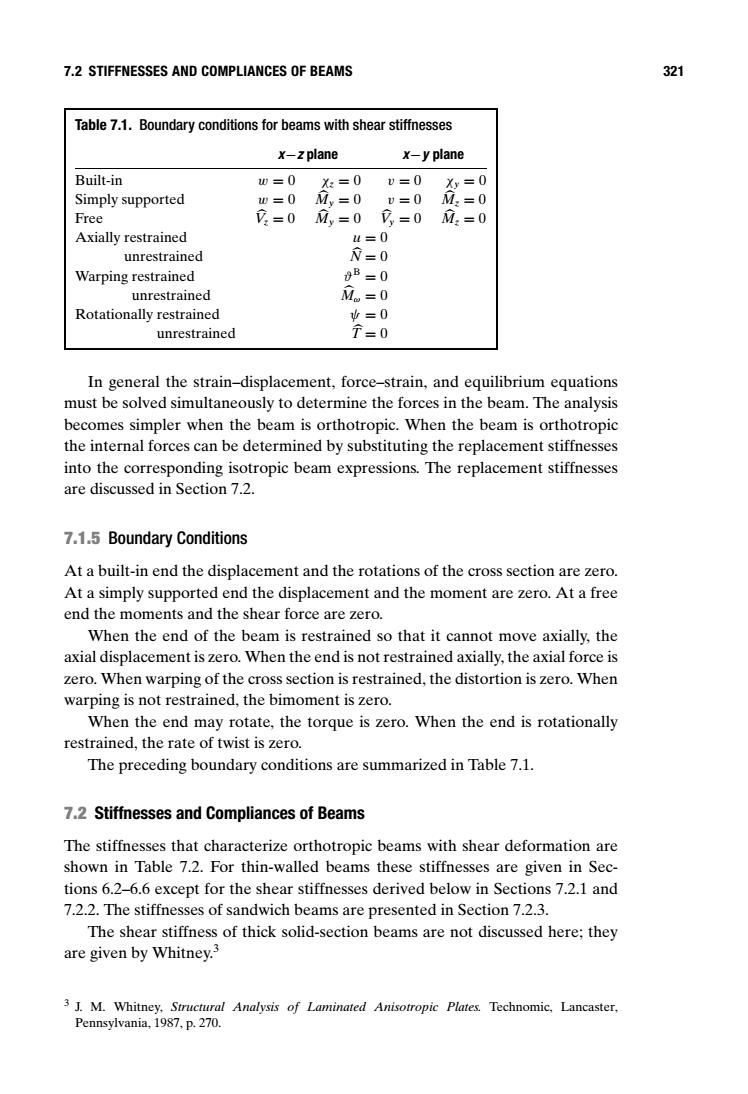
7.2 STIFFNESSES AND COMPLIANCES OF BEAMS 321 Table 7.1.Boundary conditions for beams with shear stiffnesses x-z plane x-y plane Built-in 0=0 X:=0 =0 Xy=0 Simply supported 0=0 应，=0 v=0 应=0 Free 立=0M,=0可=0应=0 Axially restrained 4=0 unrestrained N=0 Warping restrained 0B=0 unrestrained M.=0 Rotationally restrained 业=0 unrestrained f=0 In general the strain-displacement,force-strain,and equilibrium equations must be solved simultaneously to determine the forces in the beam.The analysis becomes simpler when the beam is orthotropic.When the beam is orthotropic the internal forces can be determined by substituting the replacement stiffnesses into the corresponding isotropic beam expressions.The replacement stiffnesses are discussed in Section 7.2. 7.1.5 Boundary Conditions At a built-in end the displacement and the rotations of the cross section are zero. At a simply supported end the displacement and the moment are zero.At a free end the moments and the shear force are zero. When the end of the beam is restrained so that it cannot move axially,the axial displacement is zero.When the end is not restrained axially,the axial force is zero.When warping of the cross section is restrained,the distortion is zero.When warping is not restrained,the bimoment is zero. When the end may rotate,the torque is zero.When the end is rotationally restrained,the rate of twist is zero. The preceding boundary conditions are summarized in Table 7.1. 7.2 Stiffnesses and Compliances of Beams The stiffnesses that characterize orthotropic beams with shear deformation are shown in Table 7.2.For thin-walled beams these stiffnesses are given in Sec- tions 6.2-6.6 except for the shear stiffnesses derived below in Sections 7.2.1 and 7.2.2.The stiffnesses of sandwich beams are presented in Section 7.2.3. The shear stiffness of thick solid-section beams are not discussed here;they are given by Whitney.3 3 J.M.Whitney,Structural Analysis of Laminated Anisotropic Plates.Technomic.Lancaster, Pennsylvania,1987,p.270.7.2 STIFFNESSES AND COMPLIANCES OF BEAMS 321 Table 7.1. Boundary conditions for beams with shear stiffnesses x−z plane x−y plane Built-in w = 0 χz = 0 v = 0 χy = 0 Simply supported w = 0 M
y = 0 v = 0 M
z = 0 Free V
z = 0 M
y = 0 V
y = 0 M
z = 0 Axially restrained u = 0 unrestrained N
= 0 Warping restrained ϑB = 0 unrestrained M
ω = 0 Rotationally restrained ψ = 0 unrestrained T
= 0 In general the strain–displacement, force–strain, and equilibrium equations must be solved simultaneously to determine the forces in the beam. The analysis becomes simpler when the beam is orthotropic. When the beam is orthotropic the internal forces can be determined by substituting the replacement stiffnesses into the corresponding isotropic beam expressions. The replacement stiffnesses are discussed in Section 7.2. 7.1.5 Boundary Conditions At a built-in end the displacement and the rotations of the cross section are zero. At a simply supported end the displacement and the moment are zero. At a free end the moments and the shear force are zero. When the end of the beam is restrained so that it cannot move axially, the axial displacement is zero. When the end is not restrained axially, the axial force is zero. When warping of the cross section is restrained, the distortion is zero. When warping is not restrained, the bimoment is zero. When the end may rotate, the torque is zero. When the end is rotationally restrained, the rate of twist is zero. The preceding boundary conditions are summarized in Table 7.1. 7.2 Stiffnesses and Compliances of Beams The stiffnesses that characterize orthotropic beams with shear deformation are shown in Table 7.2. For thin-walled beams these stiffnesses are given in Sec￾tions 6.2–6.6 except for the shear stiffnesses derived below in Sections 7.2.1 and 7.2.2. The stiffnesses of sandwich beams are presented in Section 7.2.3. The shear stiffness of thick solid-section beams are not discussed here; they are given by Whitney.3 3 J. M. Whitney, Structural Analysis of Laminated Anisotropic Plates. Technomic, Lancaster, Pennsylvania, 1987, p. 270