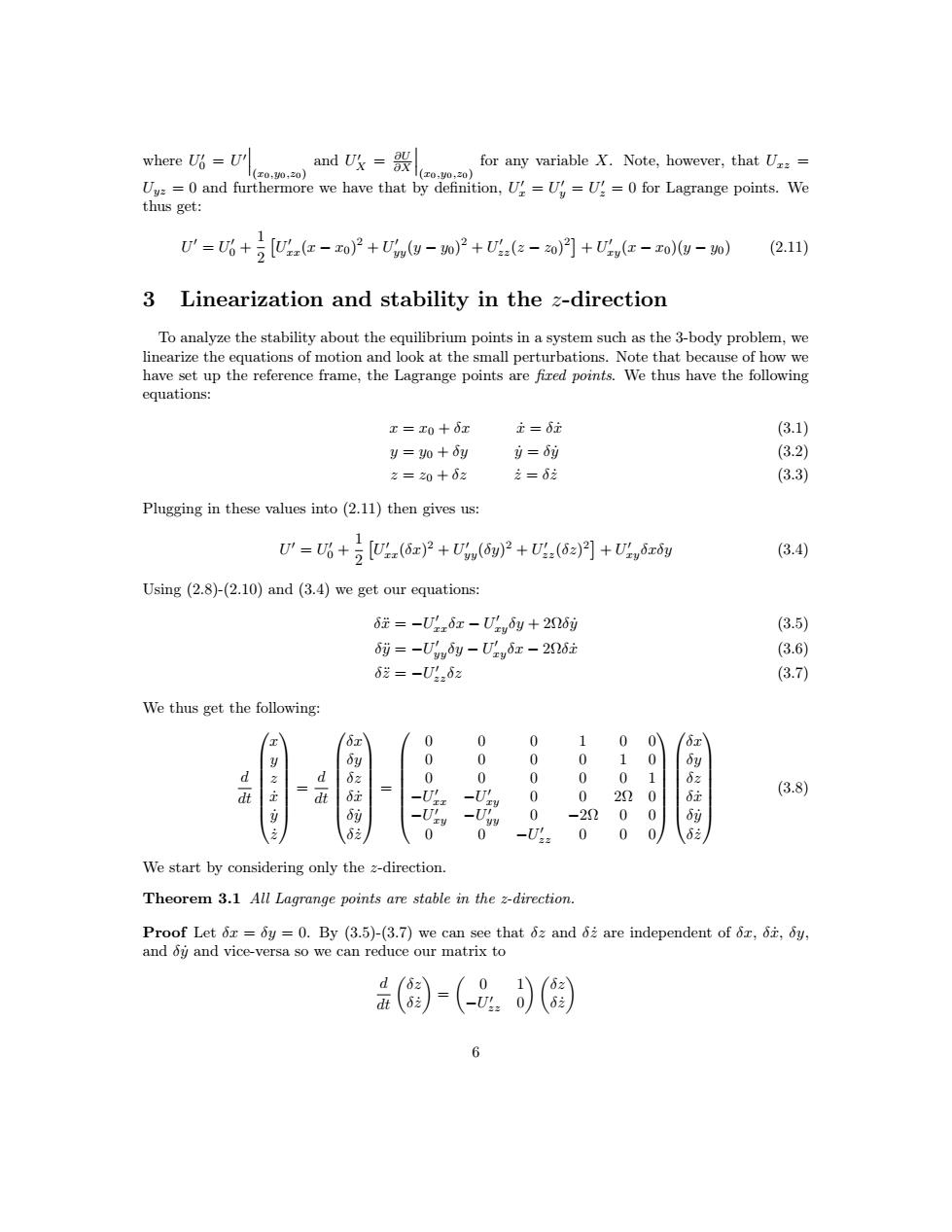
where U=U for any variable X.Note,however,that Urz= 1(0,0,20 andU收=毁on Uv:=0 and furthermore we have that by definition,U2=U=U:=0 for Lagrange points.We thus get: U'-U+U(-zo)+U(w-w)+U:(+U(-z0)(w-w) (2.11) 3 Linearization and stability in the z-direction To analyze the stability about the equilibrium points in a system such as the 3-body problem,we linearize the equations of motion and look at the small perturbations.Note that because of how we have set up the reference frame,the Lagrange points are fired points.We thus have the following equations: x=x0十6x 立=6 (3.1) y=0十dy 9=6y (3.2) 2=20+02 交=68 (3.3) Plugging in these values into(2.11)then gives us: U=6+号g(iajP+U6wP+U.(6a月+U%iai (3.4) Using(2.8)-(2.10)and(3.4)we get our equations: 6元=-UJz6x-Um6y+226i (3.5) =-Uwy-U2yi证-206i (3.6) 6龙=-U:26z (3.7) We thus get the following: 0 0 0 1 0 01 0 0 0 0 1 0 2 d 0 0 0 0 0 1 di dt -Uz 0 0 22 0 6元 (3.8) 9 60 -U' 0 -22 0 0 6问 6 0 0 -U:: 0 0 0 62 We start by considering only the z-direction. Theorem 3.1 All Lagrange points are stable in the z-direction. Proof Let 6r =y=0.By (3.5)-(3.7)we can see that 6z and 6i are independent of 6r,6i,6y, and y and vice-versa so we can reduce our matrix to 品()）=(8.)（周 6where U 0 0 = U 0    (x0,y0,z0) and U 0 X = ∂U ∂X    (x0,y0,z0) for any variable X. Note, however, that Uxz = Uyz = 0 and furthermore we have that by definition, U 0 x = U 0 y = U 0 z = 0 for Lagrange points. We thus get: U 0 = U 0 0 + 1 2 U 0 xx(x − x0) 2 + U 0 yy(y − y0) 2 + U 0 zz(z − z0) 2 + U 0 xy(x − x0)(y − y0) (2.11) 3 Linearization and stability in the z-direction To analyze the stability about the equilibrium points in a system such as the 3-body problem, we linearize the equations of motion and look at the small perturbations. Note that because of how we have set up the reference frame, the Lagrange points are fixed points. We thus have the following equations: x = x0 + δx x˙ = δx˙ (3.1) y = y0 + δy y˙ = δy˙ (3.2) z = z0 + δz z˙ = δz˙ (3.3) Plugging in these values into (2.11) then gives us: U 0 = U 0 0 + 1 2 U 0 xx(δx) 2 + U 0 yy(δy) 2 + U 0 zz(δz) 2 + U 0 xyδxδy (3.4) Using (2.8)-(2.10) and (3.4) we get our equations: δx¨ = −U 0 xxδx − U 0 xyδy + 2Ωδy˙ (3.5) δy¨ = −U 0 yyδy − U 0 xyδx − 2Ωδx˙ (3.6) δz¨ = −U 0 zzδz (3.7) We thus get the following: d dt   x y z x˙ y˙ z˙   = d dt   δx δy δz δx˙ δy˙ δz˙   =   0 0 0 1 0 0 0 0 0 0 1 0 0 0 0 0 0 1 −U 0 xx −U 0 xy 0 0 2Ω 0 −U 0 xy −U 0 yy 0 −2Ω 0 0 0 0 −U 0 zz 0 0 0     δx δy δz δx˙ δy˙ δz˙   (3.8) We start by considering only the z-direction. Theorem 3.1 All Lagrange points are stable in the z-direction. Proof Let δx = δy = 0. By (3.5)-(3.7) we can see that δz and δz˙ are independent of δx, δx˙, δy, and δy˙ and vice-versa so we can reduce our matrix to d dt  δz δz˙  =  0 1 −U 0 zz 0  δz δz˙  6