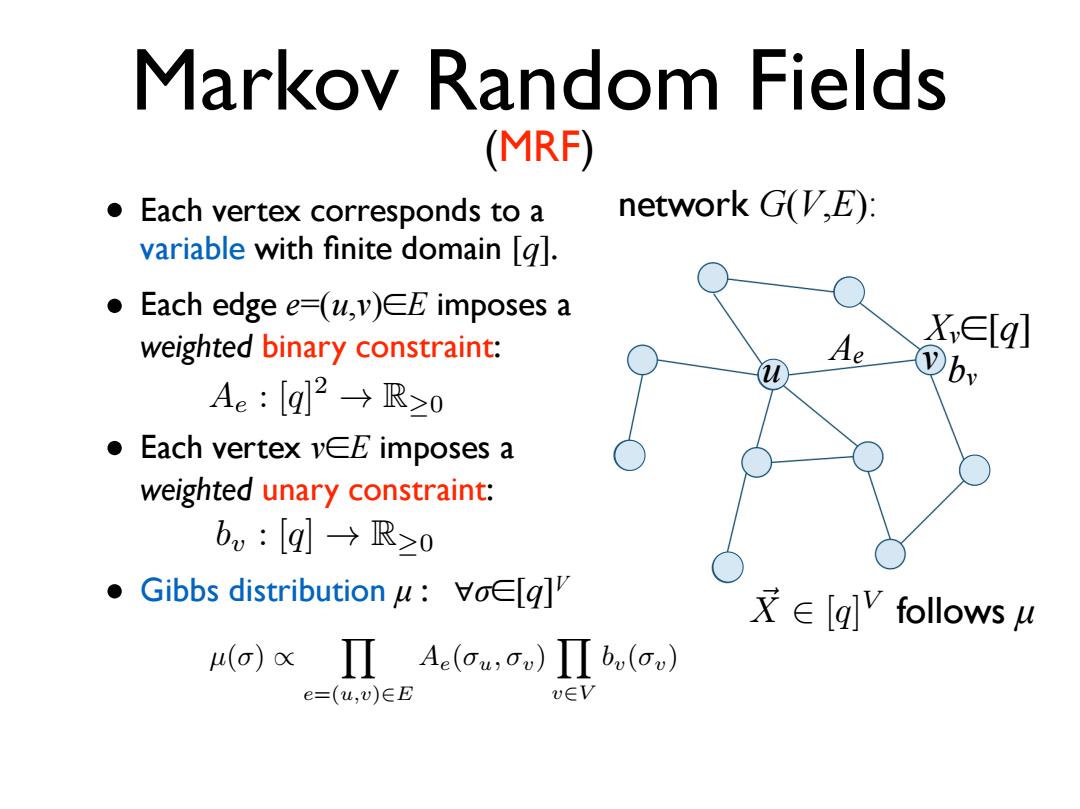
Markoy Random Fields (MRF) Each vertex corresponds to a network G(E): variable with finite domain [g]. ● Each edge e=(u,v)EE imposes a weighted binary constraint: X∈[q] ⑨bv Ae:[gl2→R≥0 。Each vertex ve∈imposes a weighted unary constraint: b:[gl→R≥o Gibbs distribution u:∀o∈[g]' X∈[a]V follows u L(o)Ae(ou,0)b() e=(u,v)∈E u∈VMarkov Random Fields network G(V,E): • Each vertex corresponds to a variable with finite domain [q]. • Each edge e=(u,v)∈E imposes a weighted binary constraint: • Each vertex v∈E imposes a weighted unary constraint: • Gibbs distribution µ : ∀σ∈[q]V Ae : [q] 2 ! R￾0 bv : [q] ! R￾0 µ(￾) / Y e=(u,v)2E Ae(￾u, ￾v) Y v2V bv(￾v) Ae bv Xv∈[q] u v (MRF) X ~ 2 [q] V follows µ