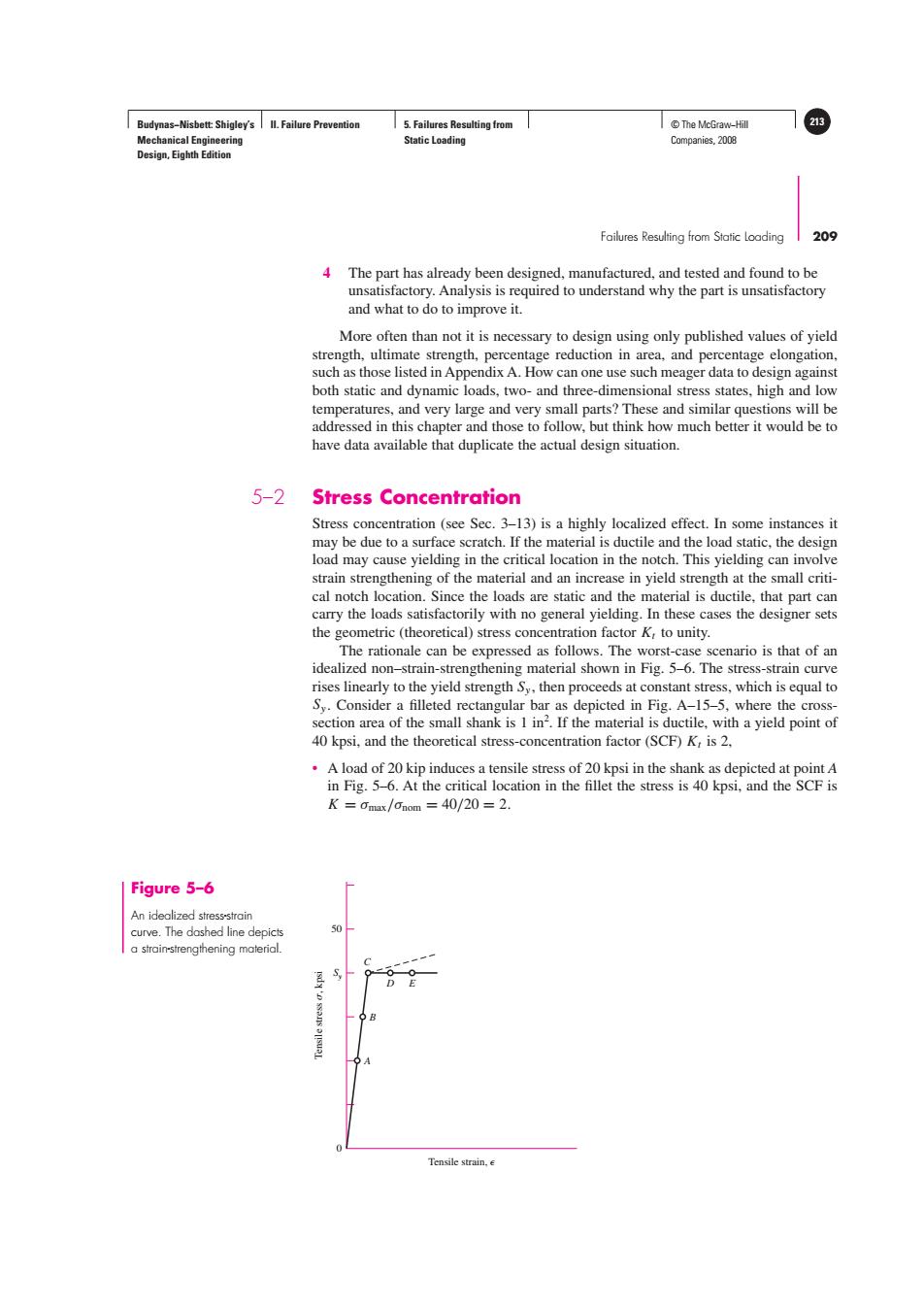
Budynas-Nisbett:Shigley's ll.Failure Prevention 5.Failures Resulting from T©The McGraw-Hill 213 Mechanical Engineering Static Loading Companies,2008 Design,Eighth Edition Failures Resulting from Static Loading 209 4 The part has already been designed,manufactured,and tested and found to be unsatisfactory.Analysis is required to understand why the part is unsatisfactory and what to do to improve it. More often than not it is necessary to design using only published values of yield strength,ultimate strength,percentage reduction in area,and percentage elongation, such as those listed in Appendix A.How can one use such meager data to design against both static and dynamic loads,two-and three-dimensional stress states,high and low temperatures,and very large and very small parts?These and similar questions will be addressed in this chapter and those to follow,but think how much better it would be to have data available that duplicate the actual design situation. 5-2 Stress Concentration Stress concentration (see Sec.3-13)is a highly localized effect.In some instances it may be due to a surface scratch.If the material is ductile and the load static,the design load may cause yielding in the critical location in the notch.This yielding can involve strain strengthening of the material and an increase in yield strength at the small criti- cal notch location.Since the loads are static and the material is ductile,that part can carry the loads satisfactorily with no general yielding.In these cases the designer sets the geometric (theoretical)stress concentration factor K,to unity. The rationale can be expressed as follows.The worst-case scenario is that of an idealized non-strain-strengthening material shown in Fig.5-6.The stress-strain curve rises linearly to the yield strength Sy,then proceeds at constant stress,which is equal to Sy.Consider a filleted rectangular bar as depicted in Fig.A-15-5,where the cross- section area of the small shank is 1 in2.If the material is ductile,with a yield point of 40 kpsi,and the theoretical stress-concentration factor(SCF)K,is 2, A load of 20 kip induces a tensile stress of 20 kpsi in the shank as depicted at point A in Fig.5-6.At the critical location in the fillet the stress is 40 kpsi,and the SCF is K=0max/onom=40/20=2. Figure 5-6 An idealized stress-strain curve.The dashed line depicts a strain-strengthening material. Tensile strain.eBudynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition II. Failure Prevention 5. Failures Resulting from Static Loading © The McGraw−Hill 213 Companies, 2008 Failures Resulting from Static Loading 209 4 The part has already been designed, manufactured, and tested and found to be unsatisfactory. Analysis is required to understand why the part is unsatisfactory and what to do to improve it. More often than not it is necessary to design using only published values of yield strength, ultimate strength, percentage reduction in area, and percentage elongation, such as those listed in Appendix A. How can one use such meager data to design against both static and dynamic loads, two- and three-dimensional stress states, high and low temperatures, and very large and very small parts? These and similar questions will be addressed in this chapter and those to follow, but think how much better it would be to have data available that duplicate the actual design situation. 5–2 Stress Concentration Stress concentration (see Sec. 3–13) is a highly localized effect. In some instances it may be due to a surface scratch. If the material is ductile and the load static, the design load may cause yielding in the critical location in the notch. This yielding can involve strain strengthening of the material and an increase in yield strength at the small criti￾cal notch location. Since the loads are static and the material is ductile, that part can carry the loads satisfactorily with no general yielding. In these cases the designer sets the geometric (theoretical) stress concentration factor Kt to unity. The rationale can be expressed as follows. The worst-case scenario is that of an idealized non–strain-strengthening material shown in Fig. 5–6. The stress-strain curve rises linearly to the yield strength Sy , then proceeds at constant stress, which is equal to Sy . Consider a filleted rectangular bar as depicted in Fig. A–15–5, where the cross￾section area of the small shank is 1 in2 . If the material is ductile, with a yield point of 40 kpsi, and the theoretical stress-concentration factor (SCF) Kt is 2, • A load of 20 kip induces a tensile stress of 20 kpsi in the shank as depicted at point A in Fig. 5–6. At the critical location in the fillet the stress is 40 kpsi, and the SCF is K = σmax/σnom = 40/20 = 2. 50 0 Sy Tensile strain, Tensile stress , kpsi A B D E C Figure 5–6 An idealized stress-strain curve. The dashed line depicts a strain-strengthening material.