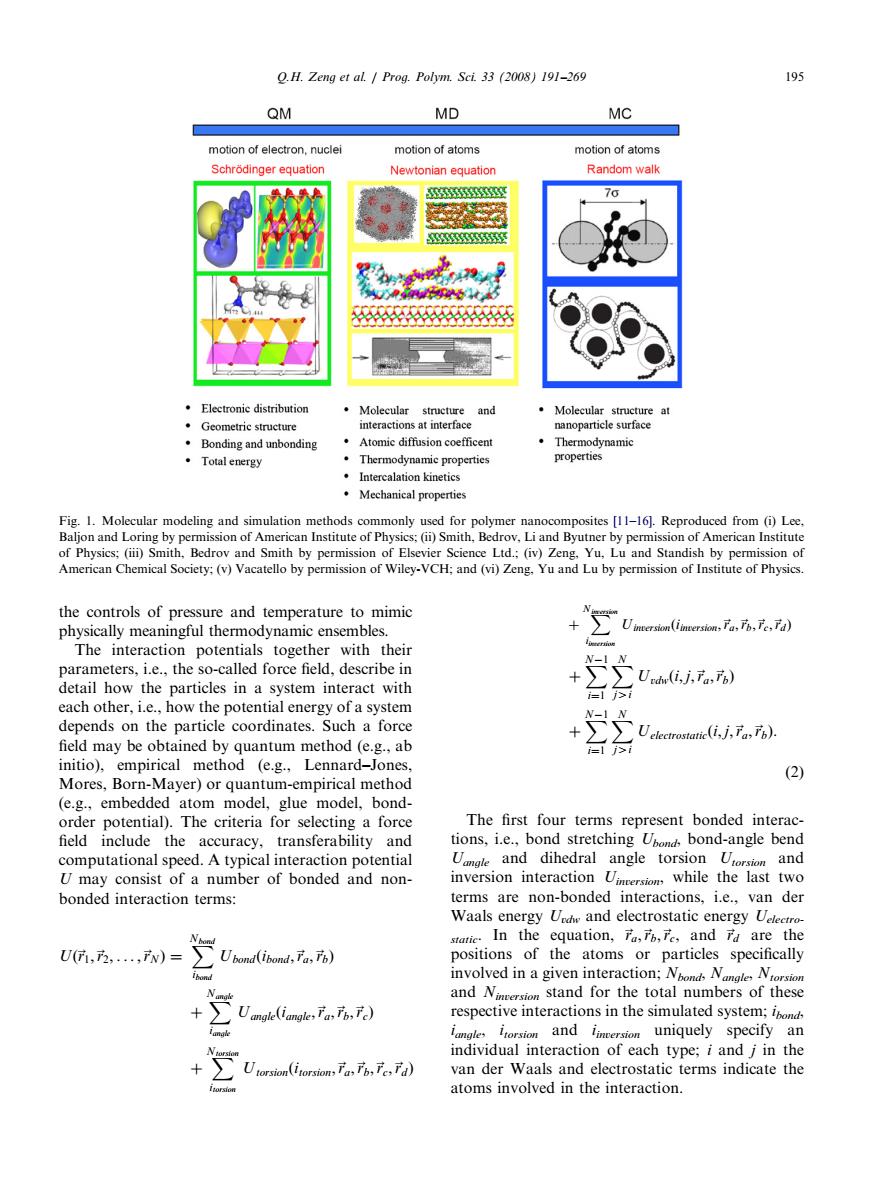
Q.H.Zeng et al Prog.Polym.SeL 33(2008)191-269 9 QM MD MC otion of electron,nuclei motion of atoms motion of atom chroainger equat 70 ●3 ctronis distribution anoparticle surface Bonding and unbonding micdifasioncocfec hterealaioakineties Mechanical properties ethods and lo n of a We-VCYn The interaction potentials together with their parameters,i.e.,the so-called force field,describe in detail how the particles in a system interact with each other,i.e.,how the potential energy of a system depends on the particle coordinates.Such a force may be obt me (e.g., (2) me0ho order potential).The criteria for selec The first four terms represent bonded interac- field include the accuracy.transferability and tions,i.e.,bond stretching ond,bond-angle bend computational speed.A typical interaction potential fhreioanierqio and dihedral angle torsion and U may consist of a number of bonded and non- whil the last two bonded interaction terms interactions 1.e.,van de energy and el energy a U,2,,fN)= involved in a given interaction:NN and N. stand for the total numbers of these respective interactions in the simulated system; and linrersion uniquely specily an indi idual interaction of each type;i and j in the the controls of pressure and temperature to mimic physically meaningful thermodynamic ensembles. The interaction potentials together with their parameters, i.e., the so-called force field, describe in detail how the particles in a system interact with each other, i.e., how the potential energy of a system depends on the particle coordinates. Such a force field may be obtained by quantum method (e.g., ab initio), empirical method (e.g., Lennard–Jones, Mores, Born-Mayer) or quantum-empirical method (e.g., embedded atom model, glue model, bond￾order potential). The criteria for selecting a force field include the accuracy, transferability and computational speed. A typical interaction potential U may consist of a number of bonded and non￾bonded interaction terms: Uð~r1;~r2; ... ;~rNÞ ¼ N Xbond ibond Ubond ðibond ;~ra;~rbÞ þ N X angle iangle Uangleðiangle;~ra;~rb;~rcÞ þ N Xtorsion itorsion Utorsionðitorsion;~ra;~rb;~rc;~rd Þ þ NXinversion iinversion Uinversionðiinversion;~ra;~rb;~rc;~rd Þ þ N X1 i¼1 X N j4i Uvdwði; j;~ra;~rbÞ þ N X1 i¼1 X N j4i Uelectrostaticði; j;~ra;~rbÞ. ð2Þ The first four terms represent bonded interac￾tions, i.e., bond stretching Ubond, bond-angle bend Uangle and dihedral angle torsion Utorsion and inversion interaction Uinversion, while the last two terms are non-bonded interactions, i.e., van der Waals energy Uvdw and electrostatic energy Uelectro￾static. In the equation, ~ra;~rb;~rc, and ~rd are the positions of the atoms or particles specifically involved in a given interaction; Nbond, Nangle, Ntorsion and Ninversion stand for the total numbers of these respective interactions in the simulated system; ibond, iangle, itorsion and iinversion uniquely specify an individual interaction of each type; i and j in the van der Waals and electrostatic terms indicate the atoms involved in the interaction. ARTICLE IN PRESS Fig. 1. Molecular modeling and simulation methods commonly used for polymer nanocomposites [11–16]. Reproduced from (i) Lee, Baljon and Loring by permission of American Institute of Physics; (ii) Smith, Bedrov, Li and Byutner by permission of American Institute of Physics; (iii) Smith, Bedrov and Smith by permission of Elsevier Science Ltd.; (iv) Zeng, Yu, Lu and Standish by permission of American Chemical Society; (v) Vacatello by permission of Wiley-VCH; and (vi) Zeng, Yu and Lu by permission of Institute of Physics. Q.H. Zeng et al. / Prog. Polym. Sci. 33 (2008) 191–269 195