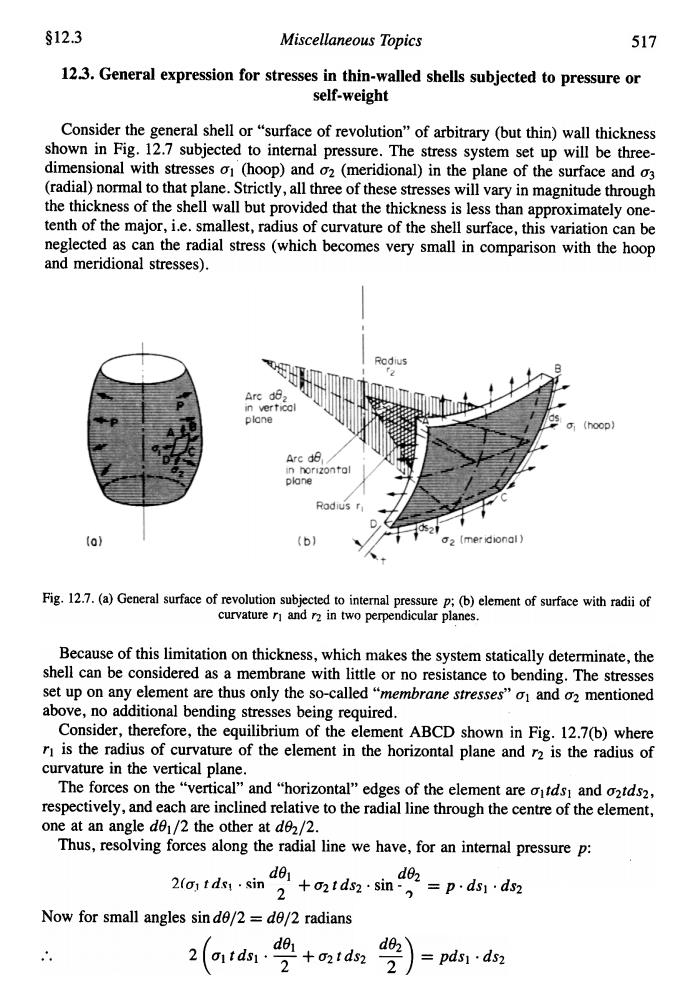
§12.3 Miscellaneous Topics 517 12.3.General expression for stresses in thin-walled shells subjected to pressure or self-weight Consider the general shell or "surface of revolution"of arbitrary (but thin)wall thickness shown in Fig.12.7 subjected to internal pressure.The stress system set up will be three- dimensional with stresses o1(hoop)and o2(meridional)in the plane of the surface and o3 (radial)normal to that plane.Strictly,all three of these stresses will vary in magnitude through the thickness of the shell wall but provided that the thickness is less than approximately one- tenth of the major,i.e.smallest,radius of curvature of the shell surface,this variation can be neglected as can the radial stress (which becomes very small in comparison with the hoop and meridional stresses). Rodius Arc d82 in vertical plane g1(hoop】 Arc de in horizontol plane Rodius r (b) 2 (mer idional Fig.12.7.(a)General surface of revolution subjected to internal pressure p:(b)element of surface with radii of curvature ri and r2 in two perpendicular planes. Because of this limitation on thickness,which makes the system statically determinate,the shell can be considered as a membrane with little or no resistance to bending.The stresses set up on any element are thus only the so-called"membrane stresses"and o2 mentioned above,no additional bending stresses being required. Consider,therefore,the equilibrium of the element ABCD shown in Fig.12.7(b)where rI is the radius of curvature of the element in the horizontal plane and r2 is the radius of curvature in the vertical plane. The forces on the "vertical"and "horizontal"edges of the element are oitds and oztds2, respectively,and each are inclined relative to the radial line through the centre of the element, one at an angle de1/2 the other at de2/2. Thus,resolving forces along the radial line we have,for an internal pressure p: 2(din d d02 Now for small angles sin de/2=de/2 radians =pds1·ds2§12.3 Miscellaneous Topics 517 12.3. General expression for stresses in thin-walled shells subjected to pressure or self-weight Consider the general shell or "surface of revolution" of arbitrary (but thin) wall thickness shown in Fig. 12.7 subjected to internal pressure. The stress system set up will be three￾dimensional with stresses a) (hoop) and a2 (meridional) in the plane of the surface and a3 (radial) normal to that plane. Strictly, all three of these stresses will vary in magnitude through the thickness of the shell wall but provided that the thickness is less than approximately one￾tenth of the major, i.e. smallest, radius of curvature of the shell surface, this variation can be neglected as can the radial stress (which becomes very small in comparison with the hoop and meridional stresses). Fig. 12.7. (a) General surface of revolution subjected to internal pressure p; (b) element of surface with radii of curvature rl and r2 in two perpendicular planes. Because of this limitation on thickness, which makes the system statically determinate, the shell can be considered as a membrane with little or no resistance to bending. The stresses set up on any element are thus only the so-called "membrane stresses" al and a2 mentioned above, no additional bending stresses being required. Consider, therefore, the equilibrium of the element ABCD shown in Fig. 12.7(b) where rl is the radius of curvature of the element in the horizontal plane and r2 is the radius of curvature in the vertical plane. The forces on the "vertical" and "horizontal" edges of the element are altdsl and a2tds2, respectively, and each are inclined relative to the radial line through the centre of the element, one at an angle d(}l/2 the other at d(}2/2. Thus, resolving forces along the radial line we have, for an internal pressure p: .d(}l .d(}2 2(al t dsJ .SIn ~ + a2 t ds2 .SIn -= p .dsl .dS2 2 " Now for small angles sinde/2 = de/2 radians ( del 2 (11 t dsl .-+ (12 t dS2 ~ ) = pdsl .dS2 2 2