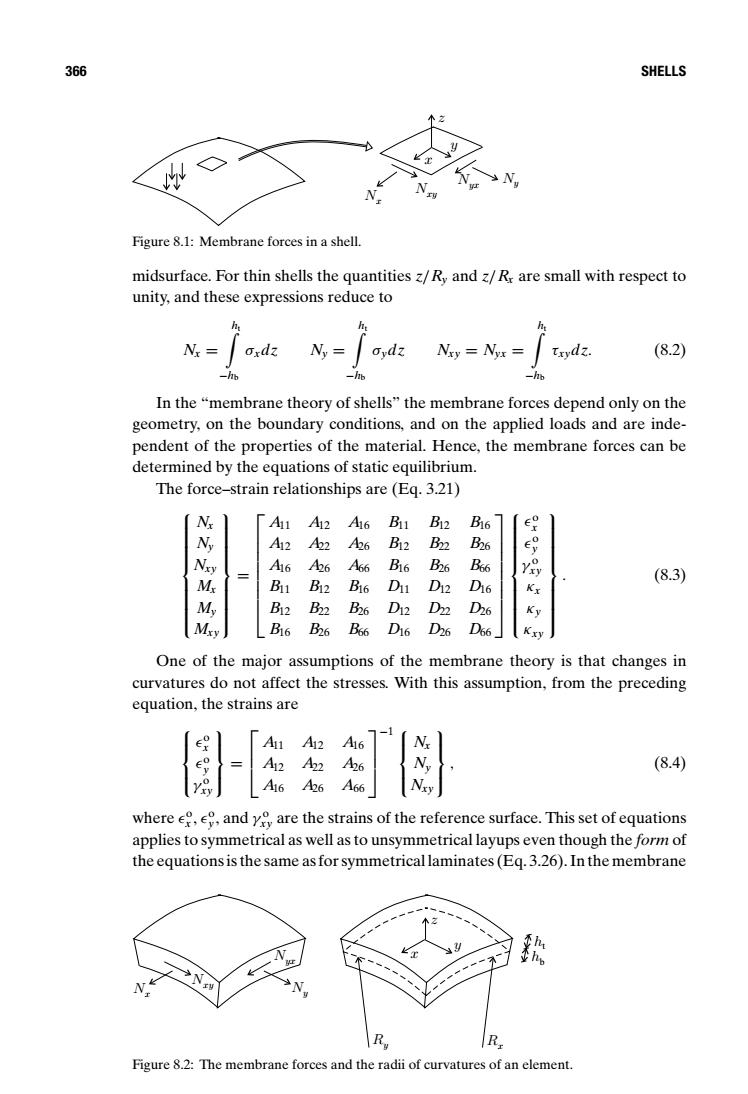
366 SHELLS Figure 8.1:Membrane forces in a shell. midsurface.For thin shells the quantities z/Ry and z/Rr are small with respect to unity,and these expressions reduce to N= (8.2) h In the "membrane theory of shells"the membrane forces depend only on the geometry,on the boundary conditions,and on the applied loads and are inde- pendent of the properties of the material.Hence,the membrane forces can be determined by the equations of static equilibrium. The force-strain relationships are(Eq.3.21) N A A2 A16 B11 B12 B16 ∈ N A2 A2 A6 B12 Bn B26 A16 A66 B16 B26 B66 Y M Bi1 D16 (8.3) B12 B16 Di1 D12 B12 B22 B26 D12 D22 D26 Mey B16 B26 B66 D16 D26 D66 Kxy One of the major assumptions of the membrane theory is that changes in curvatures do not affect the stresses.With this assumption,from the preceding equation,the strains are (8.4) A6 A26 A66 where e,andy are the strains of the reference surface.This set of equations applies to symmetrical as well as to unsymmetrical layups even though the form of the equations is the same as for symmetrical laminates(Eq.3.26).In the membrane Figure 8.2:The membrane forces and the radii of curvatures of an element.366 SHELLS y z x Nx Ny Nxy Nyx Figure 8.1: Membrane forces in a shell. midsurface. For thin shells the quantities z/Ry and z/Rx are small with respect to unity, and these expressions reduce to Nx = ) ht −hb σxdz Ny = ) ht −hb σydz Nxy = Nyx = ) ht −hb τxydz. (8.2) In the “membrane theory of shells” the membrane forces depend only on the geometry, on the boundary conditions, and on the applied loads and are inde￾pendent of the properties of the material. Hence, the membrane forces can be determined by the equations of static equilibrium. The force–strain relationships are (Eq. 3.21)    Nx Ny Nxy Mx My Mxy    =          A11 A12 A16 B11 B12 B16 A12 A22 A26 B12 B22 B26 A16 A26 A66 B16 B26 B66 B11 B12 B16 D11 D12 D16 B12 B22 B26 D12 D22 D26 B16 B26 B66 D16 D26 D66             o x o y γ o xy κx κy κxy    . (8.3) One of the major assumptions of the membrane theory is that changes in curvatures do not affect the stresses. With this assumption, from the preceding equation, the strains are    o x o y γ o xy    =    A11 A12 A16 A12 A22 A26 A16 A26 A66    −1    Nx Ny Nxy    , (8.4) where o x , o y , and γ o xy are the strains of the reference surface. This set of equations applies to symmetrical as well as to unsymmetrical layups even though the form of the equations is the same as for symmetrical laminates (Eq. 3.26). In the membrane y z x Nx Ny Nxy Nyx Ry Rx ht hb Figure 8.2: The membrane forces and the radii of curvatures of an element