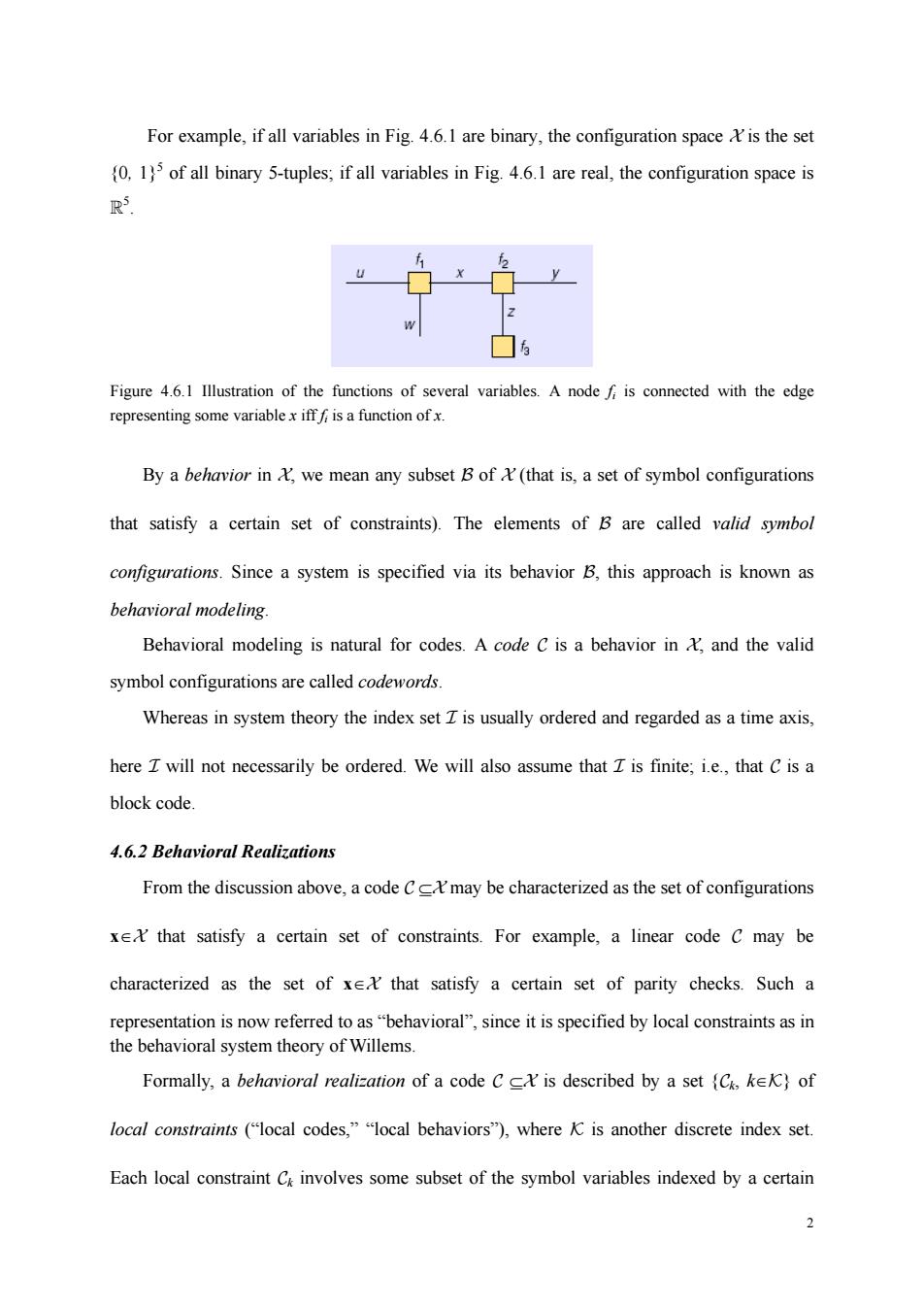
For example,if all variables in Fig.4.6.1 are binary,the configuration spaceis the set (0.1)of all binary 5-tuples;if all variables in Fig.4.6.1 are real,the configuration space is R Figure 4.6.1 Illustration of the functions of several variables.A nodeis connected with the edge representing some variableis a functionof By a behavior in &we mean any subset B of(that is,a set of symbol configurations that satisfy a certain set of constraints).The elements of B are called valid symbol configurations.Since a system is specified via its behavior B,this approach is known as behavioral modeling. Behavioral modeling is natural for codes.A code C is a behavior in and the valid symbol configurations are called Whereas in system theory the index set is usually ordered and regarded as a time axis, here Z will not necessarily be ordered.We will also assume that is finite;i.e.,that C is a block code. 4.6.2 Behavioral Realizations From the discussion above,a code Cmay be characterized as the set of configurations xthat satisfy a certain set of constraints.For example,a linear code c may be characterized as the set of xe that satisfy a certain set of parity checks.Such a representation is now referred to as"behavioral",since it is specified by local constraints as in the behavioral system theory of Willems. Formally,a behavioral realization of a code Cis described by a local conrains("lcal codes,""cal behaviors"),whereKis another discrete index set. Each local constraint C involves some subset of the symbol variables indexed by a certain2 For example, if all variables in Fig. 4.6.1 are binary, the configuration space X is the set {0, 1}5 of all binary 5-tuples; if all variables in Fig. 4.6.1 are real, the configuration space is R5 . Figure 4.6.1 Illustration of the functions of several variables. A node fi is connected with the edge representing some variable x iff fi is a function of x. By a behavior in X, we mean any subset B of X (that is, a set of symbol configurations that satisfy a certain set of constraints). The elements of B are called valid symbol configurations. Since a system is specified via its behavior B, this approach is known as behavioral modeling. Behavioral modeling is natural for codes. A code C is a behavior in X, and the valid symbol configurations are called codewords. Whereas in system theory the index set I is usually ordered and regarded as a time axis, here I will not necessarily be ordered. We will also assume that I is finite; i.e., that C is a block code. 4.6.2 Behavioral Realizations From the discussion above, a code C ⊆X may be characterized as the set of configurations x∈X that satisfy a certain set of constraints. For example, a linear code C may be characterized as the set of x∈X that satisfy a certain set of parity checks. Such a representation is now referred to as “behavioral”, since it is specified by local constraints as in the behavioral system theory of Willems. Formally, a behavioral realization of a code C ⊆X is described by a set {Ck, k∈K} of local constraints (“local codes,” “local behaviors”), where K is another discrete index set. Each local constraint Ck involves some subset of the symbol variables indexed by a certain