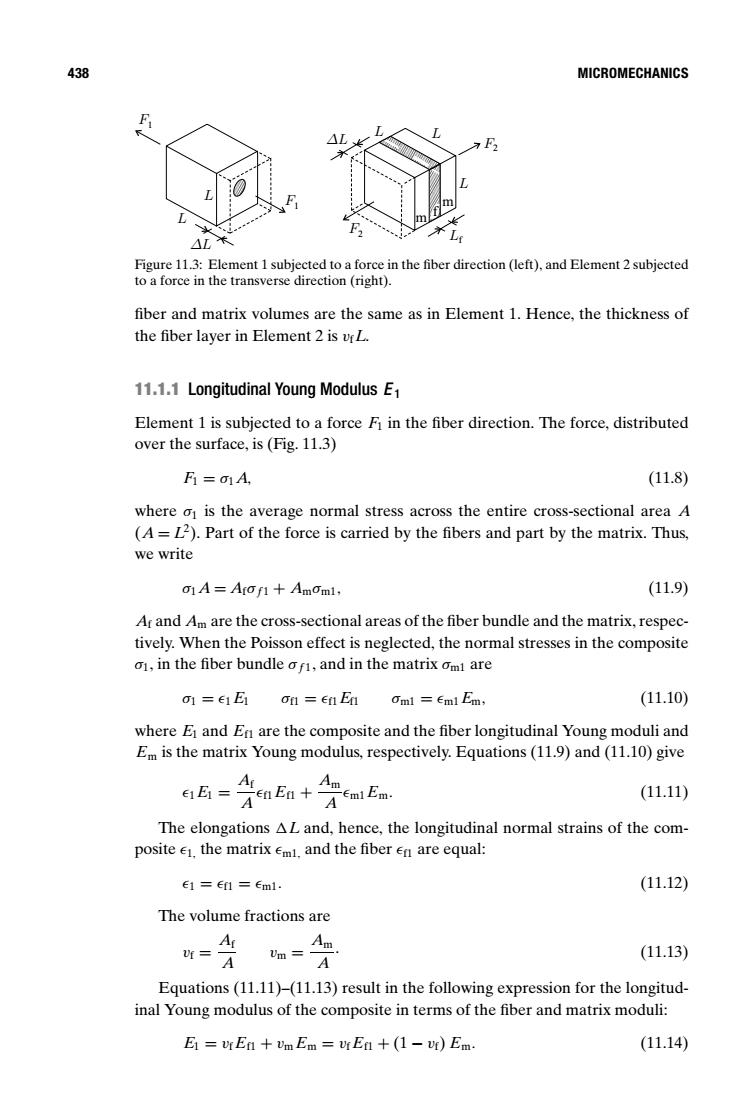
438 MICROMECHANICS F 4L米 Figure 11.3:Element 1 subjected to a force in the fiber direction(left),and Element 2 subjected to a force in the transverse direction (right). fiber and matrix volumes are the same as in Element 1.Hence,the thickness of the fiber layer in Element 2 is vrL. 11.1.1 Longitudinal Young Modulus E1 Element 1 is subjected to a force F in the fiber direction.The force,distributed over the surface,is(Fig.11.3) F1=01A, (11.8) where o1 is the average normal stress across the entire cross-sectional area A (A=L2).Part of the force is carried by the fibers and part by the matrix.Thus, we write 01A=Arof1+Amom1, (11.9) Ar and Am are the cross-sectional areas of the fiber bundle and the matrix,respec- tively.When the Poisson effect is neglected,the normal stresses in the composite o1,in the fiber bundle ofi,and in the matrix omi are 01 =E1E1 of ef En Oml =Eml Em, (11.10) where E and En are the composite and the fiber longitudinal Young moduli and Em is the matrix Young modulus,respectively.Equations(11.9)and (11.10)give a点=头aa+六mEe (11.11) The elongations AL and,hence,the longitudinal normal strains of the com- posite e1.the matrix em1.and the fiber en are equal: E1 Ef1=Eml. (11.12) The volume fractions are = m mA (11.13) Equations(11.11)-(11.13)result in the following expression for the longitud- inal Young modulus of the composite in terms of the fiber and matrix moduli: E1=vrEn vm Em=vrEa +(1-vr)Em. (11.14)438 MICROMECHANICS F1 F1 L ∆L L F2 F2 m m f ∆L L L L Lf Figure 11.3: Element 1 subjected to a force in the fiber direction (left), and Element 2 subjected to a force in the transverse direction (right). fiber and matrix volumes are the same as in Element 1. Hence, the thickness of the fiber layer in Element 2 is vfL. 11.1.1 Longitudinal Young Modulus E 1 Element 1 is subjected to a force F1 in the fiber direction. The force, distributed over the surface, is (Fig. 11.3) F1 = σ1A, (11.8) where σ1 is the average normal stress across the entire cross-sectional area A (A = L2). Part of the force is carried by the fibers and part by the matrix. Thus, we write σ1A = Afσ f 1 + Amσm1, (11.9) Af and Am are the cross-sectional areas of the fiber bundle and the matrix, respec￾tively. When the Poisson effect is neglected, the normal stresses in the composite σ1, in the fiber bundle σ f 1, and in the matrix σm1 are σ1 = 1E1 σf1 = f1Ef1 σm1 = m1Em, (11.10) where E1 and Ef1 are the composite and the fiber longitudinal Young moduli and Em is the matrix Young modulus, respectively. Equations (11.9) and (11.10) give 1E1 = Af A f1Ef1 + Am A m1Em. (11.11) The elongations L and, hence, the longitudinal normal strains of the com￾posite 1, the matrix m1, and the fiber f1 are equal: 1 = f1 = m1. (11.12) The volume fractions are vf = Af A vm = Am A . (11.13) Equations (11.11)–(11.13) result in the following expression for the longitud￾inal Young modulus of the composite in terms of the fiber and matrix moduli: E1 = vfEf1 + vm Em = vfEf1 + (1 − vf) Em. (11.14)