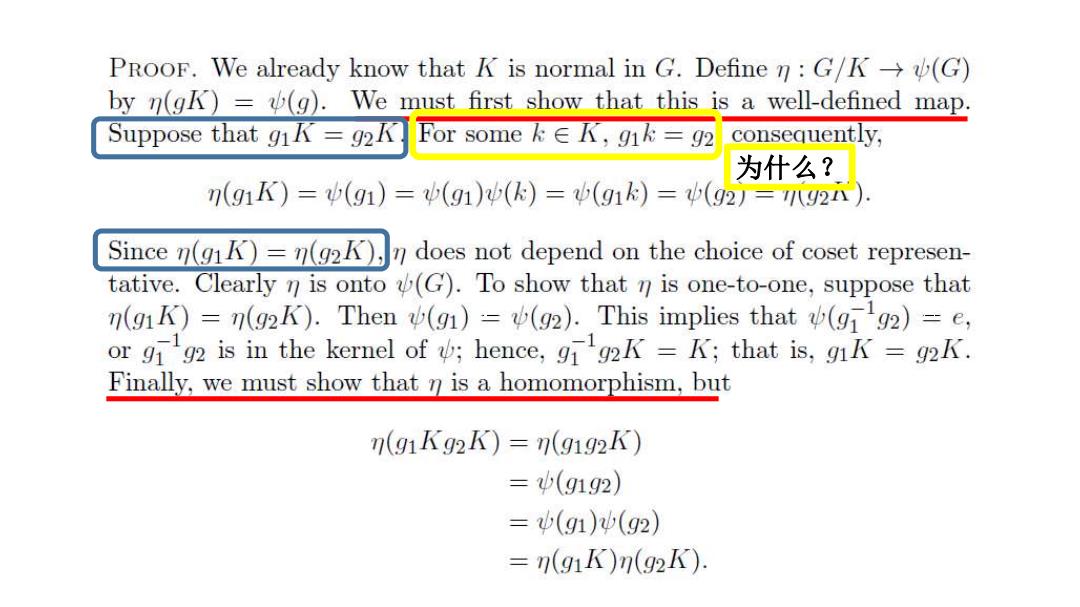
PROOF.We already know that K is normal in G.Define n:G/K(G) by n(gk)(g).We must first show that this is a well-defined map Suppose that gk=g2K For some kE K,91k =92 consequently, 为什么？ 7(g1K)=(g1)=(g1)(s)=(g1k)=(92)=92n) Since n(gk)=n(g2K),n does not depend on the choice of coset represen- tative.Clearly n is onto (G).To show that n is one-to-one,suppose that n(g1k)=n(g2K).Then (g1)=(g2).This implies that (g1g2)=e, or 91 g2 is in the kernel of v;hence,91g2k K;that is,gK =92K. Finally,we must show that n is a homomorphism,but 7(g1Kg2K)=7(g192K) =(g192) =(g1)(g2) =n(gk)n(g2K)为什么？