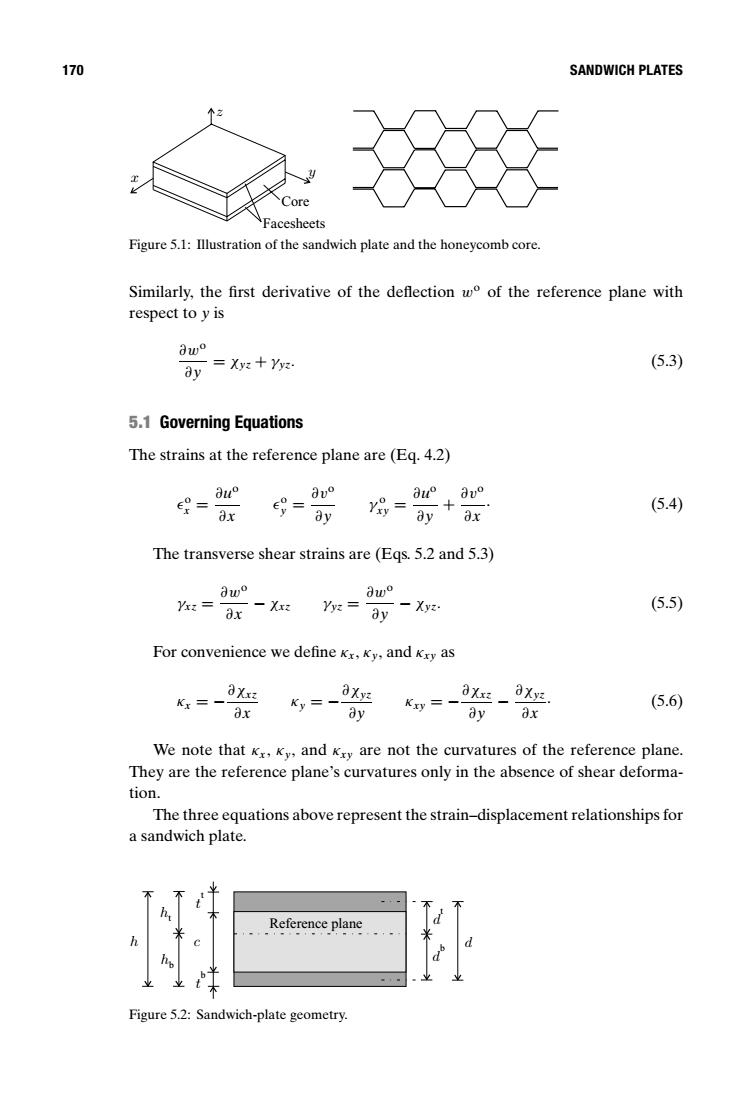
170 SANDWICH PLATES Core Facesheets Figure 5.1:Illustration of the sandwich plate and the honeycomb core. Similarly,the first derivative of the deflection wo of the reference plane with respect to y is dwo ay Xyz+Yyz. (5.3) 5.1 Governing Equations The strains at the reference plane are (Eq.4.2) au° 8v° 0°.auo e= ax ay y8= av ax (5.4) The transverse shear strains are(Egs.5.2 and 5.3) aw° 8w0 Yxz= ax -Xxz Yy:=ay -Xyz. (5.5) For convenience we define Kx,Ky,and Kxy as Kx=一 Xxz Ky=- aXyz Koy =-dxe aXy. (5.6) ax ay ay ax We note that K,K,and Ky are not the curvatures of the reference plane. They are the reference plane's curvatures only in the absence of shear deforma- tion. The three equations above represent the strain-displacement relationships for a sandwich plate. h, Reference plane Figure 5.2:Sandwich-plate geometry.170 SANDWICH PLATES x z y Core Facesheets Figure 5.1: Illustration of the sandwich plate and the honeycomb core. Similarly, the first derivative of the deflection wo of the reference plane with respect to y is ∂wo ∂y = χyz + γyz. (5.3) 5.1 Governing Equations The strains at the reference plane are (Eq. 4.2) o x = ∂uo ∂x o y = ∂vo ∂y γ o xy = ∂uo ∂y + ∂vo ∂x . (5.4) The transverse shear strains are (Eqs. 5.2 and 5.3) γxz = ∂wo ∂x − χxz γyz = ∂wo ∂y − χyz. (5.5) For convenience we define κx, κy, and κxy as κx = −∂χxz ∂x κy = −∂χyz ∂y κxy = −∂χxz ∂y − ∂χyz ∂x . (5.6) We note that κx, κy, and κxy are not the curvatures of the reference plane. They are the reference plane’s curvatures only in the absence of shear deforma￾tion. The three equations above represent the strain–displacement relationships for a sandwich plate. t b t t c hb ht d b d t h d Reference plane Figure 5.2: Sandwich-plate geometry