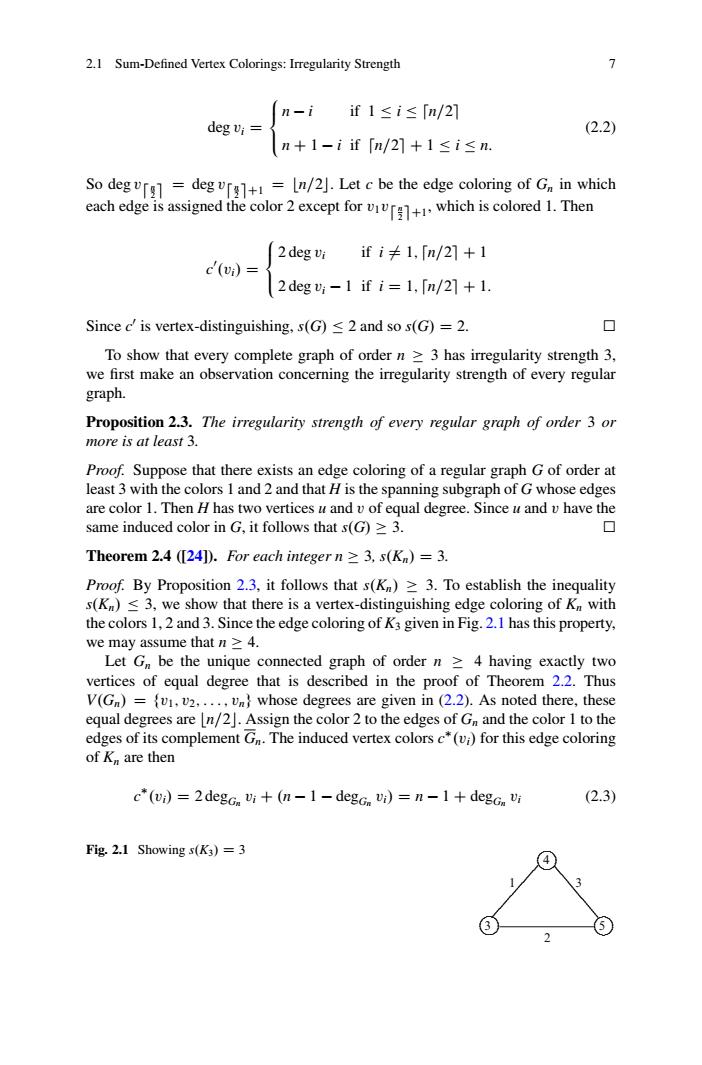
2.1 Sum-Defined Vertex Colorings:Irregularity Strength 7 n-iif1≤i≤[n/21 deg v; (2.2 n+1-iif[m/21+1≤i≤n. a盟 each edge ifi≠1，「n/21+1 c(u）= 2degy-1ifi=1,[n/21+1. Since c'is vertex-distinguishing.s(G)2 and so s(G)=2. To show that every complete graph of order n3 has irregularity strength 3 we first make an observation concerning the irregularity strength of every regular graph. Proposition 2.3.The irregularity strength of every regular graph of order 3 or more is at least 3. least 3 wi e color the span ng subgraph of C are color 1.Then H has two ertices u and of equal degree.Since uand v have th same induced color in G.it follows that s(G)>3 Theorem2.424.For each integern≥3，s(Ka）=3 Proof.By Proposition 2.3,it follows that s(K)>3.To establish the inequality s(K)<3,we show that there is a vertex-distinguishing edge coloring of Ka with the colors 1,2 and3.Since the edge coloring of K3 given in Fig.2.1 has this property connected graph of order n4 having ex vertices of equal degree that is described in the proof of Theorem 22.Thus V(Gn)=v1.v2.....v whose degrees are given in (2.2).As noted there,these equal degrees are [n/2].Assign the color 2 to the edges of Gn and the color 1 to the edges of its complement Gn.The induced vertex colors c*(v)for this edge coloring of Kn are then c*(vi)=2degG vi+(n-1-degG vi)=n-1 degg vi (2.3) Fig.2.1 Showing s(K3)=3 4 22.1 Sum-Defined Vertex Colorings: Irregularity Strength 7 deg vi D 8 < : n  i if 1 i dn=2e n C 1  i if dn=2e C 1 i n. (2.2) So deg vd n 2 e D deg vd n 2 eC1 D bn=2c. Let c be the edge coloring of Gn in which each edge is assigned the color 2 except for v1vd n 2 eC1, which is colored 1. Then c0 .vi/ D 8 < : 2 deg vi if i ¤ 1; dn=2e C 1 2 deg vi  1 if i D 1; dn=2e C 1. Since c0 is vertex-distinguishing, s.G/ 2 and so s.G/ D 2. To show that every complete graph of order n 3 has irregularity strength 3, we first make an observation concerning the irregularity strength of every regular graph. Proposition 2.3. The irregularity strength of every regular graph of order 3 or more is at least 3. Proof. Suppose that there exists an edge coloring of a regular graph G of order at least 3 with the colors 1 and 2 and that H is the spanning subgraph of G whose edges are color 1. Then H has two vertices u and v of equal degree. Since u and v have the same induced color in G, it follows that s.G/ 3. Theorem 2.4 ([24]). For each integer n 3, s.Kn/ D 3. Proof. By Proposition 2.3, it follows that s.Kn/ 3. To establish the inequality s.Kn/ 3, we show that there is a vertex-distinguishing edge coloring of Kn with the colors 1, 2 and 3. Since the edge coloring of K3 given in Fig. 2.1 has this property, we may assume that n 4. Let Gn be the unique connected graph of order n 4 having exactly two vertices of equal degree that is described in the proof of Theorem 2.2. Thus V.Gn/ D fv1; v2;:::;vng whose degrees are given in (2.2). As noted there, these equal degrees are bn=2c. Assign the color 2 to the edges of Gn and the color 1 to the edges of its complement Gn. The induced vertex colors c.vi/ for this edge coloring of Kn are then c.vi/ D 2 degGn vi C .n  1  degGn vi/ D n  1 C degGn vi (2.3) Fig. 2.1 Showing s.K3/ D 3 ... ... .. .. .... ............. .. ..... ........................................ ... ... .. .. .... ............. .. ..... ........................................ ... ... .. .. .... ........... .. .. ... ........................................ ...................................................... ...................................................... 4 3 5 2 1 3