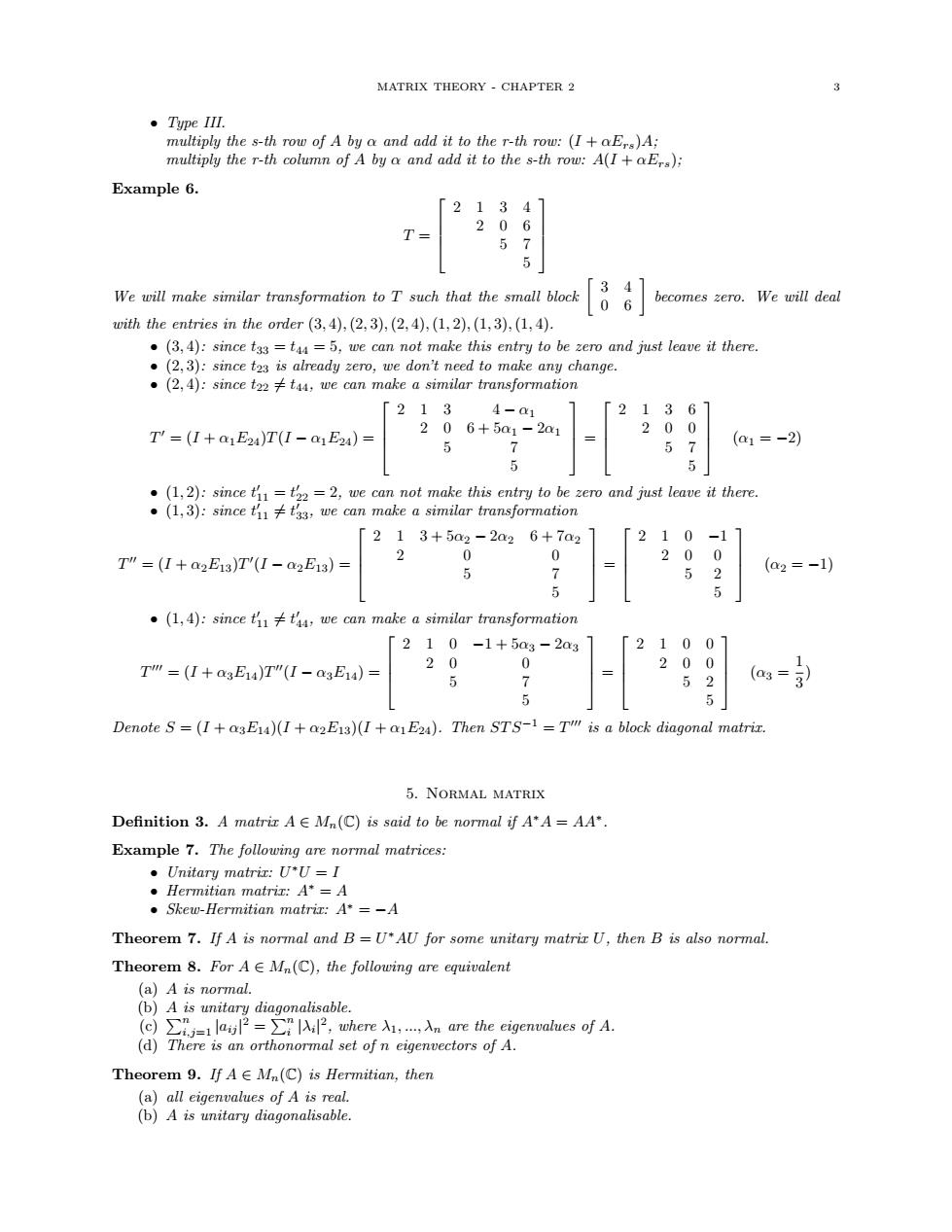
MATRIX THEORY CHAPTER 2 3 ·Tpe the s-th ro n of a b o and add it to the r-th (I+aE)A multiply the r-th column of A by and add it to the s-th row:A(): Example 6. T- 5 We will make similr trumsformation tsuch that the small bockbecomes sero.We ill deal with the entries in the order(3,4),(2,3),(2,4),(1,2),(1,3),(L,4). .(3,4):since tgs=t=5,we can not make this entry to be zero and just leave it there. 。（2,4):since t22丰t4,ve can m e a similar transformation 213 4-a1 「21361 T'-(I+aE24)T(I-E)= 206+5a1-2a1 200 0 5 (a--2) (1,2):since=2,we can not make this entry to be zero and just leave it there. 。（,3):since t1≠tg,ve can make a similar tran.sformation 「213+5a2-2a26+7a21「210-1 T"=(I+a2E13)T'(I-a2E1g)= 2 0 0 200 5 (a2=-1) .(1,4):since we can make a similar transformation 210-1+5a-2ag 21001 T"M=(I+a3E14)T"(I-a3E1)= 20 200 5 7 52 (ag=) 5 5 Denote S=(I+a3E)(I+2E3)(I+E2).Then STS-1=T"is a block diagonal matrir 5.NORMAL MATRIX Definition 3.A matrir AE M(C)is said to be normal if A'A=AA" Example 7.The following are normal matrices: .Unitaru matrir:U'U =I 。Hermitian matrir:.A'=A .Skew-Hermitian matrir:A*=-A Theorem 7.If A is normal and B=UAU for some unitary matrir U,then B is also normal Theorem 8.For AEM(C),the following are equivalent mal (∑ thA-人re te时A (d)There is an orthonormal set ofn eigenvectors of A. Theorem 9.If AE M(C)is Hermitian,then MATRIX THEORY - CHAPTER 2 3 • Type III. multiply the s-th row of A by α and add it to the r-th row: (I + αErs)A; multiply the r-th column of A by α and add it to the s-th row: A(I + αErs); Example 6. T =     2 1 3 4 2 0 6 5 7 5     We will make similar transformation to T such that the small block  3 4 0 6  becomes zero. We will deal with the entries in the order (3, 4),(2, 3),(2, 4),(1, 2),(1, 3),(1, 4). • (3, 4): since t33 = t44 = 5, we can not make this entry to be zero and just leave it there. • (2, 3): since t23 is already zero, we don’t need to make any change. • (2, 4): since t22 6= t44, we can make a similar transformation T 0 = (I + α1E24)T(I − α1E24) =     2 1 3 4 − α1 2 0 6 + 5α1 − 2α1 5 7 5     =     2 1 3 6 2 0 0 5 7 5     (α1 = −2) • (1, 2): since t 0 11 = t 0 22 = 2, we can not make this entry to be zero and just leave it there. • (1, 3): since t 0 11 6= t 0 33, we can make a similar transformation T 00 = (I + α2E13)T 0 (I − α2E13) =     2 1 3 + 5α2 − 2α2 6 + 7α2 2 0 0 5 7 5     =     2 1 0 −1 2 0 0 5 2 5     (α2 = −1) • (1, 4): since t 0 11 6= t 0 44, we can make a similar transformation T 000 = (I + α3E14)T 00(I − α3E14) =     2 1 0 −1 + 5α3 − 2α3 2 0 0 5 7 5     =     2 1 0 0 2 0 0 5 2 5     (α3 = 1 3 ) Denote S = (I + α3E14)(I + α2E13)(I + α1E24). Then ST S−1 = T 000 is a block diagonal matrix. 5. Normal matrix Definition 3. A matrix A ∈ Mn(C) is said to be normal if A∗A = AA∗ . Example 7. The following are normal matrices: • Unitary matrix: U ∗U = I • Hermitian matrix: A∗ = A • Skew-Hermitian matrix: A∗ = −A Theorem 7. If A is normal and B = U ∗AU for some unitary matrix U, then B is also normal. Theorem 8. For A ∈ Mn(C), the following are equivalent (a) A is normal. (b) A is unitary diagonalisable. (c) Pn i,j=1 |aij | 2 = Pn i |λi | 2 , where λ1, ..., λn are the eigenvalues of A. (d) There is an orthonormal set of n eigenvectors of A. Theorem 9. If A ∈ Mn(C) is Hermitian, then (a) all eigenvalues of A is real. (b) A is unitary diagonalisable