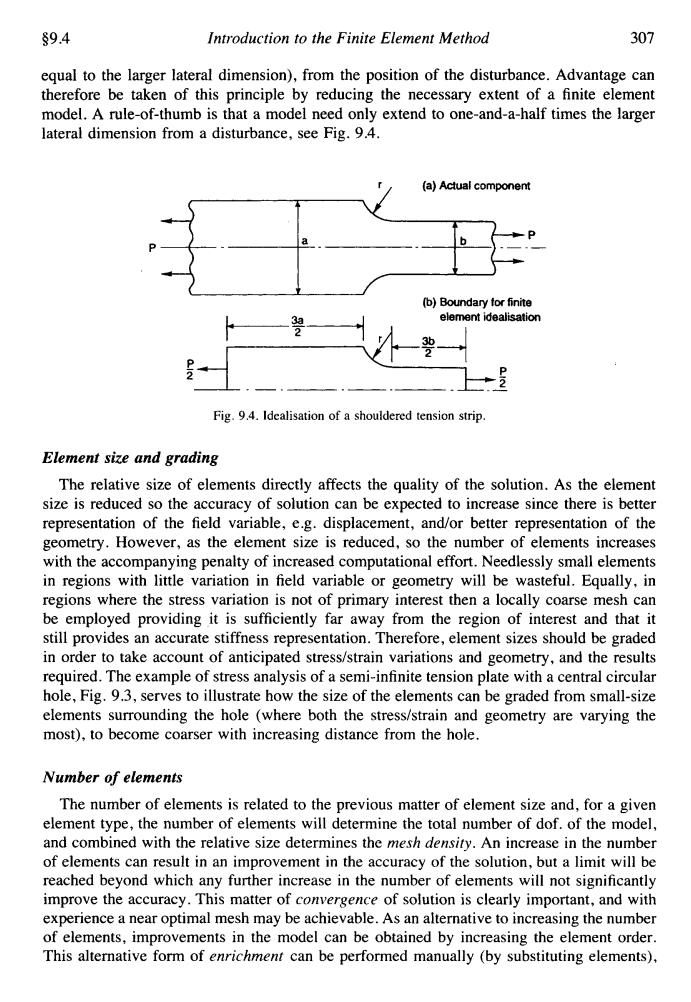
$9.4 Introduction to the Finite Element Method 307 equal to the larger lateral dimension),from the position of the disturbance.Advantage can therefore be taken of this principle by reducing the necessary extent of a finite element model.A rule-of-thumb is that a model need only extend to one-and-a-half times the larger lateral dimension from a disturbance,see Fig.9.4. (a)Actual component (b)Boundary for finite element idealisation 3b Fig.9.4.Idealisation of a shouldered tension strip. Element size and grading The relative size of elements directly affects the quality of the solution.As the element size is reduced so the accuracy of solution can be expected to increase since there is better representation of the field variable,e.g.displacement,and/or better representation of the geometry.However,as the element size is reduced,so the number of elements increases with the accompanying penalty of increased computational effort.Needlessly small elements in regions with little variation in field variable or geometry will be wasteful.Equally,in regions where the stress variation is not of primary interest then a locally coarse mesh can be employed providing it is sufficiently far away from the region of interest and that it still provides an accurate stiffness representation.Therefore,element sizes should be graded in order to take account of anticipated stress/strain variations and geometry,and the results required.The example of stress analysis of a semi-infinite tension plate with a central circular hole,Fig.9.3,serves to illustrate how the size of the elements can be graded from small-size elements surrounding the hole (where both the stress/strain and geometry are varying the most),to become coarser with increasing distance from the hole. Number of elements The number of elements is related to the previous matter of element size and,for a given element type,the number of elements will determine the total number of dof.of the model, and combined with the relative size determines the mesh density.An increase in the number of elements can result in an improvement in the accuracy of the solution,but a limit will be reached beyond which any further increase in the number of elements will not significantly improve the accuracy.This matter of convergence of solution is clearly important,and with experience a near optimal mesh may be achievable.As an alternative to increasing the number of elements,improvements in the model can be obtained by increasing the element order. This alternative form of enrichment can be performed manually (by substituting elements),99.4 Introduction to the Finite Element Method 307 equal to the larger lateral dimension), from the position of the disturbance. Advantage can therefore be taken of this principle by reducing the necessary extent of a finite element model. A rule-of-thumb is that a model need only extend to one-and-a-half times the larger lateral dimension from a disturbance, see Fig. 9.4. (a) Actual component P +q7;TEy- (b) Boundary for finite element idealition _p 2 ._.___.__.____ Fig. 9.4. ldealisation of a shouldered tension strip. Element size and grading The relative size of elements directly affects the quality of the solution. As the element size is reduced so the accuracy of solution can be expected to increase since there is better representation of the field variable, e.g. displacement, and/or better representation of the geometry. However, as the element size is reduced, so the number of elements increases with the accompanying penalty of increased computational effort. Needlessly small elements in regions with little variation in field variable or geometry will be wasteful. Equally, in regions where the stress variation is not of primary interest then a locally coarse mesh can be employed providing it is sufficiently far away from the region of interest and that it still provides an accurate stiffness representation. Therefore, element sizes should be graded in order to take account of anticipated stresshtrain variations and geometry, and the results required. The example of stress analysis of a semi-infinite tension plate with a central circular hole, Fig. 9.3, serves to illustrate how the size of the elements can be graded from small-size elements surrounding the hole (where both the stresdstrain and geometry are varying the most), to become coarser with increasing distance from the hole. Number of elements The number of elements is related to the previous matter of element size and, for a given element type, the number of elements will determine the total number of dof. of the model, and combined with the relative size determines the mesh density. An increase in the number of elements can result in an improvement in the accuracy of the solution, but a limit will be reached beyond which any further increase in the number of elements will not significantly improve the accuracy. This matter of convergence of solution is clearly important, and with experience a near optimal mesh may be achievable. As an alternative to increasing the number of elements, improvements in the model can be obtained by increasing the element order. This alternative form of enrichment can be performed manually (by substituting elements)