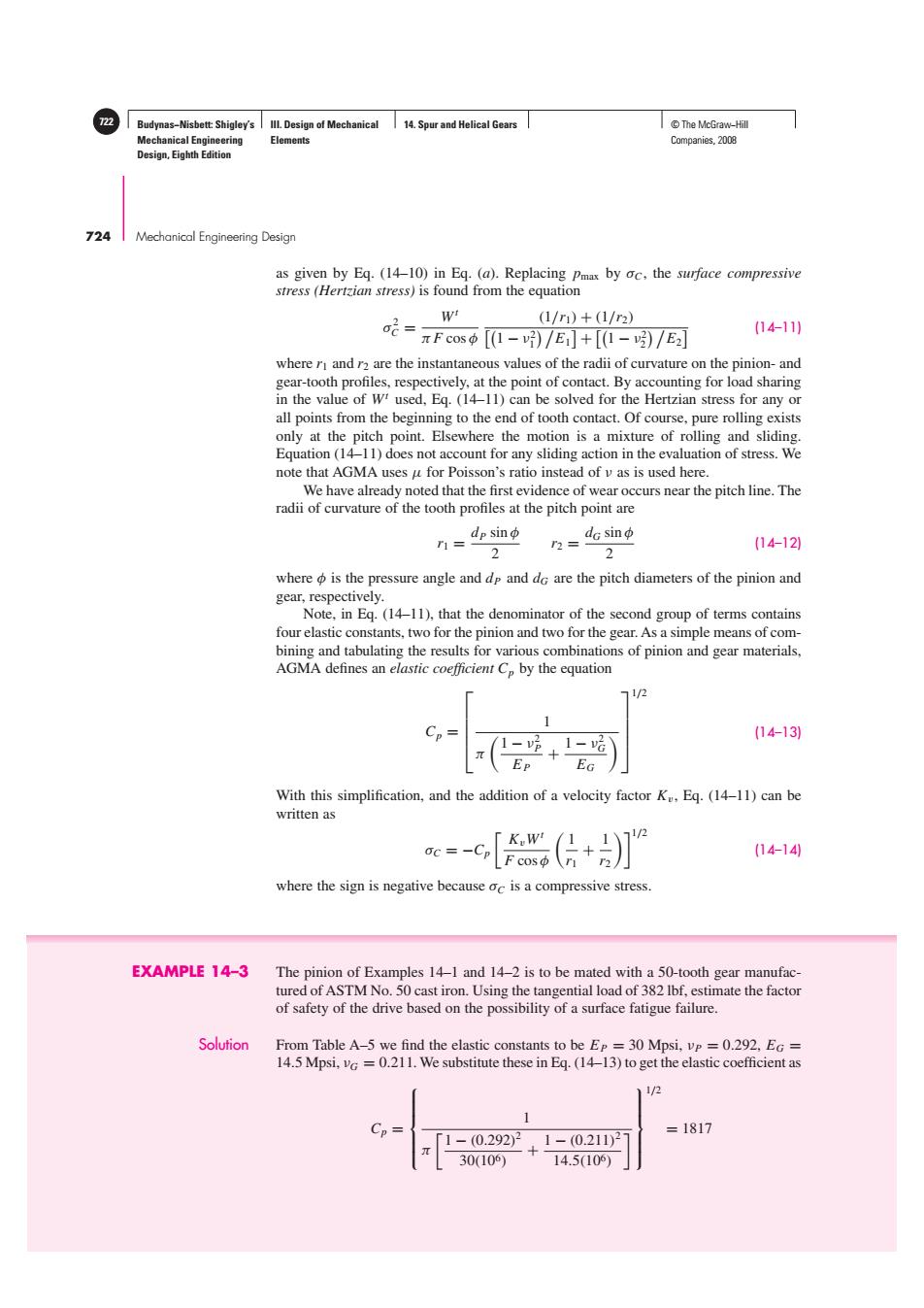
722 Budynas-Nisbett:Shigley's IIL Design of Mechanical 14.Spur and Helical Gears T©The McGraw-Hil Mechanical Engineering Elements Companies,2008 Design,Eighth Edition 724 Mechanical Engineering Design as given by Eq.(14-10)in Eq.(a).Replacing Pmax by oc,the surface compressive stress(Hertzian stress)is found from the equation (1/r)+(1/r2) 2= (14-11) -πFcos中[1-)/E1]+[(1-)/E2] where r and r2 are the instantaneous values of the radii of curvature on the pinion-and gear-tooth profiles,respectively,at the point of contact.By accounting for load sharing in the value of W used,Eg.(14-11)can be solved for the Hertzian stress for any or all points from the beginning to the end of tooth contact.Of course,pure rolling exists only at the pitch point.Elsewhere the motion is a mixture of rolling and sliding. Equation(14-11)does not account for any sliding action in the evaluation of stress.We note that AGMA uses u for Poisson's ratio instead of v as is used here. We have already noted that the first evidence of wear occurs near the pitch line.The radii of curvature of the tooth profiles at the pitch point are r=desing 2 r2=da sind (14-12) 2 where o is the pressure angle and dp and dc are the pitch diameters of the pinion and gear,respectively. Note,in Eq.(14-11),that the denominator of the second group of terms contains four elastic constants,two for the pinion and two for the gear.As a simple means of com- bining and tabulating the results for various combinations of pinion and gear materials, AGMA defines an elastic coefficient Cp by the equation 1/2 (14-13) EP EG With this simplification,and the addition of a velocity factor K,Eq.(14-11)can be written as c-c[(+】 (14-14) where the sign is negative because oc is a compressive stress. EXAMPLE 14-3 The pinion of Examples 14-1 and 14-2 is to be mated with a 50-tooth gear manufac- tured of ASTM No.50 cast iron.Using the tangential load of 382 Ibf,estimate the factor of safety of the drive based on the possibility of a surface fatigue failure. Solution From Table A-5 we find the elastic constants to be Ep =30 Mpsi,vp =0.292,EG 14.5 Mpsi,v =0.211.We substitute these in Eq.(14-13)to get the elastic coefficient as 1/2 1 C =1817 「1-(0.292)2,1-(0.211)2 30(106) 14.5(10)Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition III. Design of Mechanical Elements 14. Spur and Helical Gears 722 © The McGraw−Hill Companies, 2008 724 Mechanical Engineering Design as given by Eq. (14–10) in Eq. (a). Replacing pmax by σC , the surface compressive stress (Hertzian stress) is found from the equation σ2 C = Wt π F cos φ (1/r1) + (1/r2) 1 − ν2 1 E1  + 1 − ν2 2 E2  (14–11) where r1 and r2 are the instantaneous values of the radii of curvature on the pinion- and gear-tooth profiles, respectively, at the point of contact. By accounting for load sharing in the value of Wt used, Eq. (14–11) can be solved for the Hertzian stress for any or all points from the beginning to the end of tooth contact. Of course, pure rolling exists only at the pitch point. Elsewhere the motion is a mixture of rolling and sliding. Equation (14–11) does not account for any sliding action in the evaluation of stress. We note that AGMA uses μ for Poisson’s ratio instead of ν as is used here. We have already noted that the first evidence of wear occurs near the pitch line. The radii of curvature of the tooth profiles at the pitch point are r1 = dP sin φ 2 r2 = dG sin φ 2 (14–12) where φ is the pressure angle and dP and dG are the pitch diameters of the pinion and gear, respectively. Note, in Eq. (14–11), that the denominator of the second group of terms contains four elastic constants, two for the pinion and two for the gear. As a simple means of com￾bining and tabulating the results for various combinations of pinion and gear materials, AGMA defines an elastic coefficient Cp by the equation Cp = ⎡ ⎢ ⎢ ⎢ ⎣ 1 π 1 − ν2 P EP + 1 − ν2 G EG  ⎤ ⎥ ⎥ ⎥ ⎦ 1/2 (14–13) With this simplification, and the addition of a velocity factor Kv , Eq. (14–11) can be written as σC = −Cp  KvWt F cos φ  1 r1 + 1 r2 1/2 (14–14) where the sign is negative because σC is a compressive stress. EXAMPLE 14–3 The pinion of Examples 14–1 and 14–2 is to be mated with a 50-tooth gear manufac￾tured of ASTM No. 50 cast iron. Using the tangential load of 382 lbf, estimate the factor of safety of the drive based on the possibility of a surface fatigue failure. Solution From Table A–5 we find the elastic constants to be EP = 30 Mpsi, νP = 0.292, EG = 14.5 Mpsi, νG = 0.211. We substitute these in Eq. (14–13) to get the elastic coefficient as Cp = ⎧ ⎪⎪⎪⎨ ⎪⎪⎪⎩ 1 π  1 − (0.292)2 30(106) + 1 − (0.211)2 14.5(106)  ⎫ ⎪⎪⎪⎬ ⎪⎪⎪⎭ 1/2 = 1817