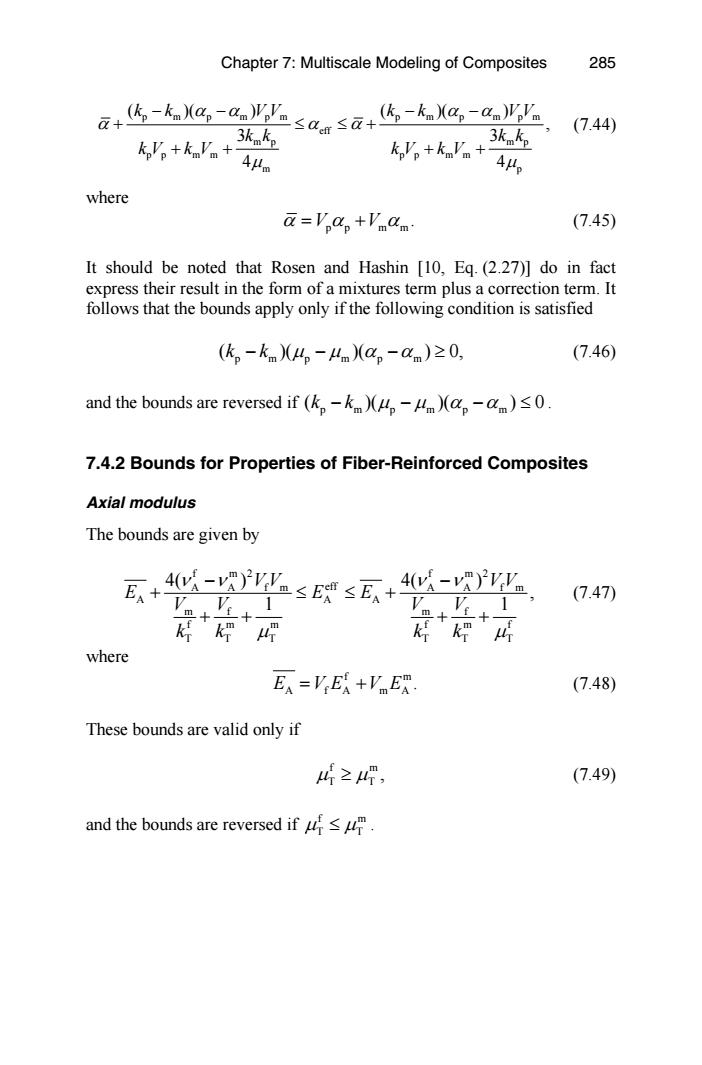
Chapter 7:Multiscale Modeling of Composites 285 g6,-kaX,-avsoah-kaXa,-ayV 3kmkp (7.44) k,'。+km'm+ 3kmkp 44m kolp+km!m+ 4μp where a='a。+Vnam (7.45) It should be noted that Rosen and Hashin [10,Eq.(2.27)]do in fact express their result in the form of a mixtures term plus a correction term.It follows that the bounds apply only if the following condition is satisfied (k。-km)4。-4m(a。-am)≥0， (7.46) and the bounds are reversed if(k,-km)(。-lm)(a。-am)≤0. 7.4.2 Bounds for Properties of Fiber-Reinforced Composites Axial modulus The bounds are given by Es+ -vW≤E≤E+ 4y-yΨ m++1 (7.47) m十 十 ” ki ki A where EA=VEA+VER (7.48) These bounds are valid only if ≥， (7.49) and the bounds are reversed ifusu.p m p m pm p m p m pm eff m p m p pp mm pp mm m p ( )( ) ( )( ) , 3 3 4 4 k k VV k k VV k k k k kV k V kV k V α α α α α α α µ µ − − − − + ≤ ≤ + + + + + (7.44) where p p mm α =V V α α + . (7.45) It should be noted that Rosen and Hashin [10, Eq. (2.27)] do in fact express their result in the form of a mixtures term plus a correction term. It follows that the bounds apply only if the following condition is satisfied pmp mp m ( )( )( ) 0, k k − − −≥ µ µαα (7.46) and the bounds are reversed if pmp mp m ( )( )( ) 0 k k − µ − −≤ µαα . 7.4.2 Bounds for Properties of Fiber-Reinforced Composites Axial modulus The bounds are given by f m2 f m2 A A fm eff A A fm A A A m f m f fmm fmf TT T TT T 4( ) 4( ) , 1 1 V V V V E E E V V V V k k k k ν ν ν ν µ µ − − + ≤ ≤ + + + + + (7.47) where f m A f A mA E VE V E = + . (7.48) These bounds are valid only if f m T T µ ≥ µ , (7.49) and the bounds are reversed if f m µ T T ≤ µ . Chapter 7: Multiscale Modeling of Composites 285