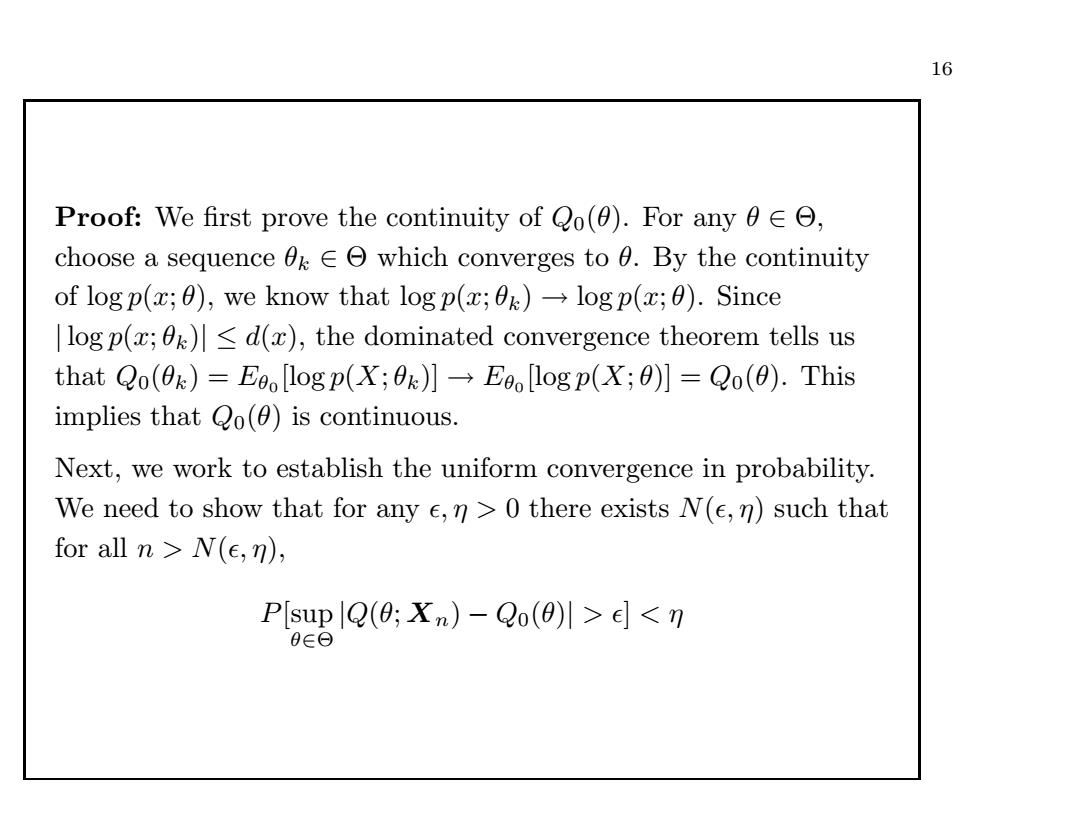
16 Proof:We first prove the continuity of Qo().For any 0, choose a sequence 0ee which converges to 0.By the continuity of logp(x;0),we know that logp(x;)logp(x;0).Since |logp(z;0)<d(z),the dominated convergence theorem tells us that Qo(x)=E0o[logp(X;0)]-E0ollogp(X;0)]=Qo(0).This implies that Qo(0)is continuous. Next,we work to establish the uniform convergence in probability. We need to show that for any e,n>0 there exists N(e,n)such that for all nN(e,n), P[sup Q(0;Xn)-Qo(0)I >e]<n 0∈Θ16 Proof: We first prove the continuity of Q0(θ). For any θ ∈ Θ, choose a sequence θk ∈ Θ which converges to θ. By the continuity of log p(x; θ), we know that log p(x; θk) → log p(x; θ). Since | log p(x; θk)| ≤ d(x), the dominated convergence theorem tells us that Q0(θk) = Eθ0 [log p(X; θk)] → Eθ0 [log p(X; θ)] = Q0(θ). This implies that Q0(θ) is continuous. Next, we work to establish the uniform convergence in probability. We need to show that for any , η > 0 there exists N(, η) such that for all n>N(, η), P[sup θ∈Θ |Q(θ; Xn) − Q0(θ)| > ] < η