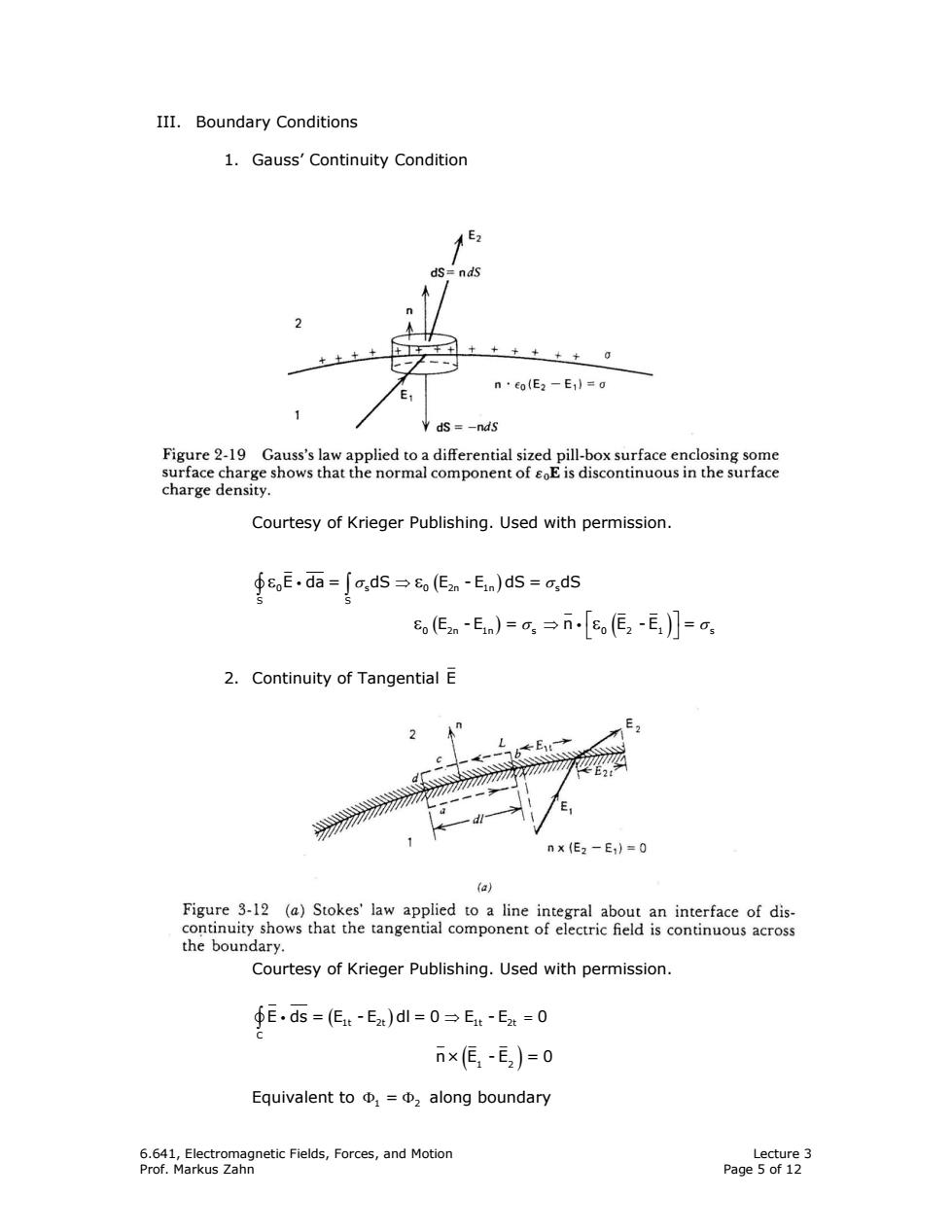
III.Boundary Conditions 1.Gauss'Continuity Condition 才 ds=nds ，+ 十 + n·co(E2-E)=o Y ds =-nds Figure 2-19 Gauss's law applied to a differential sized pill-box surface enclosing some surface charge shows that the normal component of eoE is discontinuous in the surface charge density. Courtesy of Krieger Publishing.Used with permission. Ed-[adS-E)dS-a.dS 8(En-E)=a→n…[8(2-】=a 2.Continuity of Tangential E E ←E2t nx(E2-E1)=0 (a) Figure 3-12 (a)Stokes'law applied to a line integral about an interface of dis- continuity shows that the tangential component of electric field is continuous across the boundary. Courtesy of Krieger Publishing.Used with permission. ∮E.ds=(Et-E2x)dl=0→Et-Ex=0 n×(尼-E2)=0 Equivalent toΦ，=Φ2 along boundary 6.641,Electromagnetic Fields,Forces,and Motion Lecture 3 Prof.Markus Zahn Page 5 of 126.641, Electromagnetic Fields, Forces, and Motion Lecture 3 Prof. Markus Zahn Page 5 of 12 III. Boundary Conditions 1. Gauss’ Continuity Condition Courtesy of Krieger Publishing. Used with permission. 0 s 0 2n 1n s ( ) S S v∫ ∫ ε ε E da = dS E - E dS = dS i σ σ ⇒ 0 2n 1n s 0 s ( ) E -E = n E -E = ( 2 1 ) ⇒ ⎡ ⎤ ⎣ ⎦ ε ε σ i σ 2. Continuity of Tangential E Courtesy of Krieger Publishing. Used with permission. ( ) 1t 2t 1t 2t C E ds = E - E dl = 0 E - E 0 ⇒ = ∫ i v n× E -E = 0 ( 1 2 ) Equivalent to Φ Φ 1 2 = along boundary