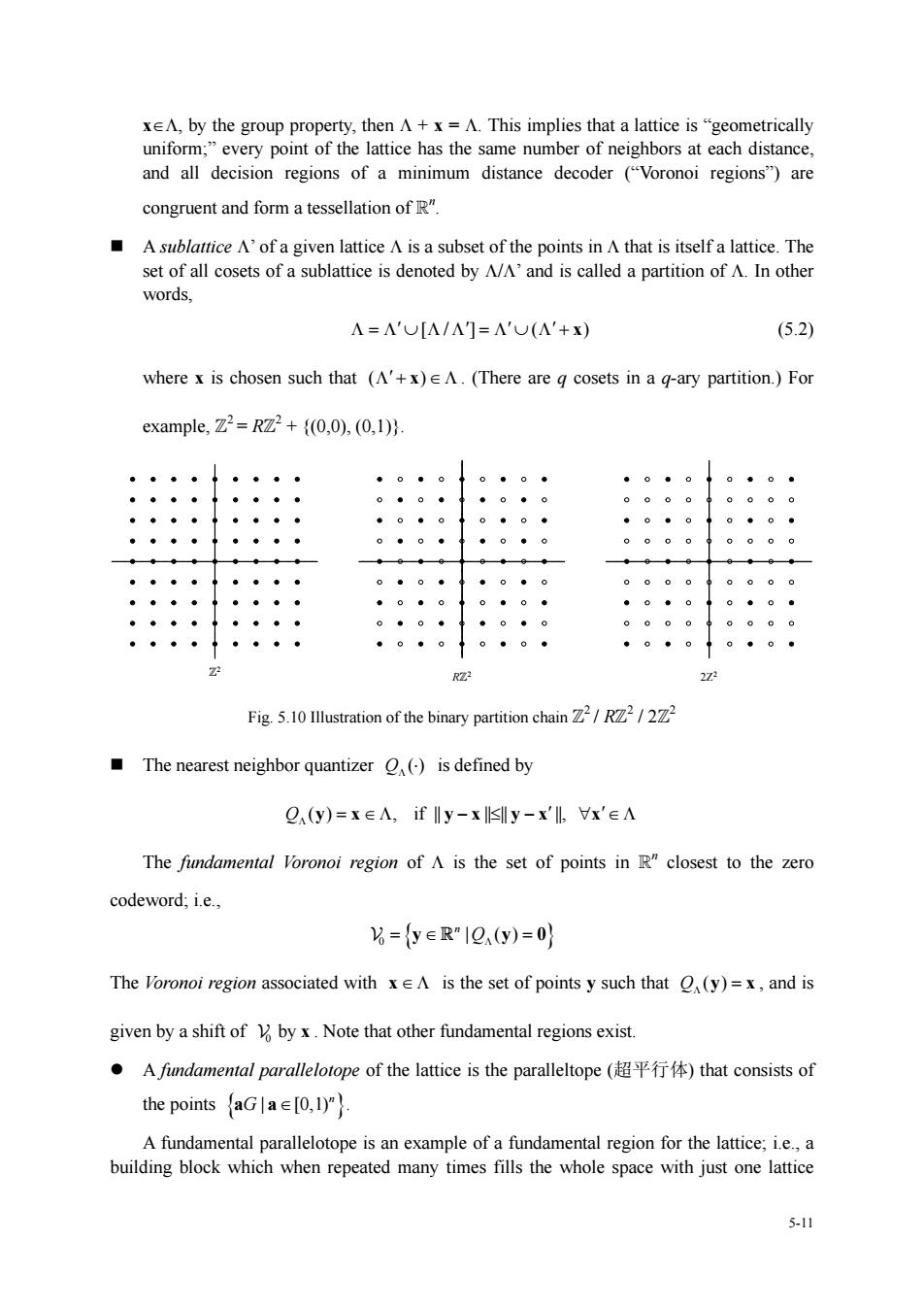
xEA,by the group property,then A+x=A.This implies that a lattice is"geometrically uniform,”every nt of the lattice has the same number of neighbors at each distance. and all decision regions of a minimum distance decode ("Voronoi regions")are congruent and form a tessellation ofR" Asublattice A'ofa given lattice A is a subset of the points in A that is itself a lattice.The lcostsof a sublattice is denoted by and is called a partition ofnot A=AO[A/AT=AU(A'+x) (5.2) where x is chosen such that (A'+x)A.(There are g cosets in a q-ary partition.)For example,2=RZ2+(0,0).(0,1)) ·。·。· 。 。 。 。年华年。海年 。&。0 ●年。●。 ●0●。0◆0 222 Fig 5.10 llustration of the binary partition chainR The nearest neighbor quantizer (is defined by e(y)=xEA,if lly-xlslly-x'll,Vx'EA The fiundamental Voronoi region of A is the set of points in R"closest to the zero codeword;i.e., 名={y∈R"I2y)=0 The Voronoi region associated with x is the set of points y such that (y)x,and is given by a shift of by x.Note that other fundamental regions exist. ●A fundamental parallelotope of the lattice is the paralleltope(超平行体)that consists of the points{aGla∈[0，lyr} A fundamental parallelotope is an example of a fundamental region for the lattice;i.e.,a building block which when repeated many times fills the whole space with just one lattice 5-10 5-11 x∈Λ, by the group property, then Λ + x = Λ. This implies that a lattice is “geometrically uniform;” every point of the lattice has the same number of neighbors at each distance, and all decision regions of a minimum distance decoder (“Voronoi regions”) are congruent and form a tessellation of Rn . „ A sublattice Λ’ of a given lattice Λ is a subset of the points in Λ that is itself a lattice. The set of all cosets of a sublattice is denoted by Λ/Λ’ and is called a partition of Λ. In other words, Λ=Λ ∪ Λ Λ =Λ ∪ Λ + ′ [/ ] ( ) ′′′ x (5.2) where x is chosen such that ( ) Λ + ∈Λ ′ x . (There are q cosets in a q-ary partition.) For example, Z2 = RZ2 + {(0,0), (0,1)}. Z2 RZ2 2Z2 Fig. 5.10 Illustration of the binary partition chain Z2 / RZ2 / 2Z2 „ The nearest neighbor quantizer ( ) QΛ ⋅ is defined by Q ( ) , if || || || ||, Λ y x yx yx x = ∈Λ − ≤ − ∀ ∈Λ ′ ′ The fundamental Voronoi region of Λ is the set of points in Rn closest to the zero codeword; i.e., 0 { | () } n = ∈ = QΛ V y y R 0 The Voronoi region associated with x ∈Λ is the set of points y such that ( ) QΛ y = x , and is given by a shift of 0 V by x . Note that other fundamental regions exist. z A fundamental parallelotope of the lattice is the paralleltope (超平行体) that consists of the points { | [0,1) }n a a G ∈ . A fundamental parallelotope is an example of a fundamental region for the lattice; i.e., a building block which when repeated many times fills the whole space with just one lattice