正在加载图片...
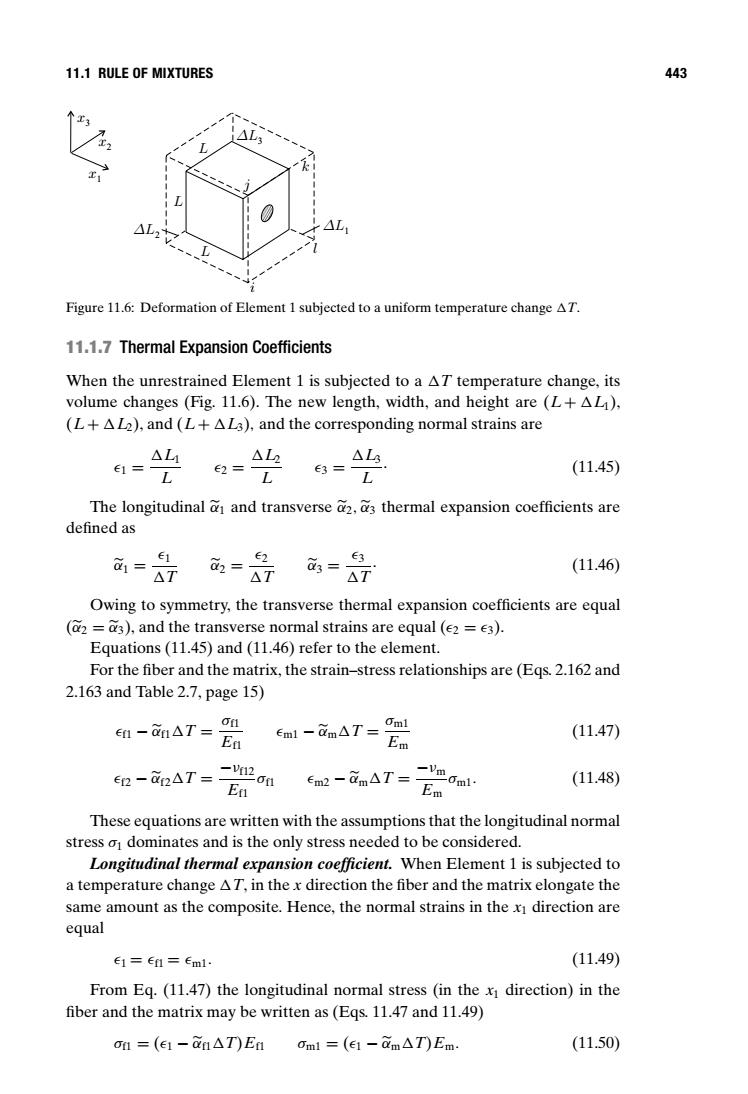
11.1 RULE OF MIXTURES 443 △L3 △L2 AL Figure 11.6:Deformation of Element 1 subjected to a uniform temperature change AT. 11.1.7 Thermal Expansion Coefficients When the unrestrained Element 1 is subjected to a AT temperature change,its volume changes(Fig.11.6).The new length,width,and height are (L+AL), (L+AL2),and (L+AL3),and the corresponding normal strains are LM e=L AL e2=L △L (11.45) The longitudinal and transverse @2,@3 thermal expansion coefficients are defined as a=1 T 应= △T a=3 (11.46) T Owing to symmetry,the transverse thermal expansion coefficients are equal (2=@3),and the transverse normal strains are equal (e2 =e3). Equations(11.45)and (11.46)refer to the element. For the fiber and the matrix,the strain-stress relationships are(Eqs.2.162 and 2.163 and Table 2.7,page 15) -ai△T=o (11.47) Ea em1-am△T=oml Em R-△T=E置ma-AT= 0m1 (11.48) Ea Em These equations are written with the assumptions that the longitudinal normal stress o dominates and is the only stress needed to be considered. Longitudinal thermal expansion coefficient.When Element 1 is subjected to a temperature change A T,in the x direction the fiber and the matrix elongate the same amount as the composite.Hence,the normal strains in the x direction are equal e1=ef1=eml· (11.49) From Eq.(11.47)the longitudinal normal stress (in the x direction)in the fiber and the matrix may be written as (Eqs.11.47 and 11.49) o0=(e1-a△T)Eom1=(e1-am△T)Em (11.50)11.1 RULE OF MIXTURES 443 L ∆L1 L i j k L l ∆L2 ∆L3 x1 x3 x2 Figure 11.6: Deformation of Element 1 subjected to a uniform temperature change
T. 11.1.7 Thermal Expansion Coefficients When the unrestrained Element 1 is subjected to a
T temperature change, its volume changes (Fig. 11.6). The new length, width, and height are (L+
L1), (L+
L2), and (L+
L3), and the corresponding normal strains are 1 =
L1 L 2 =
L2 L 3 =
L3 L . (11.45) The longitudinal α1 and transverse α2,α3 thermal expansion coefficients are defined as α1 = 1
T α2 = 2
T α3 = 3
T . (11.46) Owing to symmetry, the transverse thermal expansion coefficients are equal (α2 = α3), and the transverse normal strains are equal (2 = 3). Equations (11.45) and (11.46) refer to the element. For the fiber and the matrix, the strain–stress relationships are (Eqs. 2.162 and 2.163 and Table 2.7, page 15) f1 − αf1
T = σf1 Ef1 m1 − αm
T = σm1 Em (11.47) f2 − αf2
T = −νf12 Ef1 σf1 m2 − αm
T = −νm Em σm1. (11.48) These equations are written with the assumptions that the longitudinal normal stress σ1 dominates and is the only stress needed to be considered. Longitudinal thermal expansion coefficient. When Element 1 is subjected to a temperature change
T, in the x direction the fiber and the matrix elongate the same amount as the composite. Hence, the normal strains in the x1 direction are equal 1 = f1 = m1. (11.49) From Eq. (11.47) the longitudinal normal stress (in the x1 direction) in the fiber and the matrix may be written as (Eqs. 11.47 and 11.49) σf1 = (1 − αf1
T)Ef1 σm1 = (1 − αm
T)Em. (11.50)��������������