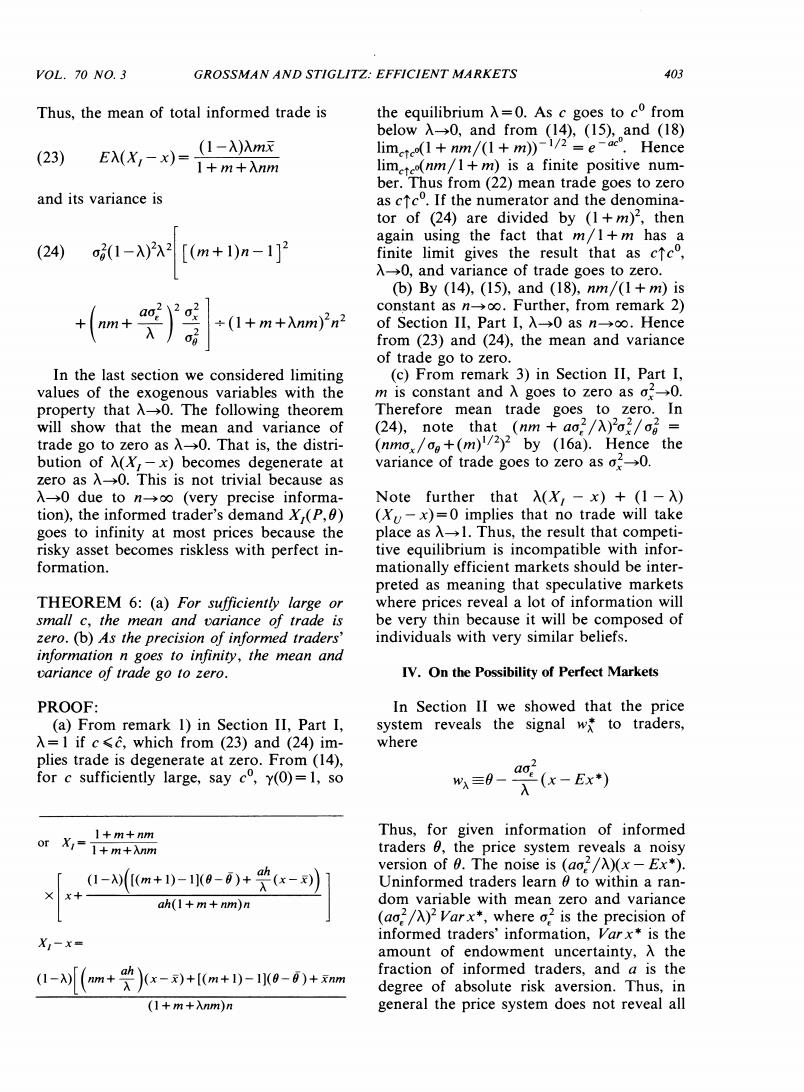
VOL.70N0.3 GROSSMAN AND STIGLITZ:EFFICIENT MARKETS 403 Thus,the mean of total informed trade is the equilibrium A=0.As c goes to co from below入→0，and from(l4),(l5),and(18) (23) EA(XI-x)= (1-入)入mx limcte(1+nm/(1+m))-1/2=e-ac.Hence 1+m+入nm limto(nm/1+m)is a finite positive num- ber.Thus from(22)mean trade goes to zero and its variance is as ctco.If the numerator and the denomina- tor of (24)are divided by (1+m),then again using the fact that m/1+m has a 24) 1-%2 [(m+1)n-1]2 finite limit gives the result that as cco, λ→0，and variance of trade goes to zero. b)By(14),(15),and(18),nm/(1+m)is m+笑 constant as noo.Further,from remark 2) ÷(1+m+入nm)2n2 of Section II,Part I,λ→0asn→o.Hence from (23)and (24),the mean and variance of trade go to zero. In the last section we considered limiting (c)From remark 3)in Section II,Part I, values of the exogenous variables with the m is constant and A goes to zero as o0. property that入→0.The following theorem Therefore mean trade goes to zero.In will show that the mean and variance of (24),note that (nm ao2/A)22/ag= trade go to zero as A-0.That is,the distri- (nmox/0+(m)/2)2 by (16a).Hence the bution of A(X,-x)becomes degenerate at variance of trade goes to zero as o0. zero as入→0.This is not trivial because as 入→0 due to n→o(very precise informa- Note further that A(X-x)+(1-A) tion),the informed trader's demand X (P,0) (Xu-x)=0 implies that no trade will take goes to infinity at most prices because the place as入→l.Thus,the result that competi- risky asset becomes riskless with perfect in- tive equilibrium is incompatible with infor- formation. mationally efficient markets should be inter- preted as meaning that speculative markets THEOREM 6:(a)For sufficiently large or where prices reveal a lot of information will small c,the mean and variance of trade is be very thin because it will be composed of zero.(b)As the precision of informed traders' individuals with very similar beliefs. information n goes to infinity,the mean and variance of trade go to zero. IV.On the Possibility of Perfect Markets PROOF: In Section II we showed that the price (a)From remark 1)in Section II,Part I, system reveals the signal w*to traders, λ=1ifc≤c,which from(23)and(24)im where plies trade is degenerate at zero.From (14), for c sufficiently large,say co,y(0)=1,so (Ex) 1+m+nm Thus,for given information of informed or X1=1+m+Anm traders 6,the price system reveals a noisy version of 6.The noise is (aoA)(x-Ex*). -m+)-0-)+(x-) Uninformed traders learn 0 to within a ran- x+ ah( +m+nm)n dom variable with mean zero and variance (a2/A)2Varx*,where o2 is the precision of informed traders'information,Varx*is the amount of endowment uncertainty,A the 1-[(am+）)x-)+m+)-(-)+mm fraction of informed traders,and a is the degree of absolute risk aversion.Thus,in (1+m+入nm)n general the price system does not reveal all