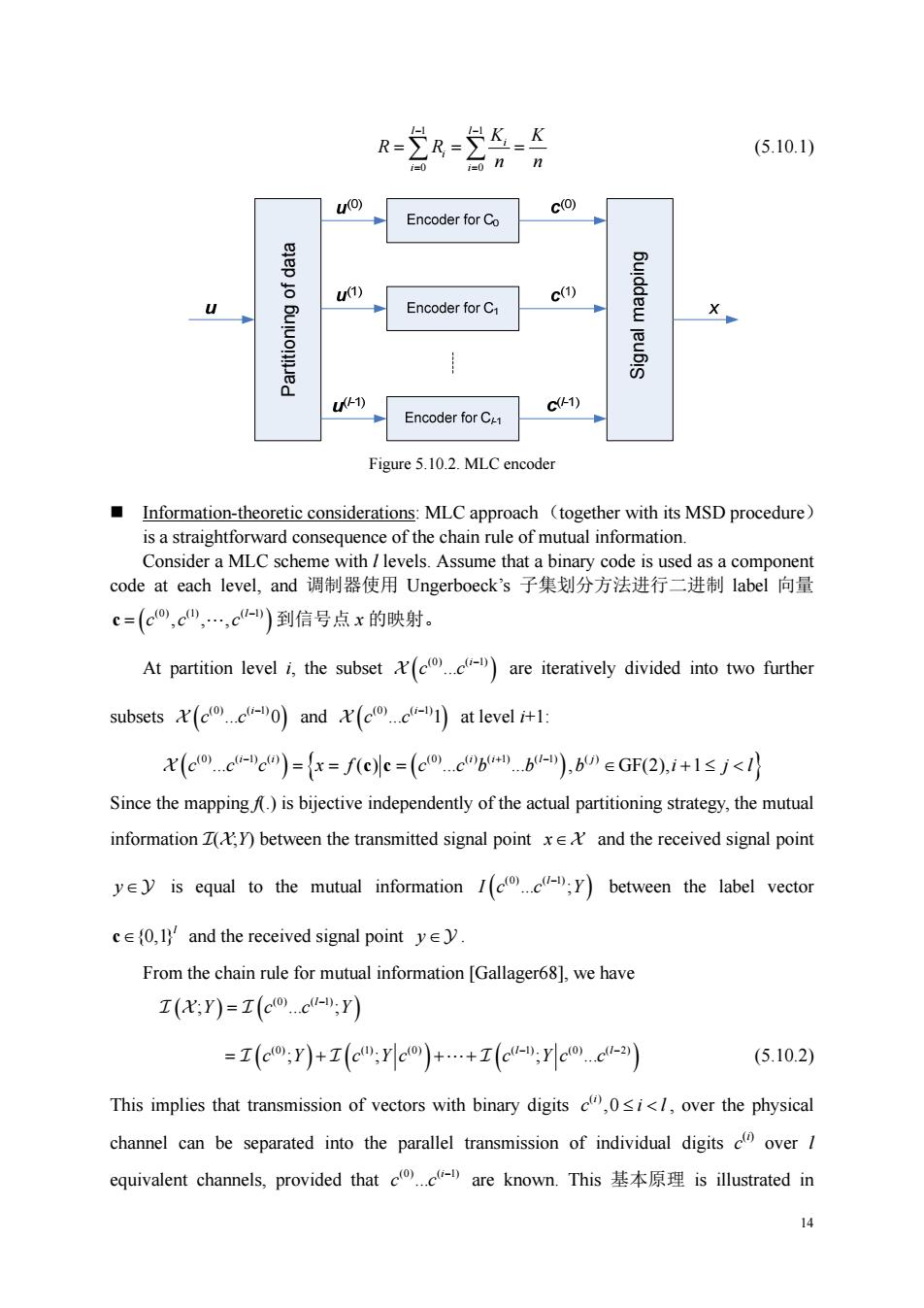
-2会- (5.10.1) =0 A)Encoder forC C) u1) -Encoder for Ca c(1) X leu6!S Encoder for C Figure 5.10.2.MLC encoder InformatiohriMLCapproac (ogether ith its MSD Proedure) is a straig a bir nary code is code at each level,and调制器使用Ungerboeck's子集划分方法进行二进制label向量 c=(c,cm,.,c-)到信号点x的映射。 At partition level i,the subset are iteratively divided into two further subsets(co.c-o）andx(coca-1）at leveli1 x(coc-cm）={x=fcc=(coc0b.b-）,b0eGF2),i+1≤j<1} Since the mappingis bijective independently of the actual partitioning strategy,the mutual information()between the transmitted signal pointx and the received signal point y)is equal to the mutual information Y)between the label vector e,l and the received signal point y From the chain rule for mutual information [Gallager68],we have T(X)=T(coc-:y） =T(co:Yy)）+T(cm:yco）++T(c-:ycoc-a） (5.10.2) This implies that transmission of vectors with binary the physical channel can be separated into the parallel transmission of individual digits c overI equivalent channels,provided that-are known.This基本原理is illustrated in14 1 1 0 0 l l i i i i K K R R n n − − = = = ∑ ∑= = (5.10.1) Partitioning of data Signal mapping Figure 5.10.2. MLC encoder „ Information-theoretic considerations: MLC approach （together with its MSD procedure） is a straightforward consequence of the chain rule of mutual information. Consider a MLC scheme with l levels. Assume that a binary code is used as a component code at each level, and 调制器使用 Ungerboeck’s 子集划分方法进行二进制 label 向量 ( ) (0) (1) ( 1) , , l cc c − c = " 到信号点 x 的映射。 At partition level i, the subset ( ) (0) ( 1) . i c c − X are iteratively divided into two further subsets ( ) (0) ( 1) . 0 i c c − X and ( ) (0) ( 1) . 1 i c c − X at level i+1: ( ) { ( ) } (0) ( 1) ( ) (0) ( ) ( 1) ( 1) ( ) . ( ) . . , GF(2), 1 i i ii l j c c c x f c cb b b i j l − +− X = = = ∈ +≤ < c c Since the mapping f(.) is bijective independently of the actual partitioning strategy, the mutual information I(X;Y) between the transmitted signal point x∈X and the received signal point y ∈Y is equal to the mutual information ( ) (0) ( 1) . ; l I ccY − between the label vector {0,1}l c∈ and the received signal point y ∈Y . From the chain rule for mutual information [Gallager68], we have ( ) ( ) (0) ( 1) ; . ; l Y ccY − IX I = ( ) ( ) ( ) (0) (1) (0) ( 1) (0) ( 2) ; ; ; . l l c Y c Yc c Yc c − − = + ++ II I " (5.10.2) This implies that transmission of vectors with binary digits ( ) ,0 i c il ≤ < , over the physical channel can be separated into the parallel transmission of individual digits c (i) over l equivalent channels, provided that (0) ( 1) . i c c − are known. This 基本原理 is illustrated in