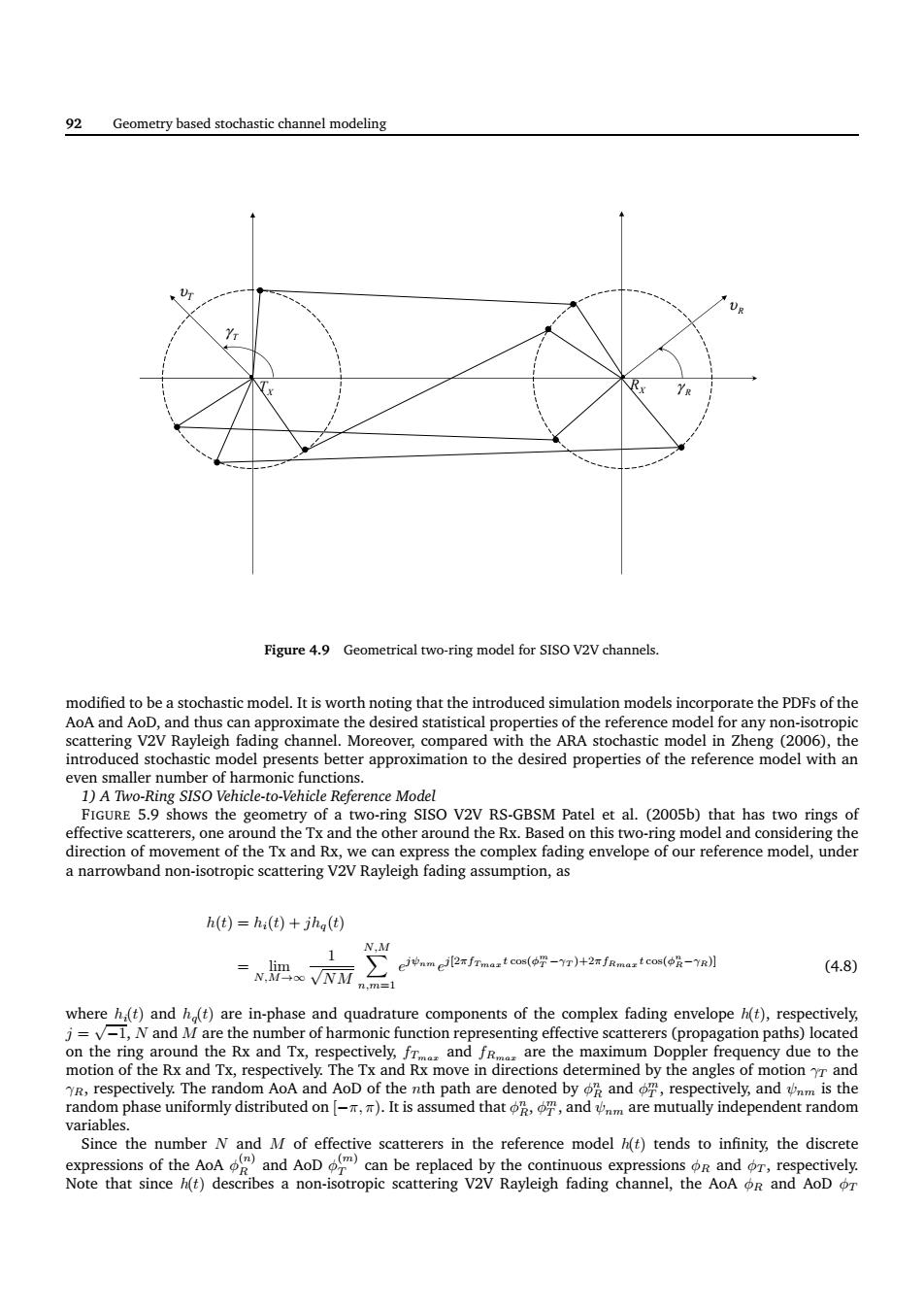
92 Geometry based stochastic channel modeling Figure 4.9 Geometrical two-ring model for SISO V2V channels modified to be a stochastic model.It is worth noting that the introduced simulation models inco orate the PDFs of the AoAandAoDandhscanapioimaietedesiedamiticapopCiesofheTeaeemodaToranyoPaopg ing channel even smaller numbe Vy BS-GBSM Patel et al (2005b)that has two rings of terers,one a oenmemtoft e Tx and ing envelope of our reference model,under a h(d)=n()+jh() A ivam(-)+(-YR) (4.8) where h(t)and ht)are in-phase and quadrature components of the complex fading envelope(t),respectively are the mmber ofharmonicfnction reprcsnting Rx and Tx nd" and .are the maximun equ cy due to the Since the number N and M of effective scatterers in the reference model)tends to infinity,the discrete and AoD 92 Geometry based stochastic channel modeling υ R υT R γ T γ TX RX Figure 4.9 Geometrical two-ring model for SISO V2V channels. modified to be a stochastic model. It is worth noting that the introduced simulation models incorporate the PDFs of the AoA and AoD, and thus can approximate the desired statistical properties of the reference model for any non-isotropic scattering V2V Rayleigh fading channel. Moreover, compared with the ARA stochastic model in Zheng (2006), the introduced stochastic model presents better approximation to the desired properties of the reference model with an even smaller number of harmonic functions. 1) A Two-Ring SISO Vehicle-to-Vehicle Reference Model FIGURE 5.9 shows the geometry of a two-ring SISO V2V RS-GBSM Patel et al. (2005b) that has two rings of effective scatterers, one around the Tx and the other around the Rx. Based on this two-ring model and considering the direction of movement of the Tx and Rx, we can express the complex fading envelope of our reference model, under a narrowband non-isotropic scattering V2V Rayleigh fading assumption, as h(t) = hi(t) + jhq(t) = lim N,M→∞ 1 √ NM N,M X n,m=1 e jψnm e j[2πfTmax t cos(φm T −γT )+2πfRmax t cos(φ n R−γR)] (4.8) where hi(t) and hq(t) are in-phase and quadrature components of the complex fading envelope h(t), respectively, j = √ −1, N and M are the number of harmonic function representing effective scatterers (propagation paths) located on the ring around the Rx and Tx, respectively, fTmax and fRmax are the maximum Doppler frequency due to the motion of the Rx and Tx, respectively. The Tx and Rx move in directions determined by the angles of motion γT and γR, respectively. The random AoA and AoD of the nth path are denoted by φ n R and φ m T , respectively, and ψnm is the random phase uniformly distributed on [−π, π). It is assumed that φ n R, φ m T , and ψnm are mutually independent random variables. Since the number N and M of effective scatterers in the reference model h(t) tends to infinity, the discrete expressions of the AoA φ (n) R and AoD φ (m) T can be replaced by the continuous expressions φR and φT , respectively. Note that since h(t) describes a non-isotropic scattering V2V Rayleigh fading channel, the AoA φR and AoD φT