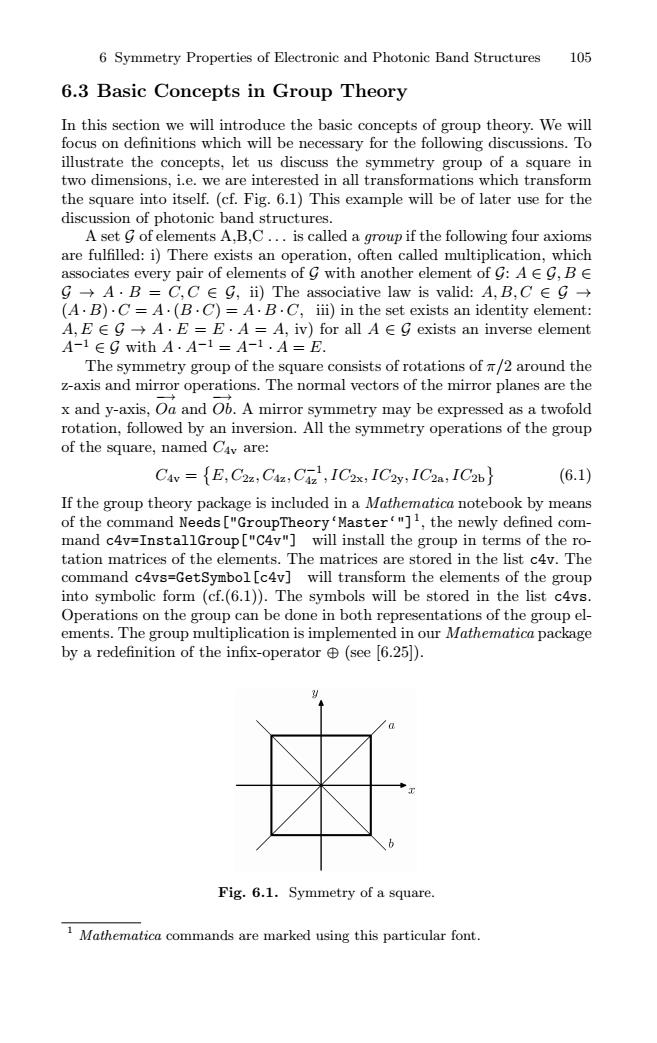
6 Symmetry Properties of Electronic and Photonic Band Structures 105 6.3 Basic Concepts in Group Theory In this section we will introduce the basic concepts of group theory.We will focus on definitions which will be necessary for the following discussions.To illustrate the concepts,let us discuss the symmetry group of a square in two dimensions,i.e.we are interested in all transformations which transform the square into itself.(cf.Fig.6.1)This example will be of later use for the discussion of photonic band structures. A set g ofelements A.B,C...is called a group if the following four axioms are fulfilled:i)There exists an operation,often called multiplication,which associates every pair of elements of g with another element of g:A Eg,BE g→A·B=C,C∈g,ii)The associative law is valid:A,B,C∈g→ (A·B).C=A·(B.C)=A·B.C,iii)in the set exists an identity element: A,E∈g→A·E=E·A=A,iv)for all A∈G exists an inverse element A-1∈G with A·A-1=A-1·A=E. The symmetry group of the square consists of rotations of /2 around the z-axis and mirror operations.The normal vectors of the mirror planes are the x and y-axis,Oa and Ob.A mirror symmetry may be expressed as a twofold rotation,followed by an inversion.All the symmetry operations of the group of the square,named C4v are: CAv=[E,Cz,CAz,Cz,ICx,IC2y:IC2a,IC2b} (6.1) If the group theory package is included in a Mathematica notebook by means of the command Needs ["GroupTheory'Master'"]1,the newly defined com- mand c4v=InstallGroup["C4v"]will install the group in terms of the ro- tation matrices of the elements.The matrices are stored in the list c4v.The command c4vs=GetSymbol[c4v]will transform the elements of the group into symbolic form (cf.(6.1)).The symbols will be stored in the list c4vs. Operations on the group can be done in both representations of the group el- ements.The group multiplication is implemented in our Mathematica package by a redefinition of the infix-operator (see 6.25). Fig.6.1.Symmetry of a square. 1 Mathematica commands are marked using this particular font.6 Symmetry Properties of Electronic and Photonic Band Structures 105 6.3 Basic Concepts in Group Theory In this section we will introduce the basic concepts of group theory. We will focus on definitions which will be necessary for the following discussions. To illustrate the concepts, let us discuss the symmetry group of a square in two dimensions, i.e. we are interested in all transformations which transform the square into itself. (cf. Fig. 6.1) This example will be of later use for the discussion of photonic band structures. A set G of elements A,B,C ... is called a group if the following four axioms are fulfilled: i) There exists an operation, often called multiplication, which associates every pair of elements of G with another element of G: A ∈ G, B ∈ G → A · B = C, C ∈ G, ii) The associative law is valid: A, B, C ∈G→ (A · B)· C = A ·(B · C) = A · B · C, iii) in the set exists an identity element: A, E ∈G→ A · E = E · A = A, iv) for all A ∈ G exists an inverse element A−1 ∈ G with A · A−1 = A−1 · A = E. The symmetry group of the square consists of rotations of π/2 around the z-axis and mirror operations. The normal vectors of the mirror planes are the x and y-axis, −→ Oa and −→ Ob. A mirror symmetry may be expressed as a twofold rotation, followed by an inversion. All the symmetry operations of the group of the square, named C4v are: C4v =  E,C2z, C4z, C−1 4z ,IC2x,IC2y,IC2a,IC2b (6.1) If the group theory package is included in a Mathematica notebook by means of the command Needs["GroupTheory‘Master‘"]1, the newly defined com￾mand c4v=InstallGroup["C4v"] will install the group in terms of the ro￾tation matrices of the elements. The matrices are stored in the list c4v. The command c4vs=GetSymbol[c4v] will transform the elements of the group into symbolic form (cf.(6.1)). The symbols will be stored in the list c4vs. Operations on the group can be done in both representations of the group el￾ements. The group multiplication is implemented in our Mathematica package by a redefinition of the infix-operator ⊕ (see [6.25]). Fig. 6.1. Symmetry of a square. 1 Mathematica commands are marked using this particular font