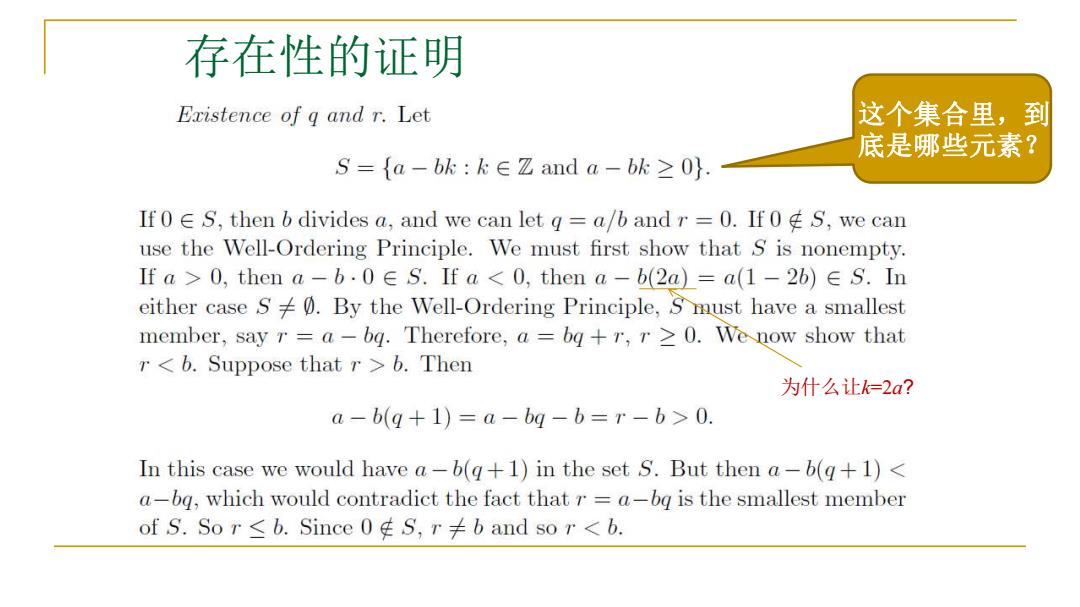
存在性的证明 Existence of q and r.Let 这个集合里，到 底是哪些元素？ S={a-bk:k∈Z and a-bk≥0}. If 0ES,then b divides a,and we can let q=a/b and r =0.If oS,we can use the Well-Ordering Principle.We must first show that S is nonempty. If a 0,then a-6.0E S.If a<0,then a-b(2a)a(1-26)ES.In either case S0.By the Well-Ordering Principle,S must have a smallest member,say r=a-bg.Therefore,a bg+r,r>0.We now show that r<b.Suppose that r>6.Then 为什么让k=2a? a-b(g+1)=a-bg-b=r-6>0. In this case we would have a-b(q+1)in the set S.But then a-b(q+1)< a-bg,which would contradict the fact that r=a-bg is the smallest member ofS.Sor≤b.Since0tS,r≠b and so r<b.存在性的证明 为什么让k=2a? 这个集合里，到 底是哪些元素？