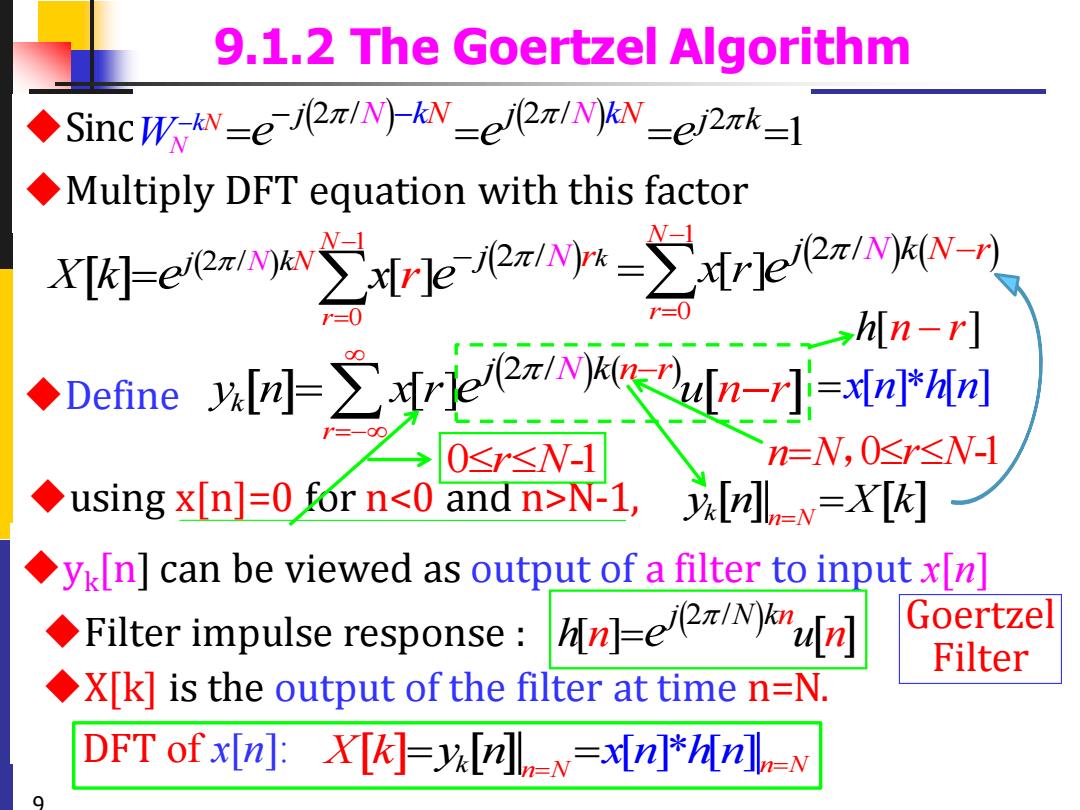
9.1.2 The Goertzel Algorithm ◆Sinc W e-eJ2rlkN=elπ/NkW=e2ak-l Multiply DFT equation with this factor X图-e2xv∑ire2aN-∑rkex/Hv- hin-r] ◆Define小-=之re2zun-r]=m*M列l 0≤≤W-1 n=N,0sr≤W-1 using x[n]=0 for n<0 and n>N-1, velnli=X[闪 yun]can be viewed as output of a filter to input x[n] Filter impulse response Hnj-erNun] Goertzel Filter X[k]is the output of the filter at time n=N. DFT of x[n]:X[k]=yalnll-N=xn]*hnll-N9 ◆yk [n] can be viewed as output of a filter to input x[n] ◆Filter impulse response : ◆X[k] is the output of the filter at time n=N. 9.1.2 The Goertzel Algorithm ◆Since ◆Multiply DFT equation with this factor (2 / 2 / ) ( ) 2 1 kN N W j j N kN N kN j k e e e − −   −  = = = =   ( ) ( ) 1 0 2 / 2 / [ ] r k N j k N N j N r X k x e er   − = − =      k n N y n X k = = ( )   2 / [ ] j N kn h u n n e  =   ( ) ( )   2 / [ ] k r j k N n r y n x r u e n r   =− − ◆Define = − ◆using x[n]=0 for n<0 and n>N-1, ( ) ( ) 1 0 2 / [ ] N r j k N N r x r e  − = − = h[ ] n r − =x n h n [ ]* [ ] 0 -1  r N n N= ，0 -1  r N Goertzel Filter DFT of x[n]: the filter is a     [ ]* [ ] X k = = yk n n N= x n h n n N=   ( ) 0 1 2 / [ ] , N n j N kn X k x n e  = − − =