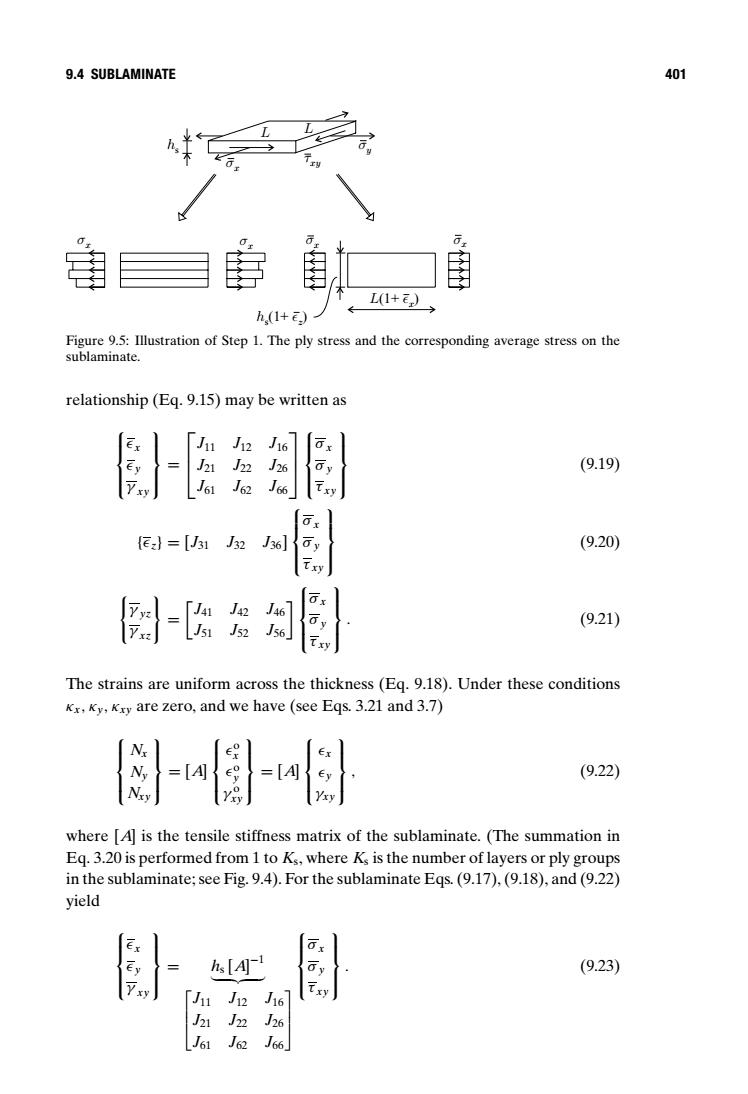
9.4 SUBLAMINATE 401 0. L(1+E) h(1+e) Figure 9.5:Illustration of Step 1.The ply stress and the corresponding average stress on the sublaminate. relationship (Eq.9.15)may be written as J12 J16 J21 J J26 (9.19) 16 J62 e}=[J31 J32 J36] (9.20) 「J4 J46 6 (9.21) J56 69 The strains are uniform across the thickness(Eq.9.18).Under these conditions Kx,Ky,Kxy are zero,and we have (see Egs.3.21 and 3.7) (9.22) where [A]is the tensile stiffness matrix of the sublaminate.(The summation in Eq.3.20 is performed from 1 to Ks,where Ks is the number of layers or ply groups in the sublaminate;see Fig.9.4).For the sublaminate Egs.(9.17),(9.18),and(9.22) yield [A (9.23) J11 J12 J16 J21 J26 J61 J62 J6小9.4 SUBLAMINATE 401 σx σx L(1+ )x hs (1+ )z σx σx hs L L σx σy τxy Figure 9.5: Illustration of Step 1. The ply stress and the corresponding average stress on the sublaminate. relationship (Eq. 9.15) may be written as    x  y γ xy    =    J11 J12 J16 J21 J22 J26 J61 J62 J66       σ x σ y τ xy    (9.19) {z} = [J31 J32 J36]    σ x σ y τ xy    (9.20) 1 γ yz γ xz6 = J41 J42 J46 J51 J52 J56!    σ x σ y τ xy    . (9.21) The strains are uniform across the thickness (Eq. 9.18). Under these conditions κx, κy, κxy are zero, and we have (see Eqs. 3.21 and 3.7)    Nx Ny Nxy    = [A]    o x o y γ o xy    = [A]    x y γxy    , (9.22) where [A] is the tensile stiffness matrix of the sublaminate. (The summation in Eq. 3.20 is performed from 1 to Ks, where Ks is the number of layers or ply groups in the sublaminate; see Fig. 9.4). For the sublaminate Eqs. (9.17), (9.18), and (9.22) yield    x  y γ xy    = hs [A] −1 % &' (      J11 J12 J16 J21 J22 J26 J61 J62 J66         σ x σ y τ xy    . (9.23)