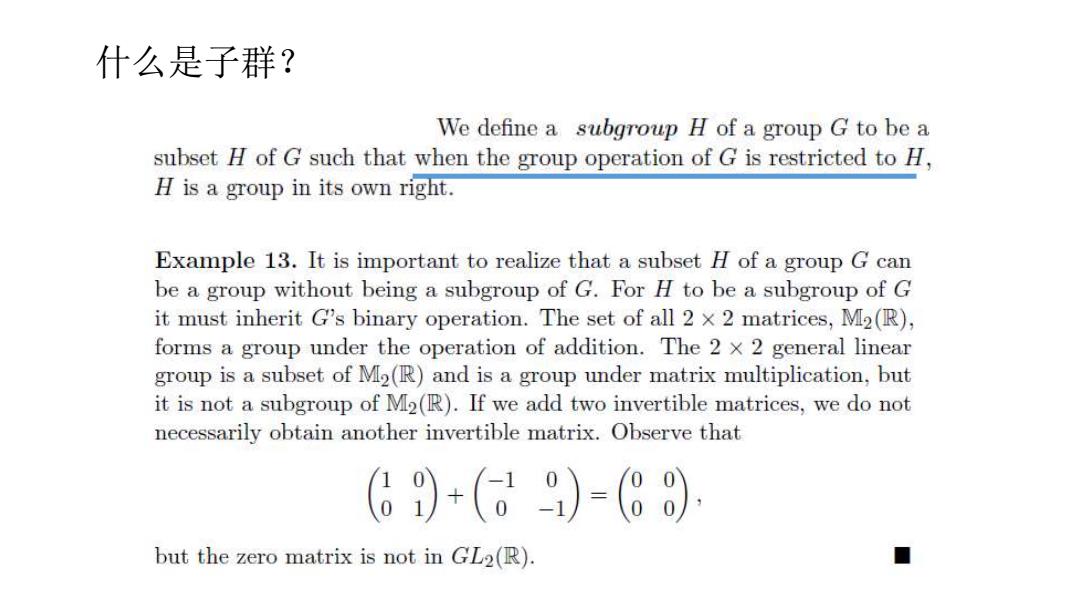
什么是子群？ We define a subgroup H of a group G to be a subset H of G such that when the group operation of G is restricted to H, H is a group in its own right. Example 13.It is important to realize that a subset H of a group G can be a group without being a subgroup of G.For H to be a subgroup of G it must inherit G's binary operation.The set of all 2 x 2 matrices,M2(R), forms a group under the operation of addition.The 2 x 2 general linear group is a subset of M2(R)and is a group under matrix multiplication,but it is not a subgroup of M2(R).If we add two invertible matrices,we do not necessarily obtain another invertible matrix.Observe that 69)+(0)=68) but the zero matrix is not in GL2(R). ■什么是子群？