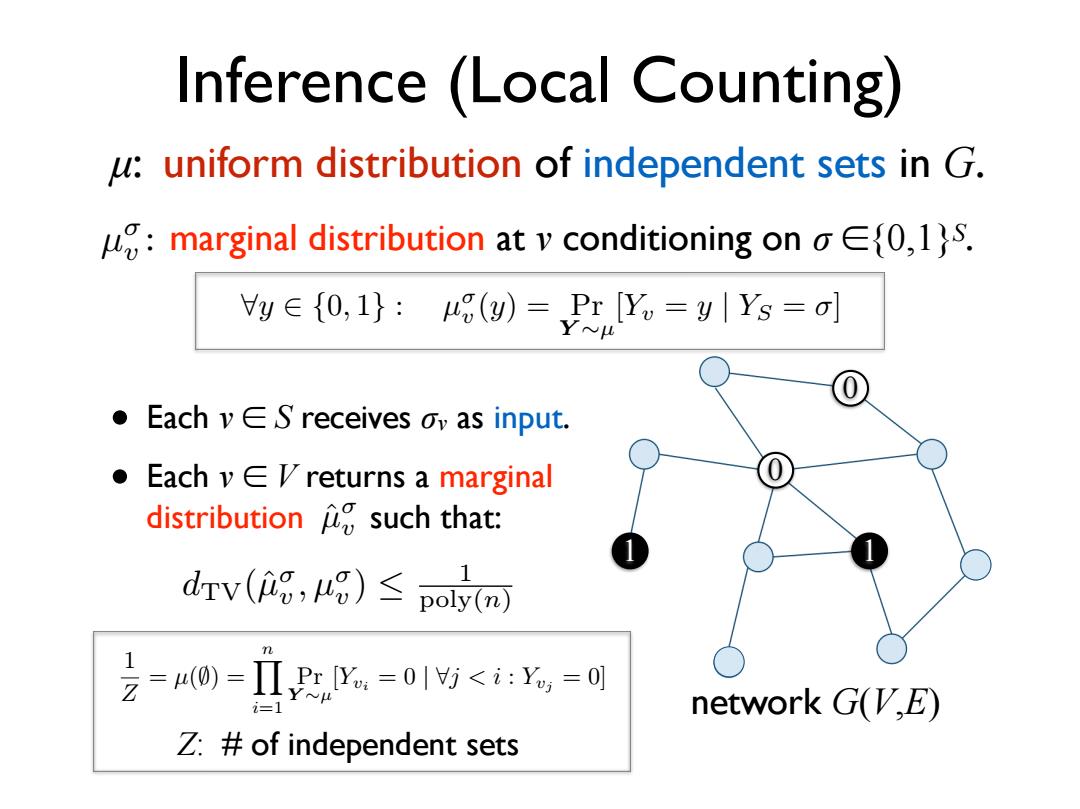
Inference (Local Counting) w:uniform distribution of independent sets in G. g:marginal distribution at v conditioning on o e0,1)5. y∈{0,1}：g(y)=Pr[Y=y|Ys=o] ●Each y∈S receives ov as input. ● Each v E D returns a marginal distributionsuch that: drv(g,hg)≤poim Z=40-ΠyX.=01<i:X,=0 i=1 network G(E) Z:of independent setsInference (Local Counting) network G(V,E) µ: uniform distribution of independent sets in G. • Each v ∈ S receives σv as input. • Each v ∈ V returns a marginal distribution such that: µ ˆ￾ v dTV(ˆµ￾ v , µ￾ v )  1 poly(n) : marginal distribution at v conditioning on σ ∈{0,1}S µ . ￾ v 0 1 1 0 8y 2 {0, 1} : µ￾ v (y) = Pr Y ⇠µ [Yv = y | YS = ￾] 1 Z = µ(;) = Y n i=1 Pr Y ⇠µ [Yvi = 0 | 8j<i : Yvj = 0] Z: # of independent sets