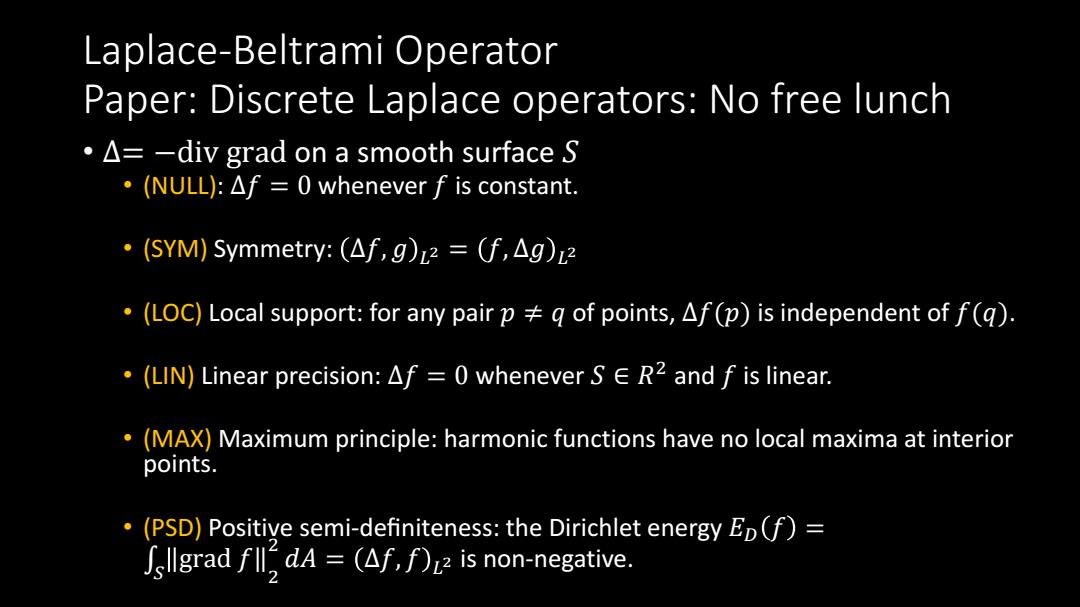
Laplace-Beltrami Operator Paper:Discrete Laplace operators:No free lunch ·△=-div grad on a smooth surface S ·(NULL):△f=0 whenever f is constant.. ·(SYM)Symmetry:(△f,g)z2=(f,△g)2 .(LOC)Local support:for any pair p q of points,Af(p)is independent of f(q) ·(LIN)Linear precision:△f=0 whenever S∈R2 and f is linear.. .(MAX)Maximum principle:harmonic functions have no local maxima at interior points. .(PSD)Positive semi-definiteness:the Dirichlet energy Ep(f)= llgrad fl dA =(Af,f)is non-negative.Laplace-Beltrami Operator Paper: Discrete Laplace operators: No free lunch • ∆= −div grad on a smooth surface 𝑆 • (NULL): Δ𝑓 = 0 whenever 𝑓 is constant. • (SYM) Symmetry: ∆𝑓, 𝑔 𝐿 2 = 𝑓, ∆𝑔 𝐿 2 • (LOC) Local support: for any pair 𝑝 ≠ 𝑞 of points, ∆𝑓(𝑝) is independent of 𝑓(𝑞). • (LIN) Linear precision: ∆𝑓 = 0 whenever 𝑆 ∈ 𝑅 2 and 𝑓 is linear. • (MAX) Maximum principle: harmonic functions have no local maxima at interior points. • (PSD) Positive semi-definiteness: the Dirichlet energy 𝐸𝐷 𝑓 = 𝑆 grad 𝑓 2 2 𝑑𝐴 = ∆𝑓, 𝑓 𝐿 2 is non-negative