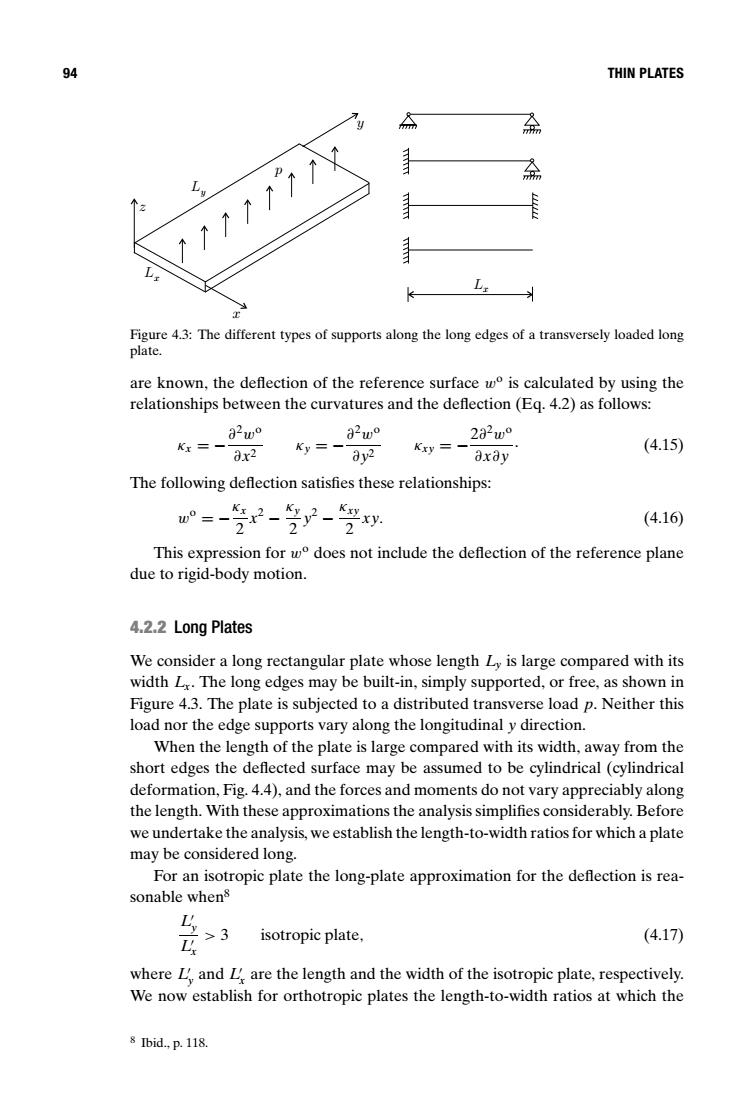
94 THIN PLATES A 品 余 Figure 4.3:The different types of supports along the long edges of a transversely loaded long plate. are known,the deflection of the reference surface wo is calculated by using the relationships between the curvatures and the deflection(Eq.4.2)as follows: 82w° 82wo 282w° Kx=一 8x2 Ky=- ay2 Kxy=一 (4.15) The following deflection satisfies these relationships: w=-受-3-受y (4.16 This expression for wo does not include the deflection of the reference plane due to rigid-body motion. 4.2.2 Long Plates We consider a long rectangular plate whose length Ly is large compared with its width L.The long edges may be built-in,simply supported,or free,as shown in Figure 4.3.The plate is subjected to a distributed transverse load p.Neither this load nor the edge supports vary along the longitudinal y direction. When the length of the plate is large compared with its width,away from the short edges the deflected surface may be assumed to be cylindrical(cylindrical deformation,Fig.4.4),and the forces and moments do not vary appreciably along the length.With these approximations the analysis simplifies considerably.Before we undertake the analysis,we establish the length-to-width ratios for which a plate may be considered long. For an isotropic plate the long-plate approximation for the deflection is rea- sonable whens isotropic plate. (4.17) where L,and L are the length and the width of the isotropic plate,respectively. We now establish for orthotropic plates the length-to-width ratios at which the 8bid,p.118.94 THIN PLATES y x Ly Lx Lx p z Figure 4.3: The different types of supports along the long edges of a transversely loaded long plate. are known, the deflection of the reference surface wo is calculated by using the relationships between the curvatures and the deflection (Eq. 4.2) as follows: κx = −∂2wo ∂x2 κy = −∂2wo ∂y2 κxy = −2∂2wo ∂x∂y . (4.15) The following deflection satisfies these relationships: wo = −κx 2 x2 − κy 2 y2 − κxy 2 xy. (4.16) This expression for wo does not include the deflection of the reference plane due to rigid-body motion. 4.2.2 Long Plates We consider a long rectangular plate whose length Ly is large compared with its width Lx. The long edges may be built-in, simply supported, or free, as shown in Figure 4.3. The plate is subjected to a distributed transverse load p. Neither this load nor the edge supports vary along the longitudinal y direction. When the length of the plate is large compared with its width, away from the short edges the deflected surface may be assumed to be cylindrical (cylindrical deformation, Fig. 4.4), and the forces and moments do not vary appreciably along the length. With these approximations the analysis simplifies considerably. Before we undertake the analysis, we establish the length-to-width ratios for which a plate may be considered long. For an isotropic plate the long-plate approximation for the deflection is rea￾sonable when8 L y L x > 3 isotropic plate, (4.17) where L y and L x are the length and the width of the isotropic plate, respectively. We now establish for orthotropic plates the length-to-width ratios at which the 8 Ibid., p. 118.