正在加载图片...
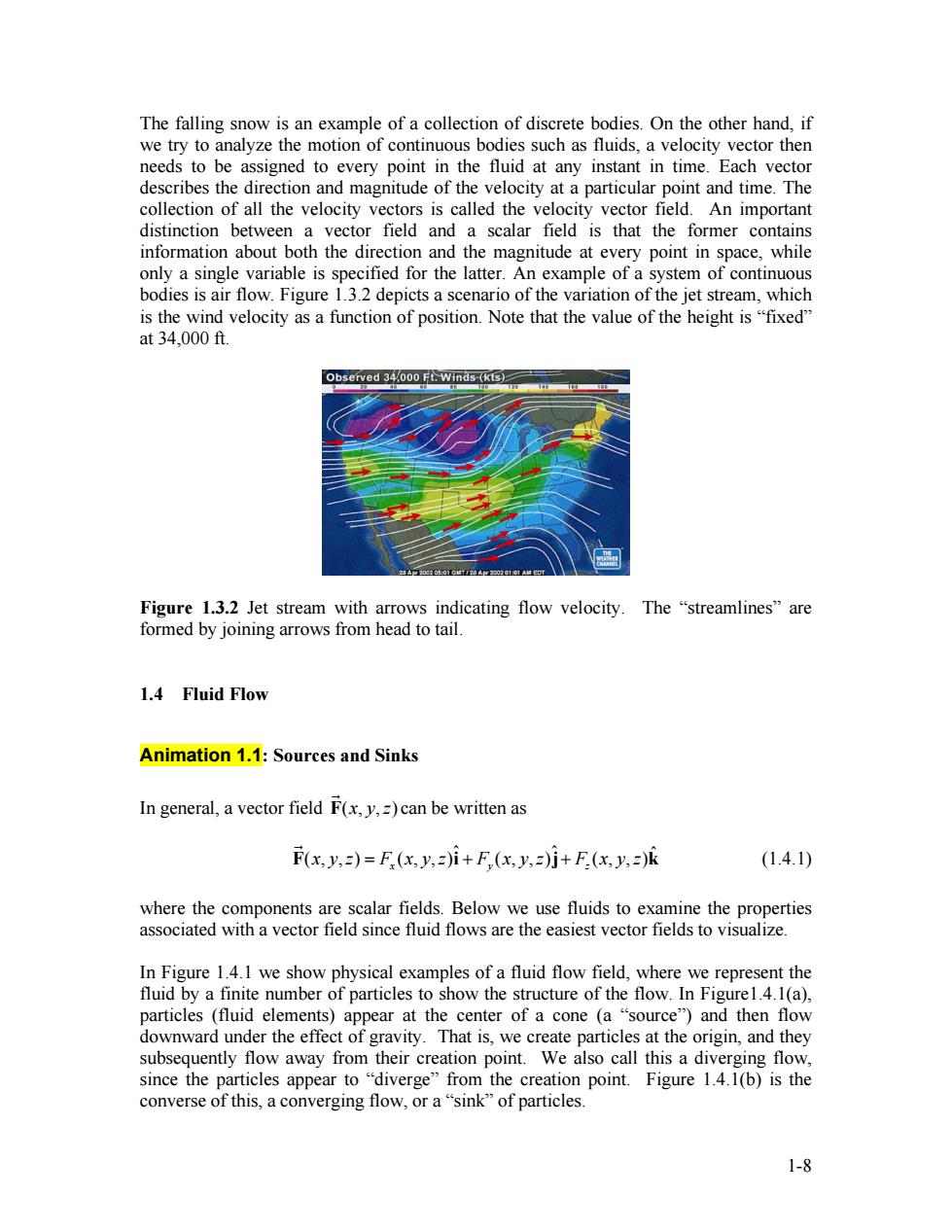
The falling snow is an example of a collection of discrete bodies.On the other hand,if we try to analyze the motion of continuous bodies such as fluids,a velocity vector then needs to be assigned to every point in the fluid at any instant in time.Each vector describes the direction and magnitude of the velocity at a particular point and time.The collection of all the velocity vectors is called the velocity vector field.An important distinction between a vector field and a scalar field is that the former contains information about both the direction and the magnitude at every point in space,while only a single variable is specified for the latter.An example of a system of continuous bodies is air flow.Figure 1.3.2 depicts a scenario of the variation of the jet stream,which is the wind velocity as a function of position.Note that the value of the height is "fixed" at34,000ft. Observed 34,000 Ft.Winds (kts) Figure 1.3.2 Jet stream with arrows indicating flow velocity.The "streamlines"are formed by joining arrows from head to tail. 1.4 Fluid Flow Animation 1.1:Sources and Sinks In general,a vector field F(x,y,)can be written as F(x.y,=)=F(x.y,=)i+F(x.y,=)j+F(x.y,2)k (1.4.1) where the components are scalar fields.Below we use fluids to examine the properties associated with a vector field since fluid flows are the easiest vector fields to visualize In Figure 1.4.1 we show physical examples of a fluid flow field,where we represent the fluid by a finite number of particles to show the structure of the flow.In Figure1.4.1(a), particles (fluid elements)appear at the center of a cone (a "source")and then flow downward under the effect of gravity.That is,we create particles at the origin,and they subsequently flow away from their creation point.We also call this a diverging flow, since the particles appear to "diverge"from the creation point.Figure 1.4.1(b)is the converse of this,a converging flow,or a "sink"of particles. 1-81-8 The falling snow is an example of a collection of discrete bodies. On the other hand, if we try to analyze the motion of continuous bodies such as fluids, a velocity vector then needs to be assigned to every point in the fluid at any instant in time. Each vector describes the direction and magnitude of the velocity at a particular point and time. The collection of all the velocity vectors is called the velocity vector field. An important distinction between a vector field and a scalar field is that the former contains information about both the direction and the magnitude at every point in space, while only a single variable is specified for the latter. An example of a system of continuous bodies is air flow. Figure 1.3.2 depicts a scenario of the variation of the jet stream, which is the wind velocity as a function of position. Note that the value of the height is “fixed” at 34,000 ft. Figure 1.3.2 Jet stream with arrows indicating flow velocity. The “streamlines” are formed by joining arrows from head to tail. 1.4 Fluid Flow Animation 1.1: Sources and Sinks In general, a vector field F(, ,) x y z G can be written as ˆ ˆ ˆ (, ,) (, ,) (, ,) (, ,) xyz F i x yz F xyz F xyz F xyz =++j k G (1.4.1) where the components are scalar fields. Below we use fluids to examine the properties associated with a vector field since fluid flows are the easiest vector fields to visualize. In Figure 1.4.1 we show physical examples of a fluid flow field, where we represent the fluid by a finite number of particles to show the structure of the flow. In Figure1.4.1(a), particles (fluid elements) appear at the center of a cone (a “source”) and then flow downward under the effect of gravity. That is, we create particles at the origin, and they subsequently flow away from their creation point. We also call this a diverging flow, since the particles appear to “diverge” from the creation point. Figure 1.4.1(b) is the converse of this, a converging flow, or a “sink” of particles