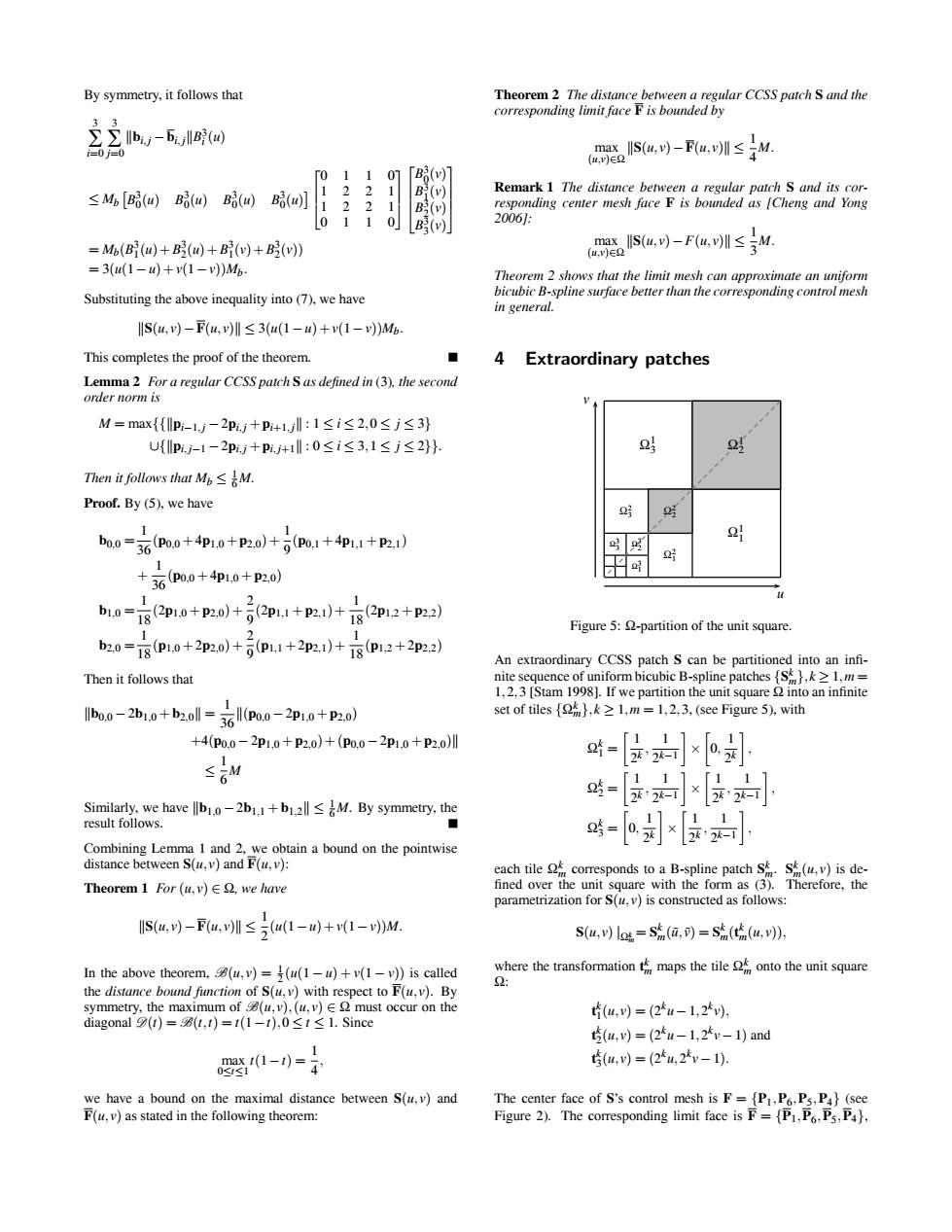
By symmetry,it follows tha hio2amarCcSsaisamh al. w到 7 二度+ mex IS(a.v]-F(o.v)sM k 4 Extraordinary patches Then at e≤表M Prof.By (5 bpo=(Ppo+4pua+P20)+(pu1+4p1.:+Pz: n一8pa+pm+号p1+p+p2+2p Then it followst 1bo0 2b1e b:ol -l(po0 2pLo p2ok +pa-2pLn+p防m+西n-2p1P2l -司 叫- s-Fas-tW- s￥a-位，可-u,) sformtiun maps the tikonto the unitsqu 明=的-1 1-小= e By symmetry, it follows that 3 ∑ i=0 3 ∑ j=0 bi, j −bi, jB3 i (u) ≤ Mb B3 0(u) B3 0(u) B3 0(u) B3 0(u) ⎡ ⎢ ⎣ 0110 1221 1221 0110 ⎤ ⎥ ⎦ ⎡ ⎢ ⎢ ⎣ B3 0(v) B3 1(v) B3 2(v) B3 3(v) ⎤ ⎥ ⎥ ⎦ = Mb(B3 1(u) +B3 2(u) +B3 1(v) +B3 2(v)) = 3(u(1−u) +v(1−v))Mb. Substituting the above inequality into (7), we have S(u, v)−F(u, v) ≤ 3(u(1−u) +v(1−v))Mb. This completes the proof of the theorem. Lemma 2 For a regular CCSS patch S as defined in (3), the second order norm is M = max{{pi−1, j −2pi, j +pi+1, j : 1 ≤ i ≤ 2,0 ≤ j ≤ 3} ∪{pi, j−1 −2pi, j +pi, j+1 : 0 ≤ i ≤ 3,1 ≤ j ≤ 2}}. Then it follows that Mb ≤ 1 6M. Proof. By (5), we have b0,0 = 1 36 (p0,0 +4p1,0 +p2,0) + 1 9 (p0,1 +4p1,1 +p2,1) + 1 36 (p0,0 +4p1,0 +p2,0) b1,0 = 1 18 (2p1,0 +p2,0) + 2 9 (2p1,1 +p2,1) + 1 18 (2p1,2 +p2,2) b2,0 = 1 18 (p1,0 +2p2,0) + 2 9 (p1,1 +2p2,1) + 1 18 (p1,2 +2p2,2) Then it follows that b0,0 −2b1,0 +b2,0 = 1 36 (p0,0 −2p1,0 +p2,0) +4(p0,0 −2p1,0 +p2,0)+(p0,0 −2p1,0 +p2,0) ≤ 1 6 M Similarly, we have b1,0 −2b1,1 +b1,2 ≤ 1 6M. By symmetry, the result follows. Combining Lemma 1 and 2, we obtain a bound on the pointwise distance between S(u, v) and F(u, v): Theorem 1 For (u, v) ∈ Ω, we have S(u, v)−F(u, v) ≤ 1 2 (u(1−u) +v(1−v))M. In the above theorem, B(u, v) = 1 2 (u(1 − u) + v(1 − v)) is called the distance bound function of S(u, v) with respect to F(u, v). By symmetry, the maximum of B(u, v),(u, v) ∈ Ω must occur on the diagonal D(t) = B(t,t) = t(1−t),0 ≤ t ≤ 1. Since max 0≤t≤1 t(1−t) = 1 4 , we have a bound on the maximal distance between S(u, v) and F(u, v) as stated in the following theorem: Theorem 2 The distance between a regular CCSS patch S and the corresponding limit face F is bounded by max (u,v)∈Ω S(u, v)−F(u, v) ≤ 1 4 M. Remark 1 The distance between a regular patch S and its cor￾responding center mesh face F is bounded as [Cheng and Yong 2006]: max (u,v)∈Ω S(u, v)−F(u, v) ≤ 1 3 M. Theorem 2 shows that the limit mesh can approximate an uniform bicubic B-spline surface better than the corresponding control mesh in general. 4 Extraordinary patches Ω1 1 Ω1 Ω 2 1 3 Ω2 1 Ω2 Ω 2 2 3 Ω3 1 Ω3 2 Ω3 3 u v Figure 5: Ω-partition of the unit square. An extraordinary CCSS patch S can be partitioned into an infi- nite sequence of uniform bicubic B-spline patches {Sk m}, k ≥ 1,m = 1,2,3 [Stam 1998]. If we partition the unit square Ω into an infinite set of tiles {Ωk m}, k ≥ 1,m = 1,2,3, (see Figure 5), with Ωk 1 =  1 2k , 1 2k−1  ×  0, 1 2k  , Ωk 2 =  1 2k , 1 2k−1  ×  1 2k , 1 2k−1  , Ωk 3 =  0, 1 2k  ×  1 2k , 1 2k−1  , each tile Ωk m corresponds to a B-spline patch Sk m. Sk m(u, v) is de- fined over the unit square with the form as (3). Therefore, the parametrization for S(u, v) is constructed as follows: S(u, v) |Ωk m= Sk m(u˜, v˜) = Sk m(t k m(u, v)), where the transformation t k m maps the tile Ωk m onto the unit square Ω: t k 1(u, v)=(2ku−1,2kv), t k 2(u, v)=(2ku−1,2kv−1) and t k 3(u, v)=(2ku,2k v−1). The center face of S’s control mesh is F = {P1,P6,P5,P4} (see Figure 2). The corresponding limit face is F = {P1,P6,P5,P4}