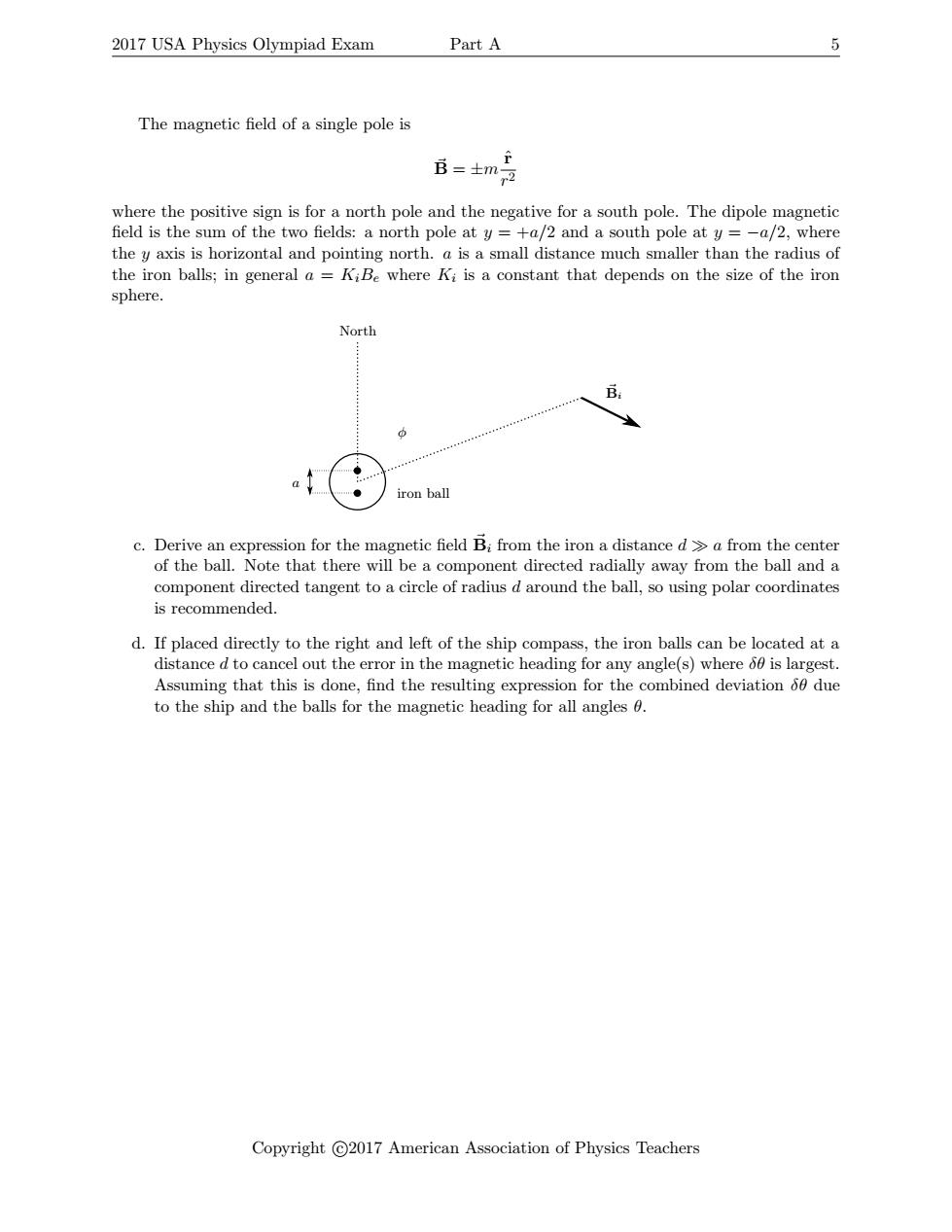
2017 USA Physics Olympiad Exam Part A 5 The magnetic field of a single pole is B=士m2 where the positive sign is for a north pole and the negative for a south pole.The dipole magnetic field is the sum of the two fields:a north pole at y=+a/2 and a south pole at y=-a/2,where the y axis is horizontal and pointing north.a is a small distance much smaller than the radius of the iron balls;in general a KiBe where Ki is a constant that depends on the size of the iron sphere. North B 4 iron ball c.Derive an expression for the magnetic field Bi from the iron a distance d>a from the center of the ball.Note that there will be a component directed radially away from the ball and a component directed tangent to a circle of radius d around the ball,so using polar coordinates is recommended. d.If placed directly to the right and left of the ship compass,the iron balls can be located at a distance d to cancel out the error in the magnetic heading for any angle(s)where 60 is largest. Assuming that this is done,find the resulting expression for the combined deviation 60 due to the ship and the balls for the magnetic heading for all angles 6. Copyright C2017 American Association of Physics Teachers2017 USA Physics Olympiad Exam Part A 5 The magnetic field of a single pole is B~ = ±m ˆr r 2 where the positive sign is for a north pole and the negative for a south pole. The dipole magnetic field is the sum of the two fields: a north pole at y = +a/2 and a south pole at y = −a/2, where the y axis is horizontal and pointing north. a is a small distance much smaller than the radius of the iron balls; in general a = KiBe where Ki is a constant that depends on the size of the iron sphere. φ North B~ i iron ball a c. Derive an expression for the magnetic field B~ i from the iron a distance d  a from the center of the ball. Note that there will be a component directed radially away from the ball and a component directed tangent to a circle of radius d around the ball, so using polar coordinates is recommended. d. If placed directly to the right and left of the ship compass, the iron balls can be located at a distance d to cancel out the error in the magnetic heading for any angle(s) where δθ is largest. Assuming that this is done, find the resulting expression for the combined deviation δθ due to the ship and the balls for the magnetic heading for all angles θ. Copyright c 2017 American Association of Physics Teachers