正在加载图片...
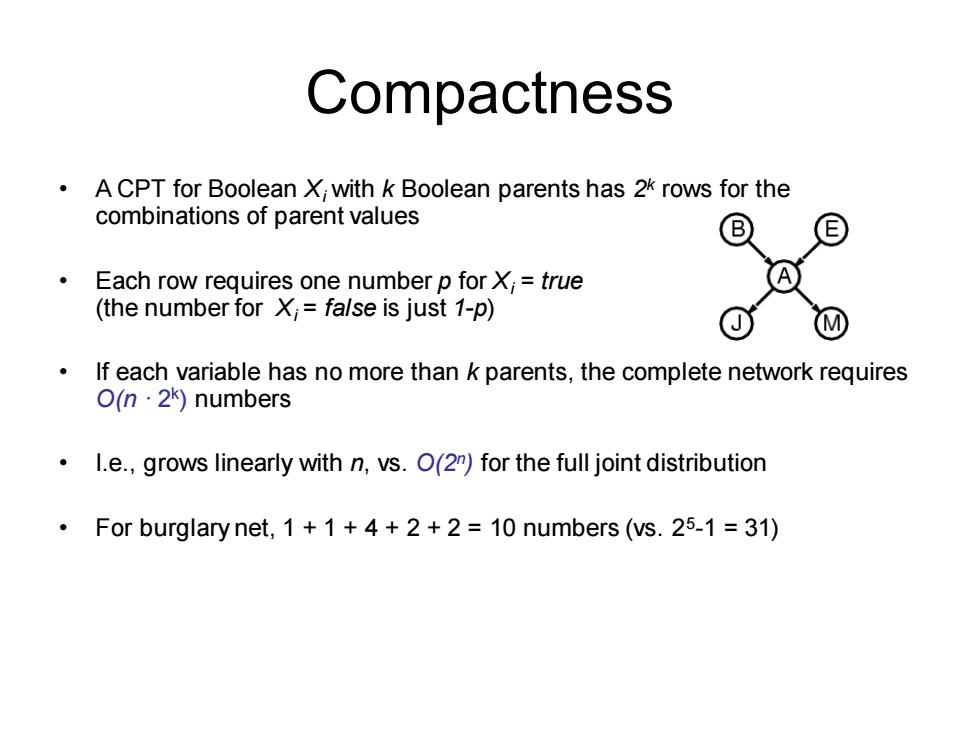
Compactness A CPT for Boolean X;with k Boolean parents has 2k rows for the combinations of parent values B Each row requires one number p for X;=true (the number for X;=false is just 1-p) M If each variable has no more than k parents,the complete network requires O(n·2 numbers .l.e.,grows linearly with n,vs.O(2)for the full joint distribution For burglary net,1+1+4+2+2=10 numbers (vs.25-1 =31) Compactness • A CPT for Boolean Xi with k Boolean parents has 2 k rows for the combinations of parent values • Each row requires one number p for Xi = true (the number for Xi = false is just 1-p) • If each variable has no more than k parents, the complete network requires O(n ·2 k ) numbers • I.e., grows linearly with n, vs. O(2n ) for the full joint distribution • For burglary net, 1 + 1 + 4 + 2 + 2 = 10 numbers (vs. 25 -1 = 31)