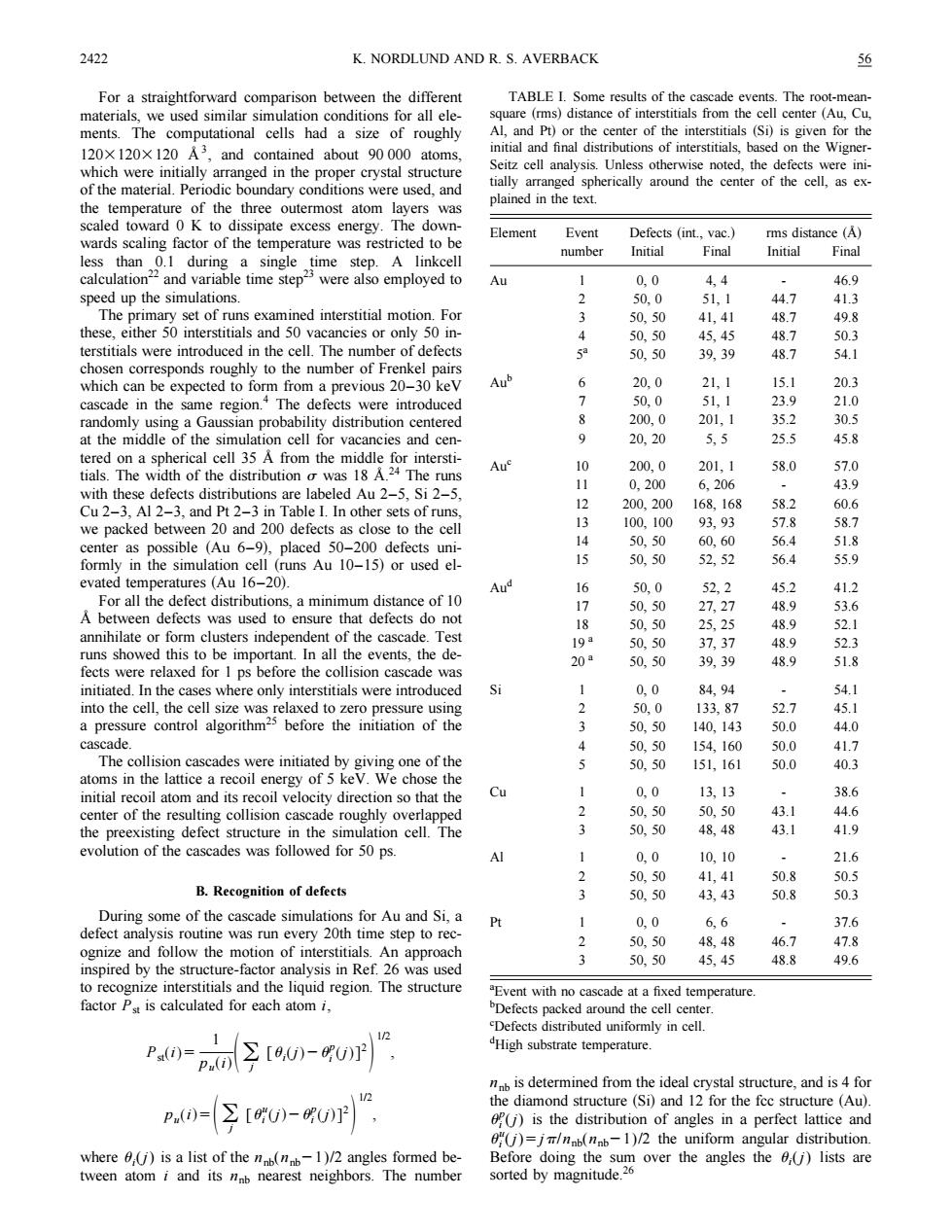
2422 K.NORDLUND AND R.S.AVERBACK 56 For a straightforward comparison between the different TABLE I.Some results of the cascade events.The root-mean- materials,we used similar simulation conditions for all ele- square (rms)distance of interstitials from the cell center (Au,Cu, ments.The computational cells had a size of roughly Al,and Pt)or the center of the interstitials (Si)is given for the 120x120X 120 A3,and contained about 90 000 atoms, initial and final distributions of interstitials,based on the Wigner- which were initially arranged in the proper crystal structure Seitz cell analysis.Unless otherwise noted,the defects were ini- of the material.Periodic boundary conditions were used,and tially arranged spherically around the center of the cell,as ex- plained in the text. the temperature of the three outermost atom layers was scaled toward 0 K to dissipate excess energy.The down- Element Event Defects (int.,vac.) rms distance (A) wards scaling factor of the temperature was restricted to be number Initial Final Initial Final less than 0.1 during a single time step.A linkcell calculation22 and variable time step23 were also employed to Au 1 0,0 4,4 46.9 speed up the simulations. 50.0 51,1 44.7 41.3 The primary set of runs examined interstitial motion.For 3 50,50 41,41 48.7 49.8 these,either 50 interstitials and 50 vacancies or only 50 in- Y 50,50 45,45 48.7 50.3 terstitials were introduced in the cell.The number of defects 5a 50,50 39,39 48.7 54.1 chosen corresponds roughly to the number of Frenkel pairs which can be expected to form from a previous 20-30 keV Au° 6 20.0 21,1 15.1 20.3 cascade in the same region.The defects were introduced > 50,0 51,1 23.9 21.0 randomly using a Gaussian probability distribution centered 8 200,0 201,1 35.2 30.5 at the middle of the simulation cell for vacancies and cen- 9 20,20 5,5 25.5 45.8 tered on a spherical cell 35 A from the middle for intersti- tials.The width of the distribution o was 18 A.24 The runs Au 10 200,0 201,1 58.0 57.0 11 0.200 6.206 43.9 with these defects distributions are labeled Au 2-5,Si 2-5, Cu 2-3,Al 2-3,and Pt 2-3 in Table I.In other sets of runs, 12 200.200 168.168 58.2 60.6 we packed between 20 and 200 defects as close to the cell 13 100,100 93,93 57.8 58.7 center as possible (Au 6-9),placed 50-200 defects uni- 14 50,50 60,60 56.4 51.8 formly in the simulation cell (runs Au 10-15)or used el- 15 50,50 52,52 56.4 55.9 evated temperatures(Au 16-20). Aud 16 50,0 52,2 45.2 41.2 For all the defect distributions,a minimum distance of 10 17 50,50 27,27 48.9 53.6 A between defects was used to ensure that defects do not 18 50,50 25,25 48.9 52.1 annihilate or form clusters independent of the cascade.Test 19a 50,50 37,37 48.9 52.3 runs showed this to be important.In all the events,the de- 20a 39,39 48.9 51.8 fects were relaxed for I ps before the collision cascade was 50,50 initiated.In the cases where only interstitials were introduced Si 0,0 84,94 54.1 into the cell,the cell size was relaxed to zero pressure using 2 50,0 133.87 52.7 45.1 a pressure control algorithm25 before the initiation of the 3 50,50 140,143 50.0 44.0 cascade. 50,50 154.160 50.0 41.7 The collision cascades were initiated by giving one of the 5 50.50 151,161 50.0 40.3 atoms in the lattice a recoil energy of 5 keV.We chose the initial recoil atom and its recoil velocity direction so that the Cu 0.0 13.13 38.6 center of the resulting collision cascade roughly overlapped 50,50 50,50 43.1 44.6 the preexisting defect structure in the simulation cell.The 3 50,50 48,48 43.1 41.9 evolution of the cascades was followed for 50 ps. 1 0,0 10,10 21.6 50,50 41,41 50.8 50.5 B.Recognition of defects 2 50,50 43,43 50.8 50.3 During some of the cascade simulations for Au and Si,a Pt 1 0,0 6,6 37.6 defect analysis routine was run every 20th time step to rec- 2 50,50 48.48 46.7 47.8 ognize and follow the motion of interstitials.An approach 3 45.45 48.8 49.6 inspired by the structure-factor analysis in Ref.26 was used 50,50 to recognize interstitials and the liquid region.The structure Event with no cascade at a fixed temperature factor P is calculated for each atom i. bDefects packed around the cell center. Defects distributed uniformly in cell. P()p Σ[,)-U2 High substrate temperature. nnb is determined from the ideal crystal structure,and is 4 for P.0-∑[U)-UP, the diamond structure(Si)and 12 for the fcc structure (Au). p()is the distribution of angles in a perfect lattice and ()=jm/nnb(nnb-1)/2 the uniform angular distribution. where ()is a list of the nb(n-1)/2 angles formed be- Before doing the sum over the angles the (j)lists are tween atom i and its nnb nearest neighbors.The number sorted by magnitude.26For a straightforward comparison between the different materials, we used similar simulation conditions for all ele￾ments. The computational cells had a size of roughly 12031203120 Å3, and contained about 90 000 atoms, which were initially arranged in the proper crystal structure of the material. Periodic boundary conditions were used, and the temperature of the three outermost atom layers was scaled toward 0 K to dissipate excess energy. The down￾wards scaling factor of the temperature was restricted to be less than 0.1 during a single time step. A linkcell calculation22 and variable time step23 were also employed to speed up the simulations. The primary set of runs examined interstitial motion. For these, either 50 interstitials and 50 vacancies or only 50 in￾terstitials were introduced in the cell. The number of defects chosen corresponds roughly to the number of Frenkel pairs which can be expected to form from a previous 20–30 keV cascade in the same region.4 The defects were introduced randomly using a Gaussian probability distribution centered at the middle of the simulation cell for vacancies and cen￾tered on a spherical cell 35 Å from the middle for intersti￾tials. The width of the distribution s was 18 Å.24 The runs with these defects distributions are labeled Au 2–5, Si 2–5, Cu 2–3, Al 2–3, and Pt 2–3 in Table I. In other sets of runs, we packed between 20 and 200 defects as close to the cell center as possible ~Au 6–9!, placed 50–200 defects uni￾formly in the simulation cell ~runs Au 10–15! or used el￾evated temperatures ~Au 16–20!. For all the defect distributions, a minimum distance of 10 Å between defects was used to ensure that defects do not annihilate or form clusters independent of the cascade. Test runs showed this to be important. In all the events, the de￾fects were relaxed for 1 ps before the collision cascade was initiated. In the cases where only interstitials were introduced into the cell, the cell size was relaxed to zero pressure using a pressure control algorithm25 before the initiation of the cascade. The collision cascades were initiated by giving one of the atoms in the lattice a recoil energy of 5 keV. We chose the initial recoil atom and its recoil velocity direction so that the center of the resulting collision cascade roughly overlapped the preexisting defect structure in the simulation cell. The evolution of the cascades was followed for 50 ps. B. Recognition of defects During some of the cascade simulations for Au and Si, a defect analysis routine was run every 20th time step to rec￾ognize and follow the motion of interstitials. An approach inspired by the structure-factor analysis in Ref. 26 was used to recognize interstitials and the liquid region. The structure factor Pst is calculated for each atom i, Pst~i!5 1 pu~i!S ( j @u i~j!2u i p ~j!# 2 D 1/2 , pu~i!5S ( j @u i u ~j!2u i p ~j!# 2 D 1/2 , where u i(j) is a list of the nnb(nnb21)/2 angles formed be￾tween atom i and its nnb nearest neighbors. The number nnb is determined from the ideal crystal structure, and is 4 for the diamond structure ~Si! and 12 for the fcc structure ~Au!. u i p (j) is the distribution of angles in a perfect lattice and u i u (j)5 jp/nnb(nnb21)/2 the uniform angular distribution. Before doing the sum over the angles the u i(j) lists are sorted by magnitude.26 TABLE I. Some results of the cascade events. The root-mean￾square ~rms! distance of interstitials from the cell center ~Au, Cu, Al, and Pt! or the center of the interstitials ~Si! is given for the initial and final distributions of interstitials, based on the Wigner￾Seitz cell analysis. Unless otherwise noted, the defects were ini￾tially arranged spherically around the center of the cell, as ex￾plained in the text. Element Event Defects ~int., vac.! rms distance ~Å! number Initial Final Initial Final Au 1 0, 0 4, 4 - 46.9 2 50, 0 51, 1 44.7 41.3 3 50, 50 41, 41 48.7 49.8 4 50, 50 45, 45 48.7 50.3 5a 50, 50 39, 39 48.7 54.1 Aub 6 20, 0 21, 1 15.1 20.3 7 50, 0 51, 1 23.9 21.0 8 200, 0 201, 1 35.2 30.5 9 20, 20 5, 5 25.5 45.8 Auc 10 200, 0 201, 1 58.0 57.0 11 0, 200 6, 206 - 43.9 12 200, 200 168, 168 58.2 60.6 13 100, 100 93, 93 57.8 58.7 14 50, 50 60, 60 56.4 51.8 15 50, 50 52, 52 56.4 55.9 Aud 16 50, 0 52, 2 45.2 41.2 17 50, 50 27, 27 48.9 53.6 18 50, 50 25, 25 48.9 52.1 19 a 50, 50 37, 37 48.9 52.3 20 a 50, 50 39, 39 48.9 51.8 Si 1 0, 0 84, 94 - 54.1 2 50, 0 133, 87 52.7 45.1 3 50, 50 140, 143 50.0 44.0 4 50, 50 154, 160 50.0 41.7 5 50, 50 151, 161 50.0 40.3 Cu 1 0, 0 13, 13 - 38.6 2 50, 50 50, 50 43.1 44.6 3 50, 50 48, 48 43.1 41.9 Al 1 0, 0 10, 10 - 21.6 2 50, 50 41, 41 50.8 50.5 3 50, 50 43, 43 50.8 50.3 Pt 1 0, 0 6, 6 - 37.6 2 50, 50 48, 48 46.7 47.8 3 50, 50 45, 45 48.8 49.6 a Event with no cascade at a fixed temperature. b Defects packed around the cell center. c Defects distributed uniformly in cell. d High substrate temperature. 2422 K. NORDLUND AND R. S. AVERBACK 56