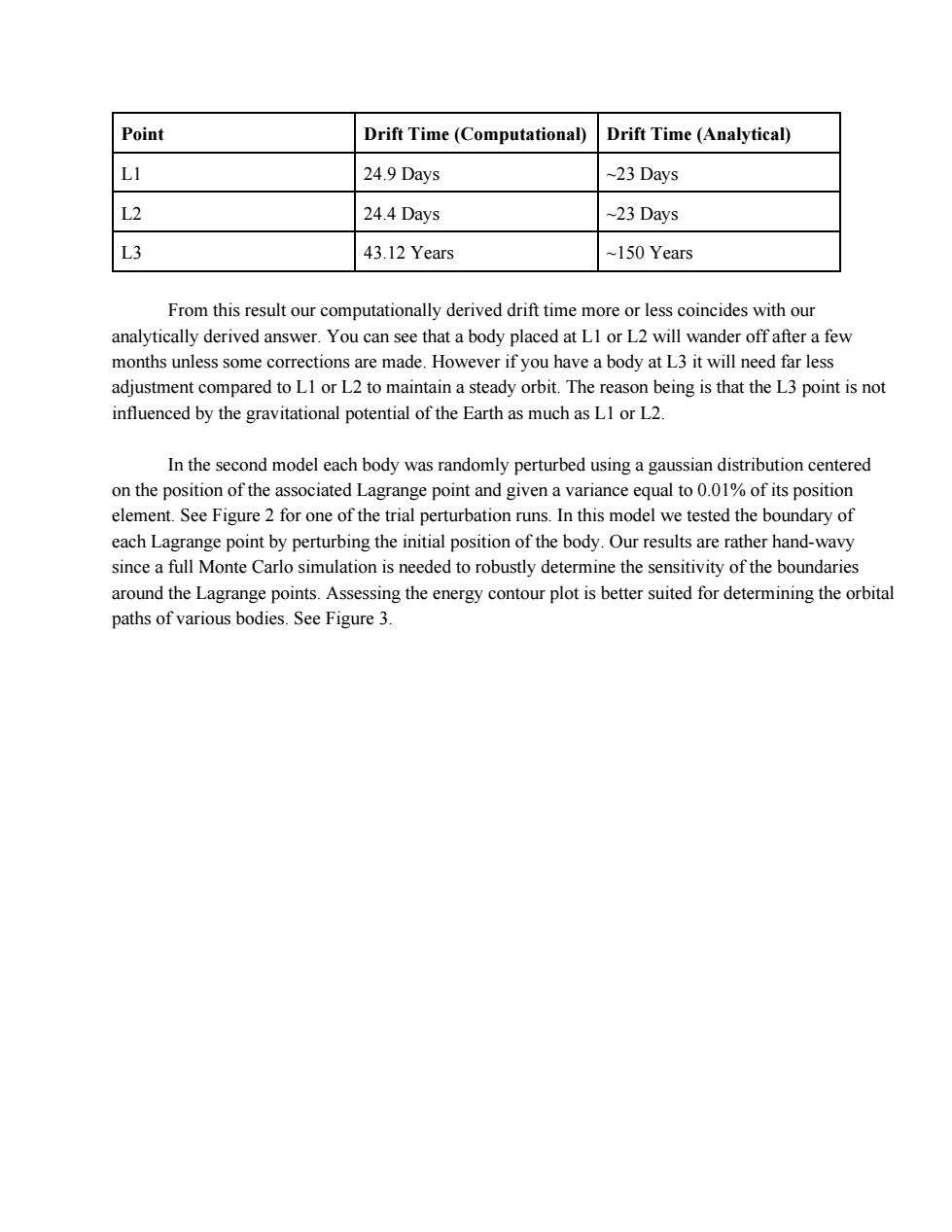
Point Drift Time (Computational) Drift Time (Analytical) LI 24.9 Days -23 Days L2 24.4 Days ~23 Days L3 43.12 Years ~150 Years From this result our computationally derived drift time more or less coincides with our analytically derived answer.You can see that a body placed at LI or L2 will wander off after a few months unless some corrections are made.However if you have a body at L3 it will need far less adjustment compared to LI or L2 to maintain a steady orbit.The reason being is that the L3 point is not influenced by the gravitational potential of the Earth as much as LI or L2. In the second model each body was randomly perturbed using a gaussian distribution centered on the position of the associated Lagrange point and given a variance equal to 0.01%of its position element.See Figure 2 for one of the trial perturbation runs.In this model we tested the boundary of each Lagrange point by perturbing the initial position of the body.Our results are rather hand-wavy since a full Monte Carlo simulation is needed to robustly determine the sensitivity of the boundaries around the Lagrange points.Assessing the energy contour plot is better suited for determining the orbital paths of various bodies.See Figure 3.Point Drift Time (Computational) Drift Time (Analytical) L1 24.9 Days ~23 Days L2 24.4 Days ~23 Days L3 43.12 Years ~150 Years From this result our computationally derived drift time more or less coincides with our analytically derived answer. You can see that a body placed at L1 or L2 will wander off after a few months unless some corrections are made. However if you have a body at L3 it will need far less adjustment compared to L1 or L2 to maintain a steady orbit. The reason being is that the L3 point is not influenced by the gravitational potential of the Earth as much as L1 or L2. In the second model each body was randomly perturbed using a gaussian distribution centered on the position of the associated Lagrange point and given a variance equal to 0.01% of its position element. See Figure 2 for one of the trial perturbation runs. In this model we tested the boundary of each Lagrange point by perturbing the initial position of the body. Our results are rather hand­wavy since a full Monte Carlo simulation is needed to robustly determine the sensitivity of the boundaries around the Lagrange points. Assessing the energy contour plot is better suited for determining the orbital paths of various bodies. See Figure 3