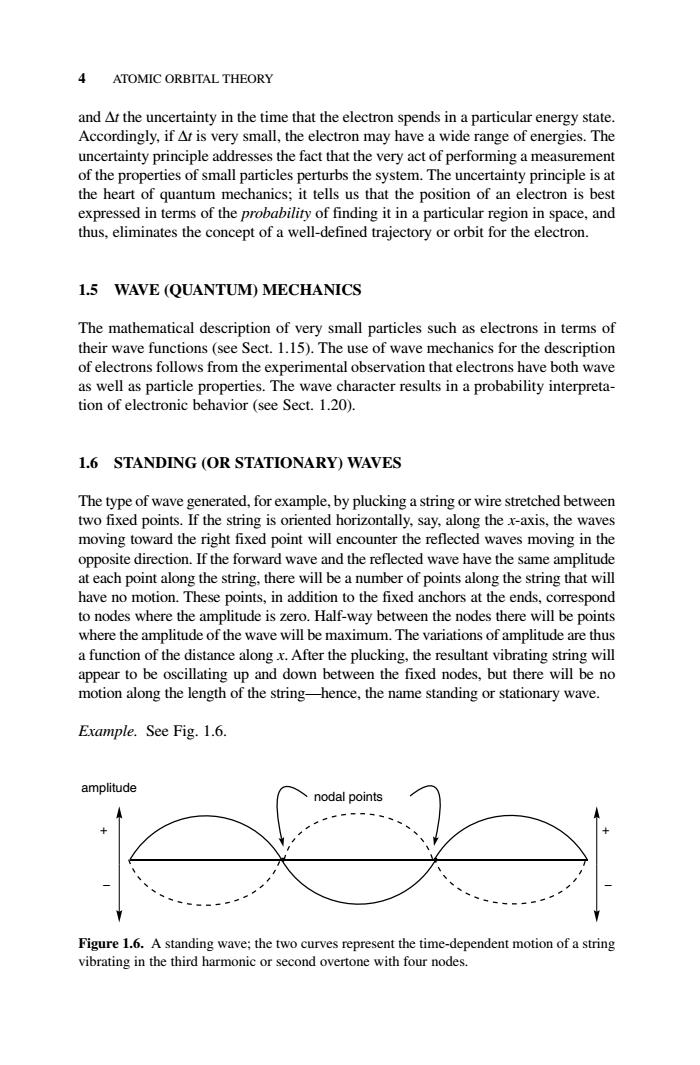
4 ATOMIC ORBITAL THEORY and At the uncertainty in the time that the electron spends in a particular energy state. Accordingly,if At is very small,the electron may have a wide range of energies.The uncertainty principle addresses the fact that the very act of performing a measurement of the properties of small particles perturbs the system.The uncertainty principle is at the heart of quantum mechanics;it tells us that the position of an electron is best essed in t ms of the probabiliry of finding it in a particular space,and .eliminates conceptfe-defmed trajectory or orbit forecle the 1.5 WAVE (QUANTUM)MECHANICS The mathematical description of very small particles such as electrons in terms of their wave functions(see Sect.1.15).The use of wave mechanics for the description of electrons follows from the experimental observation that electrons have both character results in a probability interpreta 1.6 STANDING (OR STATIONARY)WAVES The type of wave generated,for example,by plucking a string or wire stretched between two fixed points.If the string is oriented horizontally,say,along the x-axis,the waves moving toward the right fixed point will encounter the reflected waves moving in the opposite ion.If the forw d wave d the reflected w e have the ame amp at each point along the string.there will be a number of points along the string that wil have no motion.These points,in addition to the fixed anchors at the ends,correspond to nodes where the amplitude is zero.Half-way between the nodes there will be points where the amplitude of the wave will be maximum.The variations of amplitude are thus a function of the distance along x.After the plucking.the resultant vibrating string will e the m or e ne。neo Example.See Fig.1.6 amplitude nodal points Figure 1.6.A standing wave;the two curves represent the time-dependent motion of a string vibrating in the third harmonic or second overtone with four nodes. and ∆t the uncertainty in the time that the electron spends in a particular energy state. Accordingly, if ∆t is very small, the electron may have a wide range of energies. The uncertainty principle addresses the fact that the very act of performing a measurement of the properties of small particles perturbs the system. The uncertainty principle is at the heart of quantum mechanics; it tells us that the position of an electron is best expressed in terms of the probability of finding it in a particular region in space, and thus, eliminates the concept of a well-defined trajectory or orbit for the electron. 1.5 WAVE (QUANTUM) MECHANICS The mathematical description of very small particles such as electrons in terms of their wave functions (see Sect. 1.15). The use of wave mechanics for the description of electrons follows from the experimental observation that electrons have both wave as well as particle properties. The wave character results in a probability interpreta￾tion of electronic behavior (see Sect. 1.20). 1.6 STANDING (OR STATIONARY) WAVES The type of wave generated, for example, by plucking a string or wire stretched between two fixed points. If the string is oriented horizontally, say, along the x-axis, the waves moving toward the right fixed point will encounter the reflected waves moving in the opposite direction. If the forward wave and the reflected wave have the same amplitude at each point along the string, there will be a number of points along the string that will have no motion. These points, in addition to the fixed anchors at the ends, correspond to nodes where the amplitude is zero. Half-way between the nodes there will be points where the amplitude of the wave will be maximum. The variations of amplitude are thus a function of the distance along x. After the plucking, the resultant vibrating string will appear to be oscillating up and down between the fixed nodes, but there will be no motion along the length of the string—hence, the name standing or stationary wave. Example. See Fig. 1.6. 4 ATOMIC ORBITAL THEORY nodal points + − + − amplitude Figure 1.6. A standing wave; the two curves represent the time-dependent motion of a string vibrating in the third harmonic or second overtone with four nodes. c01.qxd 5/17/2005 5:12 PM Page 4