正在加载图片...
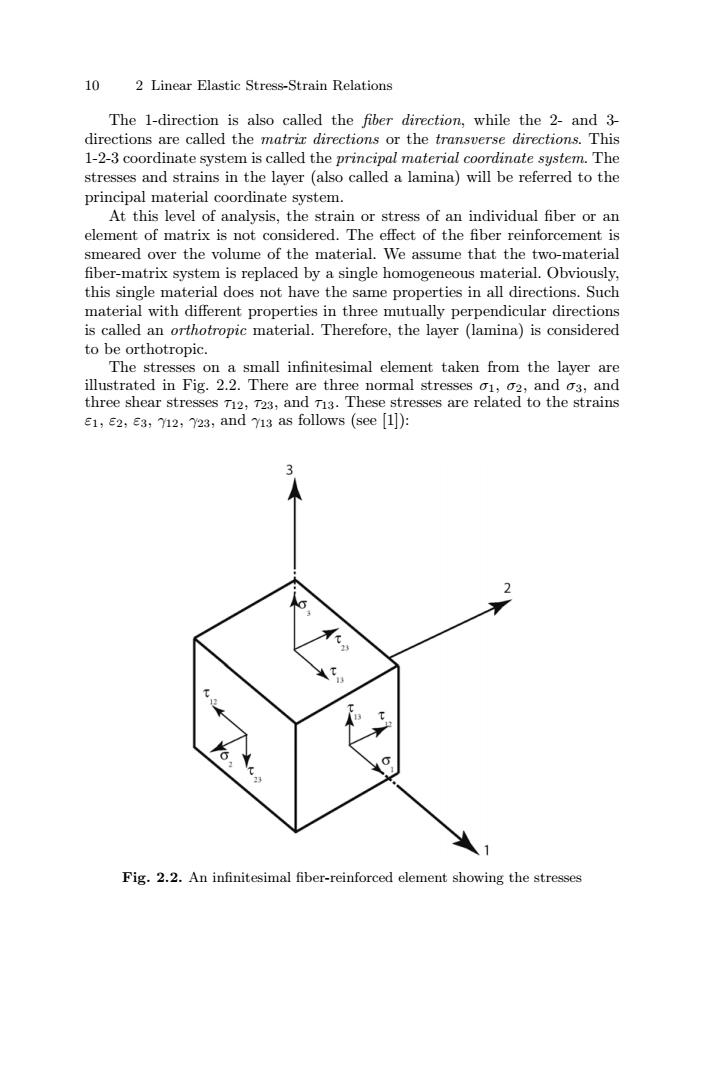
10 2 Linear Elastic Stress-Strain Relations The 1-direction is also called the fiber direction,while the 2-and 3- directions are called the matrix directions or the transverse directions.This 1-2-3 coordinate system is called the principal material coordinate system.The stresses and strains in the layer (also called a lamina)will be referred to the principal material coordinate system. At this level of analysis,the strain or stress of an individual fiber or an element of matrix is not considered.The effect of the fiber reinforcement is smeared over the volume of the material.We assume that the two-material fiber-matrix system is replaced by a single homogeneous material.Obviously, this single material does not have the same properties in all directions.Such material with different properties in three mutually perpendicular directions is called an orthotropic material.Therefore,the layer (lamina)is considered to be orthotropic. The stresses on a small infinitesimal element taken from the layer are illustrated in Fig.2.2.There are three normal stresses o1,02,and 03,and three shear stresses T12,T23,and 713.These stresses are related to the strains 61,52,E3,12,723,and 13 as follows (see [1]): 2 23 Fig.2.2.An infinitesimal fiber-reinforced element showing the stresses10 2 Linear Elastic Stress-Strain Relations The 1-direction is also called the fiber direction, while the 2- and 3- directions are called the matrix directions or the transverse directions. This 1-2-3 coordinate system is called the principal material coordinate system. The stresses and strains in the layer (also called a lamina) will be referred to the principal material coordinate system. At this level of analysis, the strain or stress of an individual fiber or an element of matrix is not considered. The effect of the fiber reinforcement is smeared over the volume of the material. We assume that the two-material fiber-matrix system is replaced by a single homogeneous material. Obviously, this single material does not have the same properties in all directions. Such material with different properties in three mutually perpendicular directions is called an orthotropic material. Therefore, the layer (lamina) is considered to be orthotropic. The stresses on a small infinitesimal element taken from the layer are illustrated in Fig. 2.2. There are three normal stresses σ1, σ2, and σ3, and three shear stresses τ12, τ23, and τ13. These stresses are related to the strains ε1, ε2, ε3, γ12, γ23, and γ13 as follows (see [1]): Fig. 2.2. An infinitesimal fiber-reinforced element showing the stresses