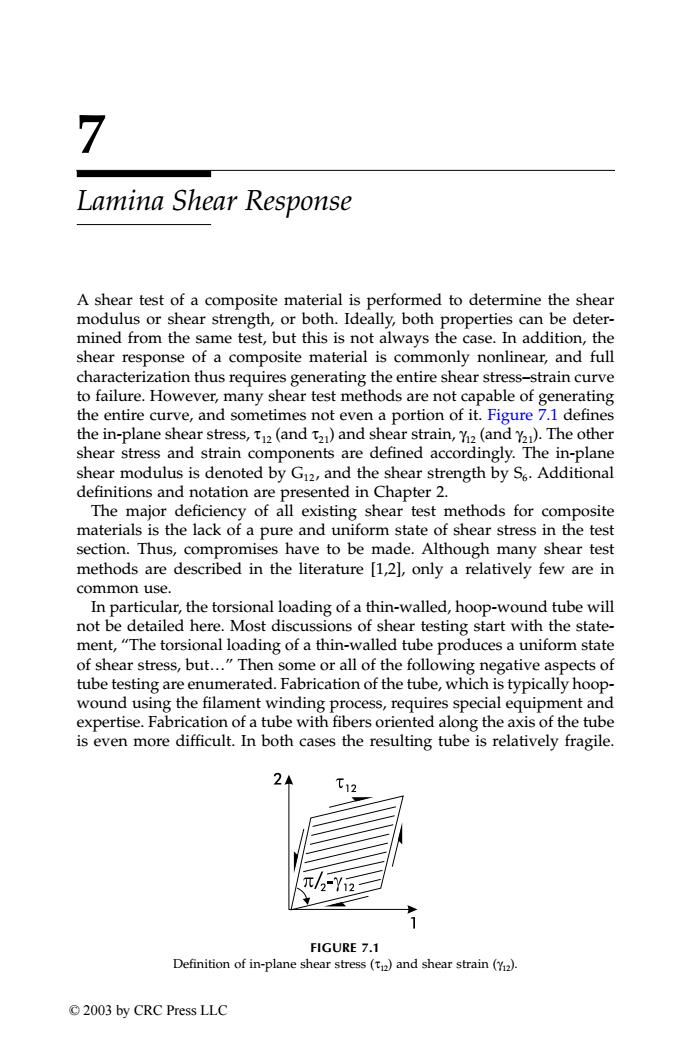
Lamina Shear Response A shear test of a composite material is performed to determine the shear modulus or shear strength,or both.Ideally,both properties can be deter- mined from the same test,but this is not always the case.In addition,the shear response of a composite material is commonly nonlinear,and full characterization thus requires generating the entire shear stress-strain curve to failure.However,many shear test methods are not capable of generating the entire curve,and sometimes not even a portion of it.Figure 7.1 defines the in-plane shear stress,t(and ta)and shear strain,2(and Y1).The other shear stress and strain components are defined accordingly.The in-plane shear modulus is denoted by G2,and the shear strength by S.Additional definitions and notation are presented in Chapter 2. The major deficiency of all existing shear test methods for composite materials is the lack of a pure and uniform state of shear stress in the test section.Thus,compromises have to be made.Although many shear test methods are described in the literature [1,2],only a relatively few are in common use. In particular,the torsional loading of a thin-walled,hoop-wound tube will not be detailed here.Most discussions of shear testing start with the state- ment,"The torsional loading of a thin-walled tube produces a uniform state of shear stress,but..."Then some or all of the following negative aspects of tube testing are enumerated.Fabrication of the tube,which is typically hoop- wound using the filament winding process,requires special equipment and expertise.Fabrication of a tube with fibers oriented along the axis of the tube is even more difficult.In both cases the resulting tube is relatively fragile. 2 /2Y12 FIGURE 7.1 Definition of in-plane shear stress()and shear strain(Y2). ©2003 by CRC Press LLC7 Lamina Shear Response A shear test of a composite material is performed to determine the shear modulus or shear strength, or both. Ideally, both properties can be deter￾mined from the same test, but this is not always the case. In addition, the shear response of a composite material is commonly nonlinear, and full characterization thus requires generating the entire shear stress–strain curve to failure. However, many shear test methods are not capable of generating the entire curve, and sometimes not even a portion of it. Figure 7.1 defines the in-plane shear stress, τ12 (and τ21) and shear strain, γ12 (and γ21). The other shear stress and strain components are defined accordingly. The in-plane shear modulus is denoted by G12 , and the shear strength by S6. Additional definitions and notation are presented in Chapter 2. The major deficiency of all existing shear test methods for composite materials is the lack of a pure and uniform state of shear stress in the test section. Thus, compromises have to be made. Although many shear test methods are described in the literature [1,2], only a relatively few are in common use. In particular, the torsional loading of a thin-walled, hoop-wound tube will not be detailed here. Most discussions of shear testing start with the state￾ment, “The torsional loading of a thin-walled tube produces a uniform state of shear stress, but…” Then some or all of the following negative aspects of tube testing are enumerated. Fabrication of the tube, which is typically hoop￾wound using the filament winding process, requires special equipment and expertise. Fabrication of a tube with fibers oriented along the axis of the tube is even more difficult. In both cases the resulting tube is relatively fragile. FIGURE 7.1 Definition of in-plane shear stress (τ12) and shear strain (γ12). TX001_ch07_Frame Page 105 Saturday, September 21, 2002 4:58 AM © 2003 by CRC Press LLC