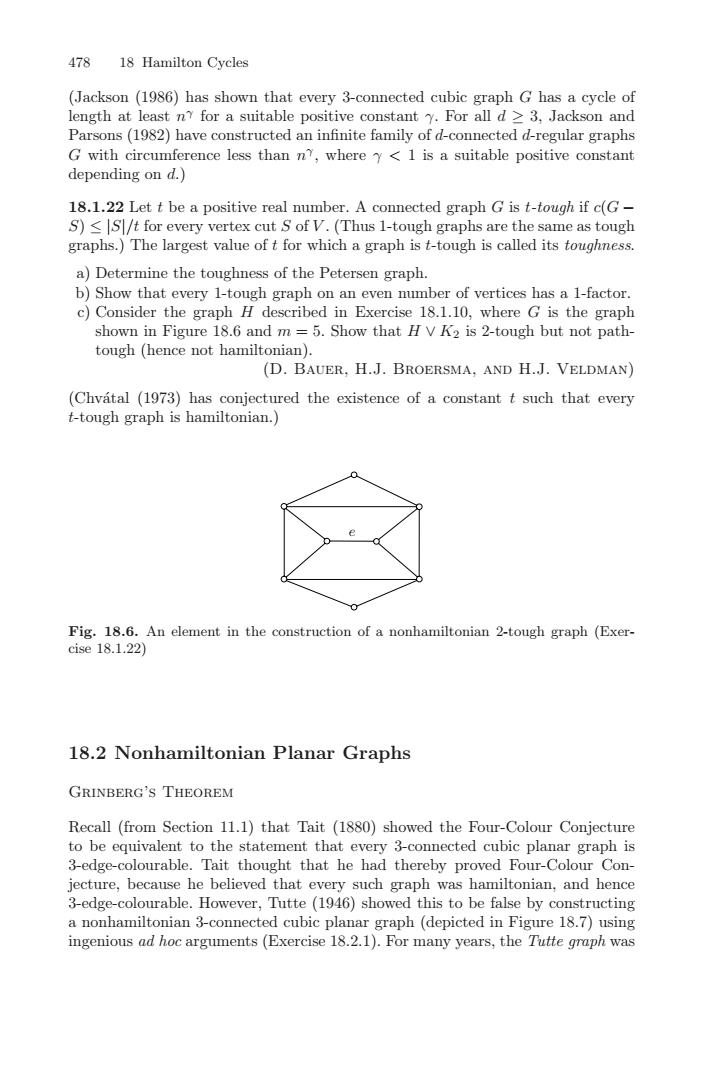
47818 Hamilton Cycles(Jackson (1986) has shown that every 3-connected cubic graph G has a cycle ofength atleast n?for asuitablepositive constant For all d >3.Jackson andParsons (1982)haveconstructed aninfinite familyofd-connectedd-regulargraphsG with circumference less than n?, where < 1 is a suitable positive constantdepending on d.)18.1.22 Let t be a positive real number. A connected graph G is t-tough if c(G -S) ≤ JS/t for every vertex cut S of V.(Thus 1-tough graphs are the same as toughgraphs.) The largest value of t for which a graph is t-tough is called its toughness.a) Determine the toughness of the Petersen graph.b) Show that every 1-tough graph on an even number of vertices has a 1-factor.) Consider the graph H described in Exercise 18.1.10, where G is the graphshown in Figure 18.6 and m = 5. Show that H v K2 is 2-tough but not path-tough (hence not hamiltonian)(D. BAUER, H.J. BROERSMA, AND H.J. VELDMAN)(Chvatal (1973) hased the existence of a constant t such that everycont-tough graph is hamiltonian.)Fig. 18.6. An element in the construction of a nonhamiltonian 2-tough graph (Exer-cise 18.1.22)18.2 Nonhamiltonian Planar GraphsGRINBERG'S THEOREMRecall (from Section 11.1) that Tait (1880) showed the Four-Colour Conjectureto be equivalent to the statement that every 3-connected cubic planar graph is3-edge-colourable, Tait thought that he had thereby proved Four-Colour Con-jecture, because he believed that every such graph was hamiltonian, and hence3-edge-colourable.However,Tutte(1946)showed this tobefalseby constructinga nonhamiltonian 3-connected cubic planar graph (depicted in Figure 18.7) usingingenious ad hoc arguments (Exercise 18.2.1). For many years, the Tutte graph was478 18 Hamilton Cycles (Jackson (1986) has shown that every 3-connected cubic graph G has a cycle of length at least nγ for a suitable positive constant γ. For all d ≥ 3, Jackson and Parsons (1982) have constructed an infinite family of d-connected d-regular graphs G with circumference less than nγ, where γ < 1 is a suitable positive constant depending on d.) 18.1.22 Let t be a positive real number. A connected graph G is t-tough if c(G − S) ≤ |S|/t for every vertex cut S of V . (Thus 1-tough graphs are the same as tough graphs.) The largest value of t for which a graph is t-tough is called its toughness. a) Determine the toughness of the Petersen graph. b) Show that every 1-tough graph on an even number of vertices has a 1-factor. c) Consider the graph H described in Exercise 18.1.10, where G is the graph shown in Figure 18.6 and m = 5. Show that H ∨ K2 is 2-tough but not path￾tough (hence not hamiltonian). (D. Bauer, H.J. Broersma, and H.J. Veldman) (Chv´atal (1973) has conjectured the existence of a constant t such that every t-tough graph is hamiltonian.) e Fig. 18.6. An element in the construction of a nonhamiltonian 2-tough graph (Exer￾cise 18.1.22) 18.2 Nonhamiltonian Planar Graphs Grinberg’s Theorem Recall (from Section 11.1) that Tait (1880) showed the Four-Colour Conjecture to be equivalent to the statement that every 3-connected cubic planar graph is 3-edge-colourable. Tait thought that he had thereby proved Four-Colour Con￾jecture, because he believed that every such graph was hamiltonian, and hence 3-edge-colourable. However, Tutte (1946) showed this to be false by constructing a nonhamiltonian 3-connected cubic planar graph (depicted in Figure 18.7) using ingenious ad hoc arguments (Exercise 18.2.1). For many years, the Tutte graph was