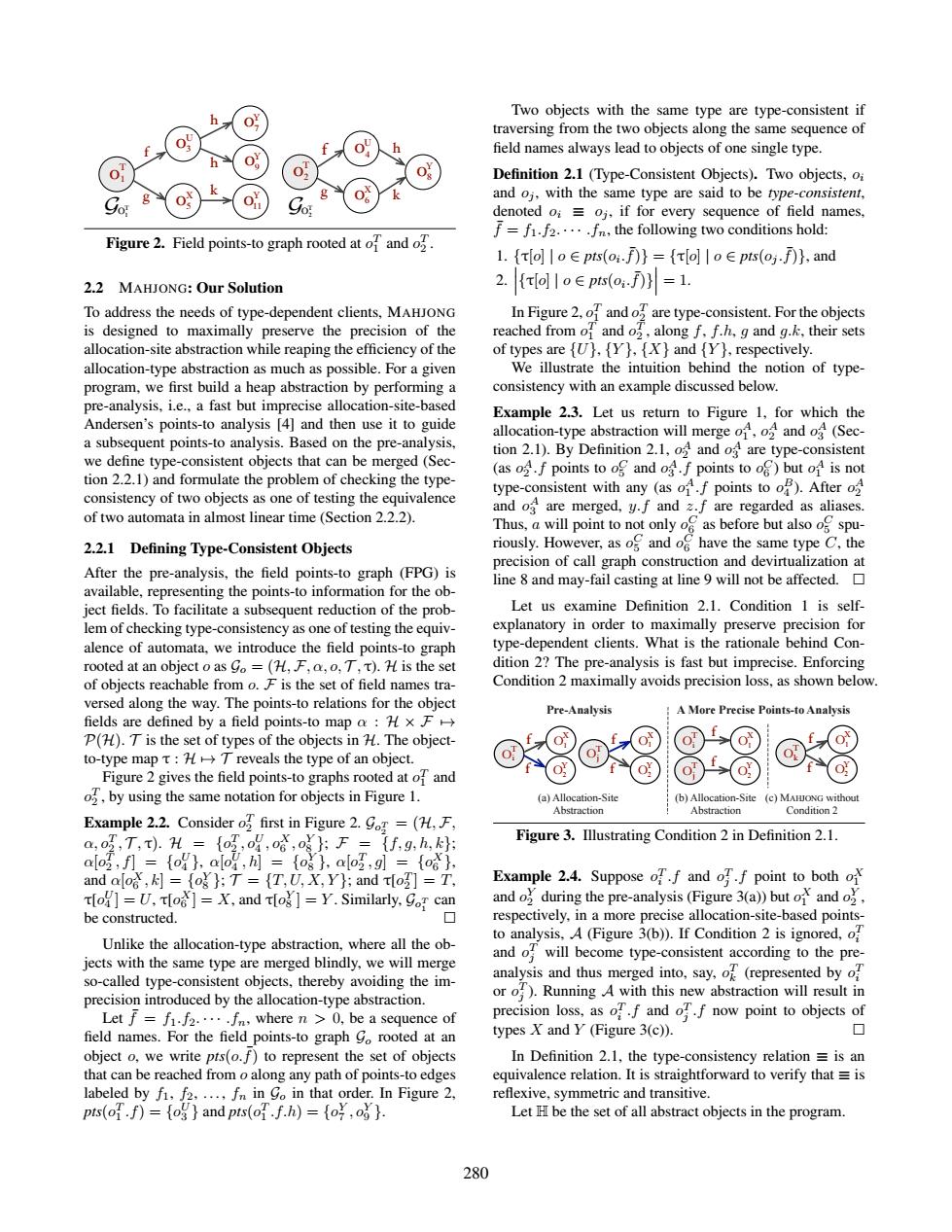
Two objects with the same type are type-consistent if 0 traversing from the two objects along the same sequence of field names always lead to objects of one single type. Definition 2.1(Type-Consistent Objects).Two objects,o and oj,with the same type are said to be type-consistent, denoted oi=oj,if for every sequence of field names. f =f1.f2.....fn,the following two conditions hold: Figure 2.Field points-to graph rooted at of and o. 1.{to|o∈pts(o.f)}={t[o|o∈pts(oj-f)},and 2.2 MAHJONG:Our Solution 2.{tlo]loE pts(oi.f))=1. To address the needs of type-dependent clients,MAHJONG In Figure 2,of and of are type-consistent.For the objects is designed to maximally preserve the precision of the reached from of and of,along f.f.h,g and g.k,their sets allocation-site abstraction while reaping the efficiency of the of types are (U),[){X}and (Y),respectively. allocation-type abstraction as much as possible.For a given We illustrate the intuition behind the notion of type- program,we first build a heap abstraction by performing a consistency with an example discussed below. pre-analysis,i.e.,a fast but imprecise allocation-site-based Example 2.3.Let us return to Figure 1,for which the Andersen's points-to analysis [4]and then use it to guide a subsequent points-to analysis.Based on the pre-analysis, allocation-type abstraction will merge of,o2 and o(Sec- tion 2.1).By Definition 2.1,o and o are type-consistent we define type-consistent objects that can be merged(Sec- tion 2.2.1)and formulate the problem of checking the type- (as os.f points to of and og.f points to o)but of is not type-consistent with any (as of.f points to of).After o2 consistency of two objects as one of testing the equivalence of two automata in almost linear time(Section 2.2.2). and o are merged,y.f and z.f are regarded as aliases. Thus,a will point to not only o as before but also of spu- 2.2.1 Defining Type-Consistent Objects riously.However,as os and o have the same type C,the precision of call graph construction and devirtualization at After the pre-analysis,the field points-to graph(FPG)is line 8 and may-fail casting at line 9 will not be affected. available,representing the points-to information for the ob- ject fields.To facilitate a subsequent reduction of the prob- Let us examine Definition 2.1.Condition 1 is self- lem of checking type-consistency as one of testing the equiv- explanatory in order to maximally preserve precision for alence of automata,we introduce the field points-to graph type-dependent clients.What is the rationale behind Con- rooted at an object o as go =(H,F,a,o,T,T).H is the set dition 2?The pre-analysis is fast but imprecise.Enforcing of objects reachable from o.F is the set of field names tra Condition 2 maximally avoids precision loss,as shown below. versed along the way.The points-to relations for the object Pre-Analysis A More Precise Points-to Analysis fields are defined by a field points-to map a HxF P(H).T is the set of types of the objects in H.The object- to-type map t:HT reveals the type of an object. Figure 2 gives the field points-to graphs rooted at of and o,by using the same notation for objects in Figure 1. (a)Allocation-Site (b)Allocation-Site (c)MAHONG without Abstraction Abstraction Condition 2 Example 2.2.Consider of first in Figure 2.gor =(H,F, a,o,T,).H={,,哈，o；F={f,g,h,k Figure 3.Illustrating Condition 2 in Definition 2.1. ao,fl={oMao,h={og,ao,g={哈1， and alo哈，={ogT={T,U,X,Y};and tlo1?]=T, Example 2.4.Suppose of.f and of.f point to bothox rou]=U.tlo ]=X,and t[os ]=Y.Similarly,gor can and o during the pre-analysis(Figure 3(a))but of and o3, be constructed. respectively,in a more precise allocation-site-based points- Unlike the allocation-type abstraction,where all the ob- to analysis,A(Figure 3(b)).If Condition 2 is ignored,o and of will become type-consistent according to the pre- jects with the same type are merged blindly,we will merge so-called type-consistent objects,thereby avoiding the im- analysis and thus merged into,say,of(represented by of precision introduced by the allocation-type abstraction. or of).Running A with this new abstraction will result in Let f =f1.f2.....fn,where n 0,be a sequence of precision loss,as o.f and of.f now point to objects of field names.For the field points-to graph go rooted at an types X and Y(Figure 3(c)). 口 object o,we write pts(o.f)to represent the set of objects In Definition 2.1,the type-consistency relation is an that can be reached from o along any path of points-to edges equivalence relation.It is straightforward to verify that =is labeled by fi.f2.....fn in o in that order.In Figure 2. reflexive,symmetric and transitive. pts(of.f)=tog}and pts(of.f.h)=to,o. Let H be the set of all abstract objects in the program. 2803 U 5 X 9 Y 11 g Y k 1 T o 7 Y h h f o o o o o 2 T 4 U 6 X 8 Y g f h k o o o o 1 T o 2 T G Go Figure 2. Field points-to graph rooted at o T 1 and o T 2 . 2.2 MAHJONG: Our Solution To address the needs of type-dependent clients, MAHJONG is designed to maximally preserve the precision of the allocation-site abstraction while reaping the efficiency of the allocation-type abstraction as much as possible. For a given program, we first build a heap abstraction by performing a pre-analysis, i.e., a fast but imprecise allocation-site-based Andersen’s points-to analysis [4] and then use it to guide a subsequent points-to analysis. Based on the pre-analysis, we define type-consistent objects that can be merged (Sec￾tion 2.2.1) and formulate the problem of checking the type￾consistency of two objects as one of testing the equivalence of two automata in almost linear time (Section 2.2.2). 2.2.1 Defining Type-Consistent Objects After the pre-analysis, the field points-to graph (FPG) is available, representing the points-to information for the ob￾ject fields. To facilitate a subsequent reduction of the prob￾lem of checking type-consistency as one of testing the equiv￾alence of automata, we introduce the field points-to graph rooted at an object o as Go = (H, F, α, o, T , τ). H is the set of objects reachable from o. F is the set of field names tra￾versed along the way. The points-to relations for the object fields are defined by a field points-to map α : H × F 7→ P(H). T is the set of types of the objects in H. The object￾to-type map τ : H 7→ T reveals the type of an object. Figure 2 gives the field points-to graphs rooted at o T 1 and o T 2 , by using the same notation for objects in Figure 1. Example 2.2. Consider o T 2 first in Figure 2. Go T 2 = (H, F, α, oT 2 , T , τ). H = {o T 2 , oU 4 , oX 6 , oY 8 }; F = {f, g, h, k}; α[o T 2 , f] = {o U 4 }, α[o U 4 , h] = {o Y 8 }, α[o T 2 , g] = {o X 6 }, and α[o X 6 , k] = {o Y 8 }; T = {T, U, X, Y }; and τ[o T 2 ] = T, τ[o U 4 ] = U, τ[o X 6 ] = X, and τ[o Y 8 ] = Y . Similarly, Go T 1 can be constructed. Unlike the allocation-type abstraction, where all the ob￾jects with the same type are merged blindly, we will merge so-called type-consistent objects, thereby avoiding the im￾precision introduced by the allocation-type abstraction. Let ¯f = f1.f2. · · · .fn, where n > 0, be a sequence of field names. For the field points-to graph Go rooted at an object o, we write pts(o. ¯f) to represent the set of objects that can be reached from o along any path of points-to edges labeled by f1, f2, . . . , fn in Go in that order. In Figure 2, pts(o T 1 .f) = {o U 3 } and pts(o T 1 .f.h) = {o Y 7 , oY 9 }. Two objects with the same type are type-consistent if traversing from the two objects along the same sequence of field names always lead to objects of one single type. Definition 2.1 (Type-Consistent Objects). Two objects, oi and oj , with the same type are said to be type-consistent, denoted oi ≡ oj , if for every sequence of field names, ¯f = f1.f2. · · · .fn, the following two conditions hold: 1. {τ[o] | o ∈ pts(oi . ¯f)} = {τ[o] | o ∈ pts(oj . ¯f)}, and 2.   {τ[o] | o ∈ pts(oi . ¯f)}    = 1. In Figure 2, o T 1 and o T 2 are type-consistent. For the objects reached from o T 1 and o T 2 , along f, f.h, g and g.k, their sets of types are {U}, {Y }, {X} and {Y }, respectively. We illustrate the intuition behind the notion of type￾consistency with an example discussed below. Example 2.3. Let us return to Figure 1, for which the allocation-type abstraction will merge o A 1 , o A 2 and o A 3 (Sec￾tion 2.1). By Definition 2.1, o A 2 and o A 3 are type-consistent (as o A 2 .f points to o C 5 and o A 3 .f points to o C 6 ) but o A 1 is not type-consistent with any (as o A 1 .f points to o B 4 ). After o A 2 and o A 3 are merged, y.f and z.f are regarded as aliases. Thus, a will point to not only o C 6 as before but also o C 5 spu￾riously. However, as o C 5 and o C 6 have the same type C, the precision of call graph construction and devirtualization at line 8 and may-fail casting at line 9 will not be affected. Let us examine Definition 2.1. Condition 1 is self￾explanatory in order to maximally preserve precision for type-dependent clients. What is the rationale behind Con￾dition 2? The pre-analysis is fast but imprecise. Enforcing Condition 2 maximally avoids precision loss, as shown below. f O T i f f Pre-Analysis A More Precise Points-to Analysis (a) Allocation-Site Abstraction (b) Allocation-Site Abstraction (c) MAHJONG without Condition 2 O X 1 O Y 2 O T i O X 1 f O T j O Y 2 O T j f f O X 1 O Y 2 O T k f f O X 1 O Y 2 Figure 3. Illustrating Condition 2 in Definition 2.1. Example 2.4. Suppose o T i .f and o T j .f point to both o X 1 and o Y 2 during the pre-analysis (Figure 3(a)) but o X 1 and o Y 2 , respectively, in a more precise allocation-site-based points￾to analysis, A (Figure 3(b)). If Condition 2 is ignored, o T i and o T j will become type-consistent according to the pre￾analysis and thus merged into, say, o T k (represented by o T i or o T j ). Running A with this new abstraction will result in precision loss, as o T i .f and o T j .f now point to objects of types X and Y (Figure 3(c)). In Definition 2.1, the type-consistency relation ≡ is an equivalence relation. It is straightforward to verify that ≡ is reflexive, symmetric and transitive. Let H be the set of all abstract objects in the program. 280