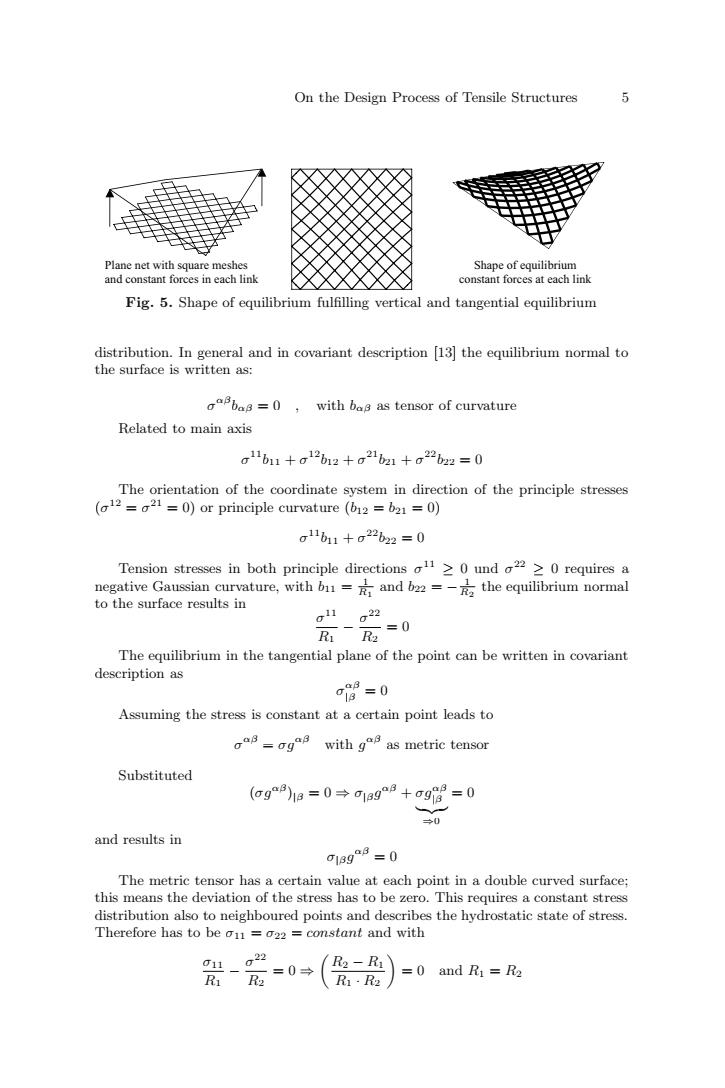
On the Design Process of Tensile Structures 5 Plane net with square meshes Shape of equilibrium and constant forces in each link constant forces at each link Fig.5.Shape of equilibrium fulfilling vertical and tangential equilibrium distribution.In general and in covariant description [13]the equilibrium normal to the surface is written as: =0,with baa as tensor of curvature Related to main axis o11b11+g12b12+o21b21+o22b22=0 The orientation of the coordinate system in direction of the principle stresses (o12=o21=0)or principle curvature (612 =621 =0) 011b11+o22b22=0 Tension stresses in both principle directions o11 >0 und o22 >0 requires a negative Gaussian curvature,with buand bthe equilibrium normal 1 to the surface results in o122 =0 R1 R2 The equilibrium in the tangential plane of the point can be written in covariant description as 8=0 Assuming the stress is constant at a certain point leads to o=agas with gas as metric tensor Substituted (cg8)1e=0→01B98+c9g2=0 ÷0 and results in 01398=0 The metric tensor has a certain value at each point in a double curved surface; this means the deviation of the stress has to be zero.This requires a constant stress distribution also to neighboured points and describes the hydrostatic state of stress. Therefore has to be o11 =022=constant and with R2-B1 =0 and R1 =R2 R1·R2On the Design Process of Tensile Structures 5 Fig. 5. Shape of equilibrium fulfilling vertical and tangential equilibrium distribution. In general and in covariant description [13] the equilibrium normal to the surface is written as: σαβbαβ = 0 , with bαβ as tensor of curvature Related to main axis σ11 b11 + σ12 b12 + σ21b21 + σ22 b22 = 0 The orientation of the coordinate system in direction of the principle stresses (σ12 = σ21 = 0) or principle curvature (b12 = b21 = 0) σ11b11 + σ22b22 = 0 Tension stresses in both principle directions σ11 ≥ 0 und σ22 ≥ 0 requires a negative Gaussian curvature, with b11 = 1 R1 and b22 = − 1 R2 the equilibrium normal to the surface results in σ11 R1 − σ22 R2 = 0 The equilibrium in the tangential plane of the point can be written in covariant description as σαβ |β = 0 Assuming the stress is constant at a certain point leads to σαβ = σgαβ with gαβ as metric tensor Substituted (σgαβ)|β = 0 ⇒ σ|βgαβ + σgαβ |β
 ⇒0 = 0 and results in σ|βgαβ = 0 The metric tensor has a certain value at each point in a double curved surface; this means the deviation of the stress has to be zero. This requires a constant stress distribution also to neighboured points and describes the hydrostatic state of stress. Therefore has to be σ11 = σ22 = constant and with σ11 R1 − σ22 R2 = 0 ⇒ R2 − R1 R1 · R2  = 0 and R1 = R2 Plane net with square meshes and constant forces in each link Shape of equilibrium constant forces at each link