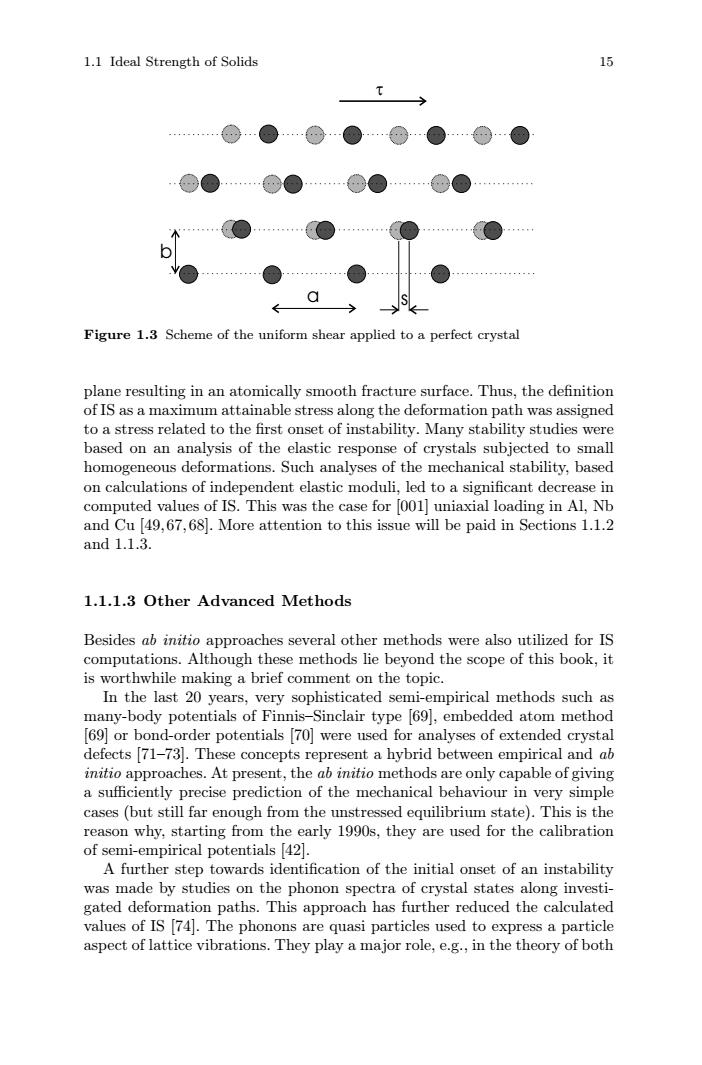
1.1 Ideal Strength of Solids 15 Figure 1.3 Scheme of the uniform shear applied to a perfect crystal plane resulting in an atomically smooth fracture surface.Thus,the definition of IS as a maximum attainable stress along the deformation path was assigned to a stress related to the first onset of instability.Many stability studies were based on an analysis of the elastic response of crystals subjected to small homogeneous deformations.Such analyses of the mechanical stability,based on calculations of independent elastic moduli,led to a significant decrease in computed values of IS.This was the case for 001 uniaxial loading in Al,Nb and Cu [49,67,68.More attention to this issue will be paid in Sections 1.1.2 and1.1.3. 1.1.1.3 Other Advanced Methods Besides ab initio approaches several other methods were also utilized for IS computations.Although these methods lie beyond the scope of this book,it is worthwhile making a brief comment on the topic. In the last 20 years,very sophisticated semi-empirical methods such as many-body potentials of Finnis-Sinclair type 69],embedded atom method 69]or bond-order potentials [70]were used for analyses of extended crystal defects [71-73].These concepts represent a hybrid between empirical and ab initio approaches.At present,the ab initio methods are only capable of giving a sufficiently precise prediction of the mechanical behaviour in very simple cases (but still far enough from the unstressed equilibrium state).This is the reason why,starting from the early 1990s,they are used for the calibration of semi-empirical potentials [42]. A further step towards identification of the initial onset of an instability was made by studies on the phonon spectra of crystal states along investi- gated deformation paths.This approach has further reduced the calculated values of IS [74].The phonons are quasi particles used to express a particle aspect of lattice vibrations.They play a major role,e.g.,in the theory of both1.1 Ideal Strength of Solids 15 a s b Figure 1.3 Scheme of the uniform shear applied to a perfect crystal plane resulting in an atomically smooth fracture surface. Thus, the definition of IS as a maximum attainable stress along the deformation path was assigned to a stress related to the first onset of instability. Many stability studies were based on an analysis of the elastic response of crystals subjected to small homogeneous deformations. Such analyses of the mechanical stability, based on calculations of independent elastic moduli, led to a significant decrease in computed values of IS. This was the case for [001] uniaxial loading in Al, Nb and Cu [49, 67, 68]. More attention to this issue will be paid in Sections 1.1.2 and 1.1.3. 1.1.1.3 Other Advanced Methods Besides ab initio approaches several other methods were also utilized for IS computations. Although these methods lie beyond the scope of this book, it is worthwhile making a brief comment on the topic. In the last 20 years, very sophisticated semi-empirical methods such as many-body potentials of Finnis–Sinclair type [69], embedded atom method [69] or bond-order potentials [70] were used for analyses of extended crystal defects [71–73]. These concepts represent a hybrid between empirical and ab initio approaches. At present, the ab initio methods are only capable of giving a sufficiently precise prediction of the mechanical behaviour in very simple cases (but still far enough from the unstressed equilibrium state). This is the reason why, starting from the early 1990s, they are used for the calibration of semi-empirical potentials [42]. A further step towards identification of the initial onset of an instability was made by studies on the phonon spectra of crystal states along investi￾gated deformation paths. This approach has further reduced the calculated values of IS [74]. The phonons are quasi particles used to express a particle aspect of lattice vibrations. They play a major role, e.g., in the theory of both