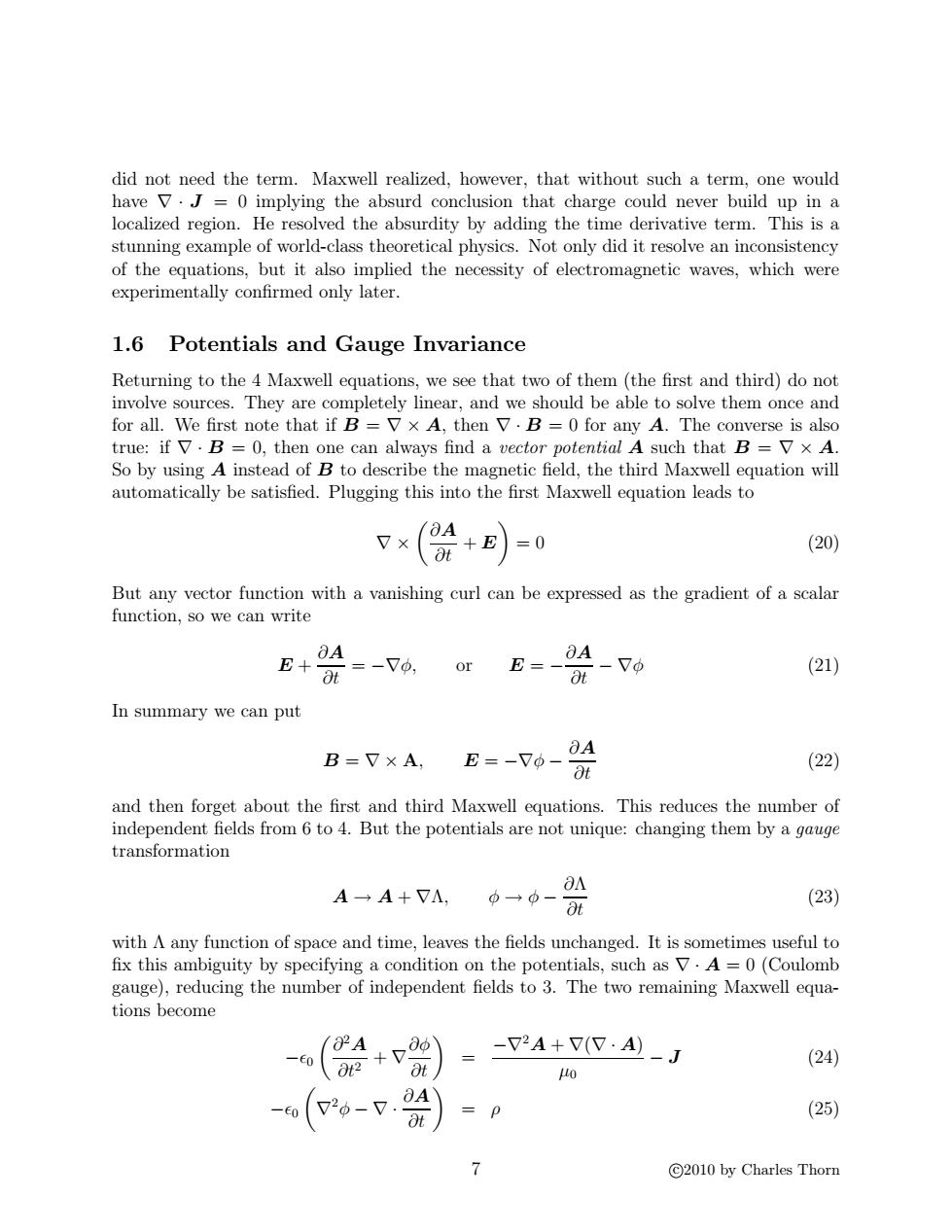
did not need the term.Maxwell realized,however,that without such a term,one would have V.J=0 implying the absurd conclusion that charge could never build up in a localized region.He resolved the absurdity by adding the time derivative term.This is a stunning example of world-class theoretical physics.Not only did it resolve an inconsistency of the equations,but it also implied the necessity of electromagnetic waves,which were experimentally confirmed only later. 1.6 Potentials and Gauge Invariance Returning to the 4 Maxwell equations,we see that two of them (the first and third)do not involve sources.They are completely linear,and we should be able to solve them once and for all.We first note that if B=Vx A,then V.B =0 for any A.The converse is also true:if V.B =0,then one can always find a vector potential A such that B=V x A. So by using A instead of B to describe the magnetic field,the third Maxwell equation will automatically be satisfied.Plugging this into the first Maxwell equation leads to (+ =0 (20) But any vector function with a vanishing curl can be expressed as the gradient of a scalar function,so we can write ∂A E+ =-7φ， or E-- Ot aA-Vφ 8t (21) In summary we can put E=-V6- 6A B=V×A, Ot (22) and then forget about the first and third Maxwell equations.This reduces the number of independent fields from 6 to 4.But the potentials are not unique:changing them by a gauge transformation a A→A+7Λ， 0→φ- (23) with A any function of space and time,leaves the fields unchanged.It is sometimes useful to fix this ambiguity by specifying a condition on the potentials,such as V.A=0(Coulomb gauge),reducing the number of independent fields to 3.The two remaining Maxwell equa- tions become 02A 一E0 +V -V2A+(.A-J (24) 8t 0 720-7 (25) ©2010 by Charles Thorndid not need the term. Maxwell realized, however, that without such a term, one would have ∇ · J = 0 implying the absurd conclusion that charge could never build up in a localized region. He resolved the absurdity by adding the time derivative term. This is a stunning example of world-class theoretical physics. Not only did it resolve an inconsistency of the equations, but it also implied the necessity of electromagnetic waves, which were experimentally confirmed only later. 1.6 Potentials and Gauge Invariance Returning to the 4 Maxwell equations, we see that two of them (the first and third) do not involve sources. They are completely linear, and we should be able to solve them once and for all. We first note that if B = ∇ × A, then ∇ · B = 0 for any A. The converse is also true: if ∇ · B = 0, then one can always find a vector potential A such that B = ∇ × A. So by using A instead of B to describe the magnetic field, the third Maxwell equation will automatically be satisfied. Plugging this into the first Maxwell equation leads to ∇ ×  ∂A ∂t + E  = 0 (20) But any vector function with a vanishing curl can be expressed as the gradient of a scalar function, so we can write E + ∂A ∂t = −∇φ, or E = − ∂A ∂t − ∇φ (21) In summary we can put B = ∇ × A, E = −∇φ − ∂A ∂t (22) and then forget about the first and third Maxwell equations. This reduces the number of independent fields from 6 to 4. But the potentials are not unique: changing them by a gauge transformation A → A + ∇Λ, φ → φ − ∂Λ ∂t (23) with Λ any function of space and time, leaves the fields unchanged. It is sometimes useful to fix this ambiguity by specifying a condition on the potentials, such as ∇ · A = 0 (Coulomb gauge), reducing the number of independent fields to 3. The two remaining Maxwell equa￾tions become −0  ∂ 2A ∂t 2 + ∇ ∂φ ∂t  = −∇2A + ∇(∇ · A) µ0 − J (24) −0  ∇ 2φ − ∇ · ∂A ∂t  = ρ (25) 7 c 2010 by Charles Thorn