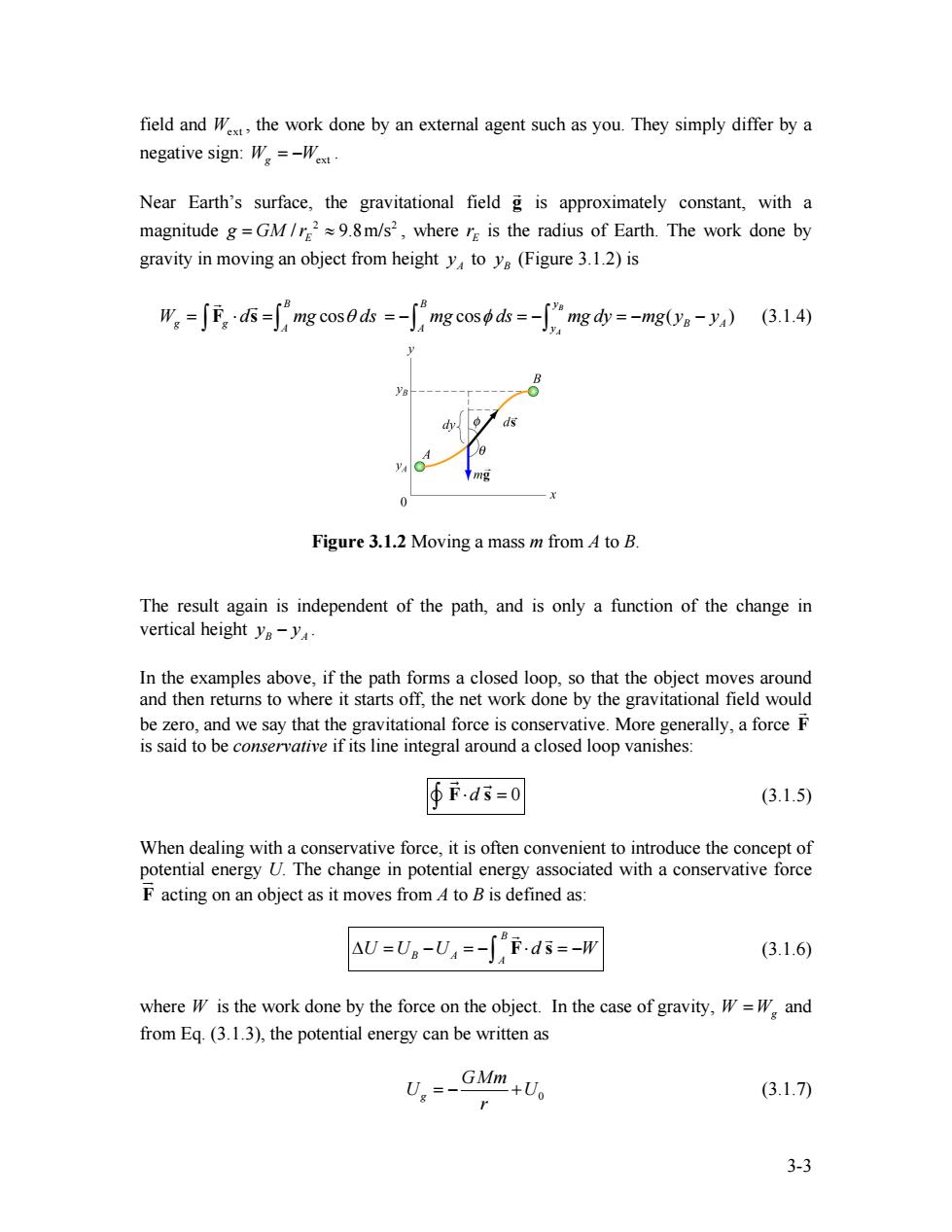
field and W,the work done by an external agent such as you.They simply differ by a negative sign:W=-West Near Earth's surface,the gravitational field g is approximately constant,with a magnitude g=GM/29.8m/s2,where re is the radius of Earth.The work done by gravity in moving an object from height y to y(Figure 3.1.2)is W=∫厘ds=-∫mg cos6s=-∫mg cods=-∫mg少=-mg0a-y4)(6.14) B dy Figure 3.1.2 Moving a mass m from A to B. The result again is independent of the path,and is only a function of the change in vertical height ye-y In the examples above,if the path forms a closed loop,so that the object moves around and then returns to where it starts off,the net work done by the gravitational field would be zero,and we say that the gravitational force is conservative.More generally,a force F is said to be conservative if its line integral around a closed loop vanishes: ∮F.ds=0 (3.1.5) When dealing with a conservative force,it is often convenient to introduce the concept of potential energy U.The change in potential energy associated with a conservative force F acting on an object as it moves from A to B is defined as: AU=U。-U4=-∫F.ds=-W (3.1.6) where W is the work done by the force on the object.In the case of gravity,W=W and from Eq.(3.1.3),the potential energy can be written as U,=-GMm+U。 (3.1.7) 3-3field and , the work done by an external agent such as you. They simply differ by a negative sign: . Wext Wg = −Wext Near Earth’s surface, the gravitational field g G is approximately constant, with a magnitude , where is the radius of Earth. The work done by gravity in moving an object from height 2 2 / 9.8m/ E g G= M r ≈ s Er A y to (Figure 3.1.2) is B y cos cos ( ) B A B B y g g B A A A y W = ⋅ d = mg θ φ ds = − mg ds = − mg dy = −mg y − y ∫ ∫ ∫ ∫ F s G G (3.1.4) Figure 3.1.2 Moving a mass m from A to B. The result again is independent of the path, and is only a function of the change in vertical height . B A y y − In the examples above, if the path forms a closed loop, so that the object moves around and then returns to where it starts off, the net work done by the gravitational field would be zero, and we say that the gravitational force is conservative. More generally, a force F G is said to be conservative if its line integral around a closed loop vanishes: ⋅ d = 0 ∫ F s G G v (3.1.5) When dealing with a conservative force, it is often convenient to introduce the concept of potential energy U. The change in potential energy associated with a conservative force F acting on an object as it moves from A to B is defined as: JG B B A A ∆ = U U −U = −∫ F s ⋅ d = −W G G (3.1.6) where W is the work done by the force on the object. In the case of gravity, W = Wg and from Eq. (3.1.3), the potential energy can be written as g 0 GMm U r = − +U (3.1.7) 3-3