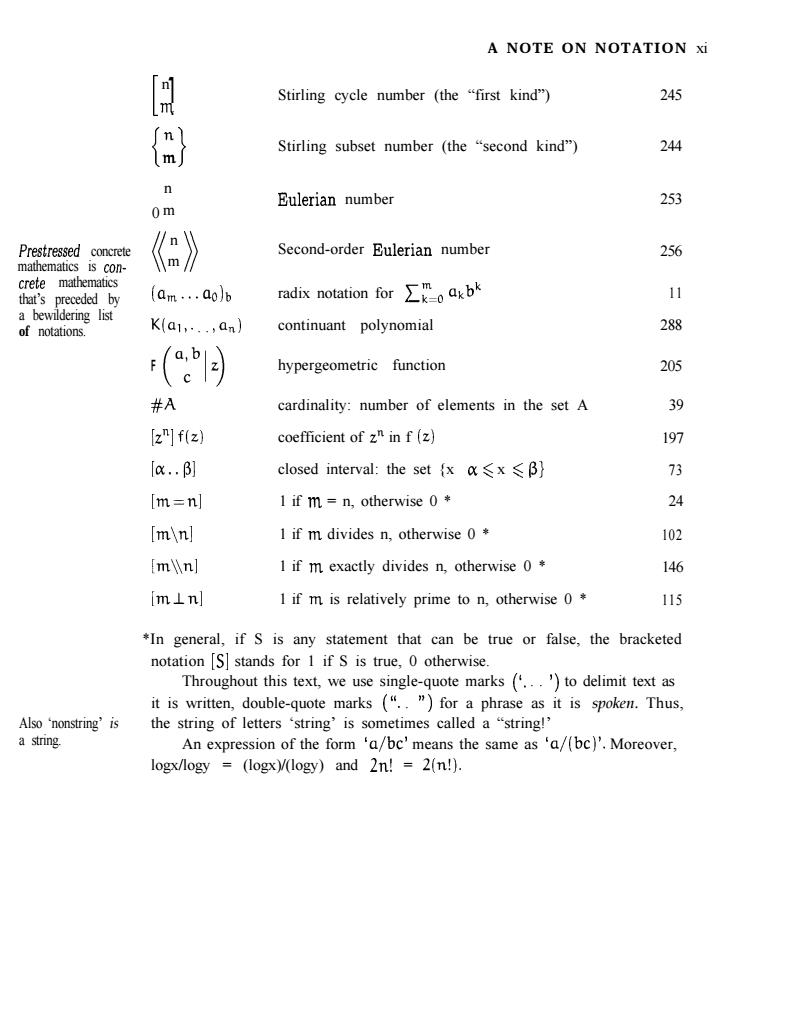
A NOTE ON NOTATION xi R Stirling cycle number (the "first kind") 245 Stirling subset number (the "second kind") 244 n Eulerian number 253 0m n Prestressed concrete Second-order Eulerian number 256 mathematics is con- m/∥ crete mathematics that's preceded by (am...ao)b radix notation for ∑oakb水 11 a bewildering list of notations. K(a1,.,an) continuant polynomial 288 (1习 hypergeometric function 205 #A cardinality:number of elements in the set A 39 [z"]f(z) coefficient of z in f (z) 197 [c.] closed interval:the set{x≤x≤y 73 [m=n] 1 if m n,otherwise 0* 24 [m\n] 1 if m divides n,otherwise 0* 102 [m\n] 1 if m exactly divides n,otherwise 0* 146 m⊥n 1 if m is relatively prime to n,otherwise 0* 115 *In general,if S is any statement that can be true or false,the bracketed notation [S]stands for 1 if S is true,0 otherwise. Throughout this text,we use single-quote marks(...')to delimit text as it is written,double-quote marks ("..")for a phrase as it is spoken.Thus, Also‘nonstring'is the string of letters 'string'is sometimes called a "string!' a string An expression of the form 'a/bc'means the same as 'a/(bc)'.Moreover, logx/logy (logx)/(logy)and 2n!=2(n!).n [ 1n-l n {Im n 0 m n Prestressed concrete mathematics is con- (i m >> Crete mathematics that’s preceded by (‘h...%)b a bewildering list of notations. K(al,. . . ,a,) F #A iz”l f(z) la..@1 [m=nl [m\nl Im\nl [m-l-n1 A NOTE ON NOTATION xi Stirling cycle number (the “first kind”) 245 Stirling subset number (the “second kind”) 244 Eulerian number 253 Second-order Eulerian number 256 radix notation for z,“=, akbk 11 continuant polynomial 288 hypergeometric function 205 cardinality: number of elements in the set A 39 coefficient of zn in f (2) 197 closed interval: the set {x 1016 x 6 (3} 73 1 if m = n, otherwise 0 * 24 1 if m divides n, otherwise 0 * 102 1 if m exactly divides n, otherwise 0 * 146 1 if m is relatively prime to n, otherwise 0 * 115 *In general, if S is any statement that can be true or false, the bracketed notation [S] stands for 1 if S is true, 0 otherwise. Throughout this text, we use single-quote marks (‘. . . ‘) to delimit text as it is written, double-quote marks (“. . “ ) for a phrase as it is spoken. Thus, Also ‘nonstring’ is the string of letters ‘string’ is sometimes called a “string!’ a string. An expression of the form ‘a/be’ means the same as ‘a/(bc)‘. Moreover, logx/logy = (logx)/(logy) and 2n! = 2(n!)