正在加载图片...
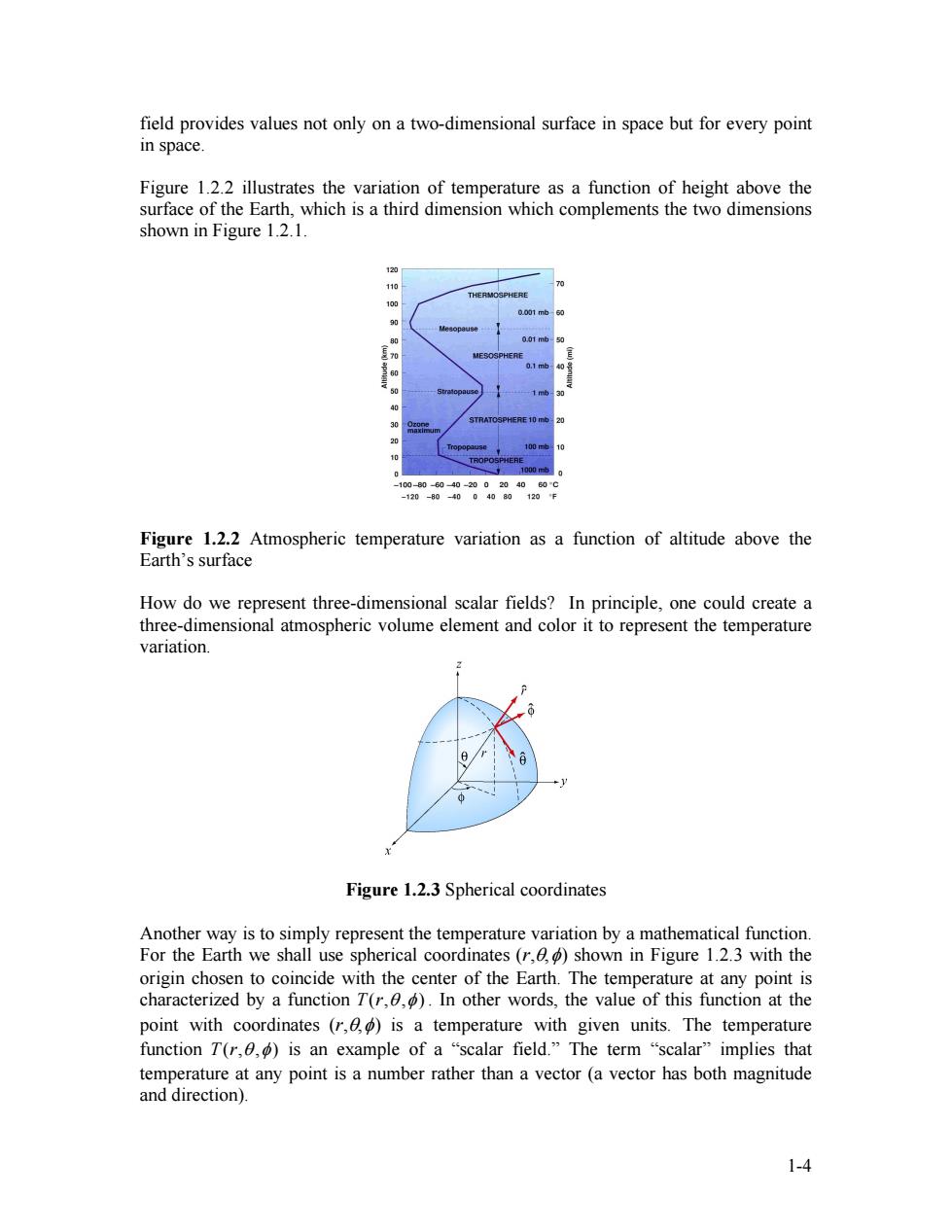
field provides values not only on a two-dimensional surface in space but for every point in space. Figure 1.2.2 illustrates the variation of temperature as a function of height above the surface of the Earth,which is a third dimension which complements the two dimensions shown in Figure 1.2.1. 0001m 40 30 20 100 10 10000 -1008060402002040600 -120 -80-40 04080 120F Figure 1.2.2 Atmospheric temperature variation as a function of altitude above the Earth's surface How do we represent three-dimensional scalar fields?In principle,one could create a three-dimensional atmospheric volume element and color it to represent the temperature variation. Figure 1.2.3 Spherical coordinates Another way is to simply represent the temperature variation by a mathematical function. For the Earth we shall use spherical coordinates (r,e)shown in Figure 1.2.3 with the origin chosen to coincide with the center of the Earth.The temperature at any point is characterized by a function T(r,,)In other words,the value of this function at the point with coordinates (r,e)is a temperature with given units.The temperature function T(r,g,p)is an example of a“scalar field.”The term“scalar'”implies that temperature at any point is a number rather than a vector(a vector has both magnitude and direction). 1-41-4 field provides values not only on a two-dimensional surface in space but for every point in space. Figure 1.2.2 illustrates the variation of temperature as a function of height above the surface of the Earth, which is a third dimension which complements the two dimensions shown in Figure 1.2.1. Figure 1.2.2 Atmospheric temperature variation as a function of altitude above the Earth’s surface How do we represent three-dimensional scalar fields? In principle, one could create a three-dimensional atmospheric volume element and color it to represent the temperature variation. Figure 1.2.3 Spherical coordinates Another way is to simply represent the temperature variation by a mathematical function. For the Earth we shall use spherical coordinates (r,θ,φ) shown in Figure 1.2.3 with the origin chosen to coincide with the center of the Earth. The temperature at any point is characterized by a function T (r,θ ,φ) . In other words, the value of this function at the point with coordinates (r,θ,φ) is a temperature with given units. The temperature function T r(, , ) θ φ is an example of a “scalar field.” The term “scalar” implies that temperature at any point is a number rather than a vector (a vector has both magnitude and direction)