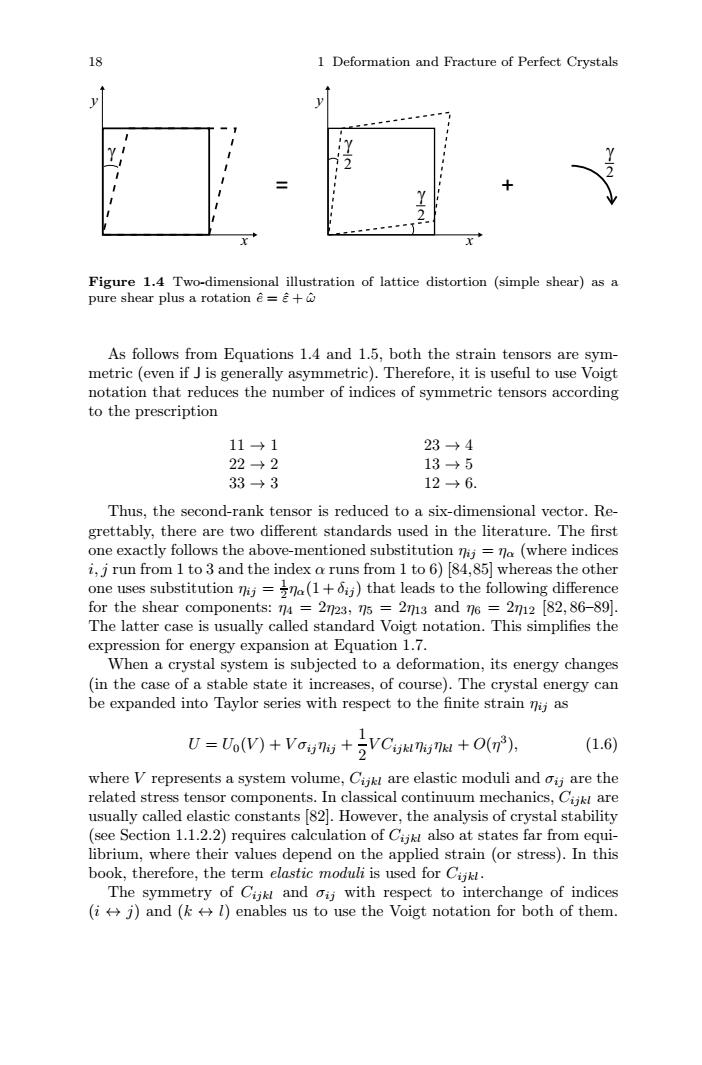
18 1 Deformation and Fracture of Perfect Crystals 十 Figure 1.4 Two-dimensional illustration of lattice distortion (simple shear)as a pure shear plus a rotation = As follows from Equations 1.4 and 1.5,both the strain tensors are sym- metric(even if J is generally asymmetric).Therefore,it is useful to use Voigt notation that reduces the number of indices of symmetric tensors according to the prescription 11→1 23→4 22→2 13→5 33→3 12→6. Thus,the second-rank tensor is reduced to a six-dimensional vector.Re- grettably,there are two different standards used in the literature.The first one exactly follows the above-mentioned substitution nij=na (where indices i,j run from 1 to 3 and the index a runs from 1 to 6)84,85]whereas the other one uses substitution nij =gn(1+ij)that leads to the following difference for the shear components:n4 2n23,n5 2m3 and n6 2n2 [82,86-89. The latter case is usually called standard Voigt notation.This simplifies the expression for energy expansion at Equation 1.7. When a crystal system is subjected to a deformation,its energy changes (in the case of a stable state it increases,of course).The crystal energy can be expanded into Taylor series with respect to the finite strain nij as U=Uo(V)+Vo+VCms+(n ) (1.6) where V represents a system volume,Cijki are elastic moduli and oij are the related stress tensor components.In classical continuum mechanics,Ciikt are usually called elastic constants [82.However,the analysis of crystal stability (see Section 1.1.2.2)requires calculation of Ciikl also at states far from equi- librium,where their values depend on the applied strain (or stress).In this book,therefore,the term elastic moduli is used for Cijki. The symmetry of Cijkt and oij with respect to interchange of indices (ij)and (enables us to use the Voigt notation for both of them.18 1 Deformation and Fracture of Perfect Crystals y x y x = + 2  2  2   Figure 1.4 Two-dimensional illustration of lattice distortion (simple shear) as a pure shear plus a rotation ˆe = ˆε + ˆω As follows from Equations 1.4 and 1.5, both the strain tensors are sym￾metric (even if J is generally asymmetric). Therefore, it is useful to use Voigt notation that reduces the number of indices of symmetric tensors according to the prescription 11 → 1 23 → 4 22 → 2 13 → 5 33 → 3 12 → 6. Thus, the second-rank tensor is reduced to a six-dimensional vector. Re￾grettably, there are two different standards used in the literature. The first one exactly follows the above-mentioned substitution ηij = ηα (where indices i, j run from 1 to 3 and the index α runs from 1 to 6) [84,85] whereas the other one uses substitution ηij = 1 2 ηα(1 + δij ) that leads to the following difference for the shear components: η4 = 2η23, η5 = 2η13 and η6 = 2η12 [82, 86–89]. The latter case is usually called standard Voigt notation. This simplifies the expression for energy expansion at Equation 1.7. When a crystal system is subjected to a deformation, its energy changes (in the case of a stable state it increases, of course). The crystal energy can be expanded into Taylor series with respect to the finite strain ηij as U = U0(V ) + V σijηij + 1 2 V Cijklηijηkl + O(η3), (1.6) where V represents a system volume, Cijkl are elastic moduli and σij are the related stress tensor components. In classical continuum mechanics, Cijkl are usually called elastic constants [82]. However, the analysis of crystal stability (see Section 1.1.2.2) requires calculation of Cijkl also at states far from equi￾librium, where their values depend on the applied strain (or stress). In this book, therefore, the term elastic moduli is used for Cijkl. The symmetry of Cijkl and σij with respect to interchange of indices (i ↔ j) and (k ↔ l) enables us to use the Voigt notation for both of them